Hostname: page-component-76d6cb85b7-lcgwf Total loading time: 0 Render date: 2026-07-11T01:56:34.774Z Has data issue: false hasContentIssue false
  • English
  • Français

Large-scale convective flow sustained by thermally active Lagrangian tracers

Published online by Cambridge University Press:  02 December 2022

Lokahith Agasthya Open the ORCID record for Lokahith Agasthya [Opens in a new window] ,
Andreas Bartel ,
Luca Biferale Open the ORCID record for Luca Biferale [Opens in a new window] ,
Matthias Ehrhardt  and
Federico Toschi Open the ORCID record for Federico Toschi [Opens in a new window]
Lokahith Agasthya*
Affiliation:
Department of Physics, University of Rome ‘Tor Vergata’ and INFN, Via della Ricerca Scientifica 1, 00133 Rome RM, Italy Angewandte Mathematik und Numerische Analysis, Bergische Universität Wuppertal, Gaußstrasse 20, D-42119 Wuppertal, Germany Computation-based Science and Technology Research Center, The Cyprus Institute, 20 Kavafi Str., Nicosia 2121, Cyprus
Andreas Bartel
Affiliation:
Angewandte Mathematik und Numerische Analysis, Bergische Universität Wuppertal, Gaußstrasse 20, D-42119 Wuppertal, Germany
Luca Biferale
Affiliation:
Department of Physics, University of Rome ‘Tor Vergata’ and INFN, Via della Ricerca Scientifica 1, 00133 Rome RM, Italy
Matthias Ehrhardt
Affiliation:
Angewandte Mathematik und Numerische Analysis, Bergische Universität Wuppertal, Gaußstrasse 20, D-42119 Wuppertal, Germany
Federico Toschi
Affiliation:
Department of Applied Physics, Eindhoven University of Technology, PO Box 513, 5600 MB Eindhoven, The Netherlands
*
†Email address for correspondence: lnagasthya@gmail.com
Get access Rights & Permissions [Opens in a new window]

Abstract

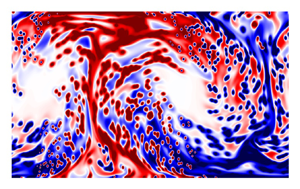
Non-isothermal particles suspended in a fluid lead to complex interactions – the particles respond to changes in the fluid flow, which in turn is modified by their temperature anomaly. Here, we perform a novel proof-of-concept numerical study based on tracer particles that are thermally coupled to the fluid. We imagine that particles can adjust their internal temperature reacting to some local fluid properties, and follow simple, hard-wired active control protocols. We study the case where instabilities are induced by switching the particle temperature from hot to cold depending on whether it is ascending or descending in the flow. A macroscopic transition from stable to unstable convective flow is achieved, depending on the number of active particles and their excess negative/positive temperature. The stable state is characterized by a flow with low turbulent kinetic energy, strongly stable temperature gradient, and no large-scale features. The convective state is characterized by higher turbulent kinetic energy, self-sustaining large-scale convection, and weakly stable temperature gradients. Individually, the particles promote the formation of stable temperature gradients, while their aggregated effect induces large-scale convection. When the Lagrangian temperature scale is small, a weakly convective laminar system forms. The Lagrangian approach is also compared to a uniform Eulerian bulk heating with the same mean injection profile, and no such transition is observed. Our empirical approach shows that thermal convection can be controlled by pure Lagrangian forcing, and opens the way for other data-driven particle-based protocols to enhance or deplete large-scale motion in thermal flows.

JFM classification

Convection: Buoyancy-driven instability Flow Control: Instability control

Information

Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 953 , 25 December 2022 , A5
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

REFERENCES

Ahlers, G., Grossmann, S. & Lohse, D. 2009 Heat transfer and large scale dynamics in turbulent Rayleigh–Bénard convection. Rev. Mod. Phys. 81 (2), 503.CrossRefGoogle Scholar
Bec, J., Homann, H. & Ray, S.S. 2014 Gravity-driven enhancement of heavy particle clustering in turbulent flow. Phys. Rev. Lett. 112 (18), 184501.CrossRefGoogle ScholarPubMed
Beintema, G., Corbetta, A., Biferale, L. & Toschi, F. 2020 Controlling Rayleigh–Bénard convection via reinforcement learning. J. Turbul. 21 (9–10), 585–605.CrossRefGoogle Scholar
Carbone, M., Bragg, A.D. & Iovieno, M. 2019 Multiscale fluid–particle thermal interaction in isotropic turbulence. J. Fluid Mech. 881, 679–721.CrossRefGoogle Scholar
Cencini, M., Bec, J., Biferale, L., Boffetta, G., Celani, A., Lanotte, A.S., Musacchio, S. & Toschi, F. 2006 Dynamics and statistics of heavy particles in turbulent flows. J. Turbul. 7, N36.CrossRefGoogle Scholar
Ching, E.S.C. 2014 Statistics and Scaling in Turbulent Rayleigh–Bénard Convection. Springer.CrossRefGoogle Scholar
Elperin, T., Kleeorin, N. & Rogachevskii, I. 1996 Turbulent thermal diffusion of small inertial particles. Phys. Rev. Lett. 76 (2), 224.CrossRefGoogle ScholarPubMed
Falkovich, G., Fouxon, A. & Stepanov, M.G. 2002 Acceleration of rain initiation by cloud turbulence. Nature 419 (6903), 151–154.CrossRefGoogle ScholarPubMed
Fernando, H.J.S., Zajic, D., Di Sabatino, S., Dimitrova, R., Hedquist, B. & Dallman, A. 2010 Flow, turbulence, and pollutant dispersion in urban atmospheres. Phys. Fluids 22 (5), 051301.CrossRefGoogle Scholar
Gayen, B., Griffiths, R.W. & Hughes, G.O. 2014 Stability transitions and turbulence in horizontal convection. J. Fluid Mech. 751, 698–724.CrossRefGoogle Scholar
Guo, Z., Zheng, C. & Shi, B. 2002 Discrete lattice effects on the forcing term in the lattice Boltzmann method. Phys. Rev. E 65, 046308.CrossRefGoogle ScholarPubMed
He, X., Chen, S. & Doolen, G.D. 1998 A novel thermal model for the lattice Boltzmann method in incompressible limit. J. Comput. Phys. 146 (1), 282–300.CrossRefGoogle Scholar
Irannejad, A., Banaeizadeh, A. & Jaberi, F. 2015 Large eddy simulation of turbulent spray combustion. Combust. Flame 162 (2), 431–450.CrossRefGoogle Scholar
Kim, H., et al. 2013 Liquid metal batteries: past, present, and future. Chem. Rev. 113 (3), 2075–2099.CrossRefGoogle ScholarPubMed
Ladd, A.J.C. 1994 Numerical simulations of particulate suspensions via a discretized Boltzmann equation. Part 1. Theoretical foundation. J. Fluid Mech. 271, 285–309.CrossRefGoogle Scholar
Lay, T., Hernlund, J. & Buffett, B.A. 2008 Core–mantle boundary heat flow. Nat. Geosci. 1 (1), 25–32.CrossRefGoogle Scholar
Limare, A., Vilella, K., Di Giuseppe, E., Farnetani, C.G., Kaminski, E., Surducan, E., Surducan, V., Neamtu, C., Fourel, L. & Jaupart, C. 2015 Microwave-heating laboratory experiments for planetary mantle convection. J. Fluid Mech. 777, 50–67.CrossRefGoogle Scholar
Lohse, D. & Xia, K.-Q. 2010 Small-scale properties of turbulent Rayleigh–Bénard convection. Annu. Rev. Fluid Mech. 42, 335–364.CrossRefGoogle Scholar
Markowski, P. 2007 An overview of atmospheric convection. In Atmospheric Convection: Research and Operational Forecasting Aspects, pp. 1–6. Springer.CrossRefGoogle Scholar
Marshall, J. & Schott, F. 1999 Open-ocean convection: observations, theory, and models. Rev. Geophys. 37 (1), 1–64.CrossRefGoogle Scholar
Mazin, I. 1999 The effect of condensation and evaporation on turbulence in clouds. Atmos. Res. 51 (2), 171–174.Google Scholar
Park, H.J., O'Keefe, K. & Richter, D.H. 2018 Rayleigh–Bénard turbulence modified by two-way coupled inertial, nonisothermal particles. Phys. Rev. Fluids 3 (3), 034307.CrossRefGoogle Scholar
Salesky, S.T. & Anderson, W. 2018 Buoyancy effects on large-scale motions in convective atmospheric boundary layers: implications for modulation of near-wall processes. J. Fluid Mech. 856, 135–168.CrossRefGoogle Scholar
Seta, T. 2013 Implicit temperature-correction-based immersed-boundary thermal lattice Boltzmann method for the simulation of natural convection. Phys. Rev. E 87, 063304.CrossRefGoogle ScholarPubMed
Siggia, E.D. 1994 High Rayleigh number convection. Annu. Rev. Fluid Mech. 26 (1), 137–168.CrossRefGoogle Scholar
Squires, K.D. & Eaton, J.K. 1991 Preferential concentration of particles by turbulence. Phys. Fluids A 3 (5), 1169–1178.CrossRefGoogle Scholar
Tang, J. & Bau, H.H. 1994 Stabilization of the no-motion state in the Rayleigh–Bénard problem. Proc. R. Soc. Lond. A 447 (1931), 587–607.Google Scholar
Tritton, D.J. 1975 Internally heated convection in the atmosphere of Venus and in the laboratory. Nature 257 (5522), 110–112.CrossRefGoogle Scholar
Wang, Q., Lohse, D. & Shishkina, O. 2021 Scaling in internally heated convection: a unifying theory. Geophys. Res. Lett. 48 (4), e2020GL091198.Google Scholar
Xi, H.-D., Zhang, Y.-B., Hao, J.-T. & Xia, K.-Q. 2016 Higher-order flow modes in turbulent Rayleigh–Bénard convection. J. Fluid Mech. 805, 31–51.CrossRefGoogle Scholar
Yang, T.S. & Shy, S.S. 2005 Two-way interaction between solid particles and homogeneous air turbulence: particle settling rate and turbulence modification measurements. J. Fluid Mech. 526, 171–216.CrossRefGoogle Scholar
Yoshino, M. & Inamuro, T. 2003 Lattice Boltzmann simulations for flow and heat/mass transfer problems in a three-dimensional porous structure. Intl J. Numer. Meth. Fluids 43 (2), 183–198.CrossRefGoogle Scholar
Zamansky, R., Coletti, F., Massot, M. & Mani, A. 2014 Radiation induces turbulence in particle-laden fluids. Phys. Fluids 26 (7), 071701.CrossRefGoogle Scholar
Zamansky, R., Coletti, F., Massot, M. & Mani, A. 2016 Turbulent thermal convection driven by heated inertial particles. J. Fluid Mech. 809, 390–437.CrossRefGoogle Scholar