Hostname: page-component-546b4f848f-bvkm5 Total loading time: 0 Render date: 2023-05-31T19:37:24.344Z Has data issue: false Feature Flags: { "useRatesEcommerce": true } hasContentIssue false
  • English

Low-dimensional dynamics of a turbulent axisymmetric wake

Published online by Cambridge University Press:  14 August 2014

G. Rigas ,
A. R. Oxlade ,
A. S. Morgans  and
J. F. Morrison
G. Rigas*
Affiliation:
Department of Aeronautics, Imperial College London, London SW7 2AZ, UK
A. R. Oxlade
Affiliation:
Department of Aeronautics, Imperial College London, London SW7 2AZ, UK
A. S. Morgans
Affiliation:
Department of Aeronautics, Imperial College London, London SW7 2AZ, UK
J. F. Morrison
Affiliation:
Department of Aeronautics, Imperial College London, London SW7 2AZ, UK
*
†Email address for correspondence: g.rigas@imperial.ac.uk
Get access Rights & Permissions[Opens in a new window]

Abstract

The coherent structures of a turbulent wake generated behind a bluff three-dimensional axisymmetric body are investigated experimentally at a diameter-based Reynolds number of $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}{\sim }2\times 10^5$. Proper orthogonal decomposition of base pressure measurements indicates that the most energetic coherent structures retain the structure of the symmetry-breaking laminar instabilities and are manifested as unsteady vortex shedding with azimuthal wavenumber 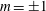$m={\pm }1$. In a rotating reference frame, the shedding preserves the reflectional symmetry and is linked with a reflectionally symmetric mean pressure distribution on the base. Due to a slow rotation of the symmetry plane of the turbulent wake around the axis of the body, statistical axisymmetry is recovered in the time average. The ratio of the time scales associated with the slow rotation of the symmetry plane and the vortex shedding is of order 100.

JFM classification

Turbulent Flows: Turbulent Flows Vortex Flows: Vortex shedding Wakes/Jets: Wakes/Jets
Type
Rapids
Information
Journal of Fluid Mechanics , Volume 755 , 25 September 2014 , R5
Copyright
© 2014 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Achenbach, E. 1974 Vortex shedding from spheres. J. Fluid Mech. 62, 209–221.CrossRefGoogle Scholar
Berger, E., Scholz, D. & Schumm, M. 1990 Coherent vortex structures in the wake of a sphere and a circular disk at rest and under forced vibrations. J. Fluids Struct. 4, 231–257.CrossRefGoogle Scholar
Bohorquez, P., Sanmiguel-Rojas, E., Sevilla, A., Jiménez-González, J. I. & Martínez-Bazán, C. 2011 Stability and dynamics of the laminar wake past a slender blunt-based axisymmetric body. J. Fluid Mech. 676, 110–144.CrossRefGoogle Scholar
Brown, E. & Ahlers, G. 2006 Rotations and cessations of the large-scale circulation in turbulent Rayleigh–Bénard convection. J. Fluid Mech. 568, 351–386.CrossRefGoogle Scholar
Bury, Y. & Jardin, T. 2012 Transitions to chaos in the wake of an axisymmetric bluff body. Phys. Lett. A 376, 3219–3222.CrossRefGoogle Scholar
Frisch, U. 1996 Turbulence. Cambridge University Press.Google ScholarPubMed
Fuchs, H. V., Mercker, E. & Michel, U. 1979 Large-scale coherent structures in the wake of axisymmetric bodies. J. Fluid Mech. 93, 185–207.CrossRefGoogle Scholar
Grandemange, M.2013 Analysis and control of three-dimensional turbulent wakes: from axisymmetric bodies to real road vehicles. PhD thesis, ENSTA ParisTech.Google Scholar
Grandemange, M., Gohlke, M. & Cadot, O. 2013 Turbulent wake past a three-dimensional blunt body. Part 1. Global modes and bi-stability. J. Fluid Mech. 722, 51–84.CrossRefGoogle Scholar
Grandemange, M., Gohlke, M., Parezanović, V. & Cadot, O. 2012 On experimental sensitivity analysis of the turbulent wake from an axisymmetric blunt trailing edge. Phys. Fluids 24, 035106.CrossRefGoogle Scholar
Holmes, P., Lumley, J. L., Berkooz, G. & Rowley, C. W. 2012 Turbulence, Coherent Structures, Dynamical Systems and Symmetry, 2nd edn. Cambridge University Press.CrossRefGoogle Scholar
Hucho, W.-H.(Ed.) 1998 Aerodynamics of Road Vehicles, 4th edn. Society of Automotive Engineers, SAE.Google Scholar
Lin, N., Reed, H. L. & Saric, W. S. 1992 Effect of leading-edge geometry on boundary-layer receptivity to freestream sound. In Instability, Transition, and Turbulence, pp. 421–440. Springer.CrossRefGoogle Scholar
Lumley, J. L. 1970 Stochastic Tools in Turbulence. Academic Press.Google Scholar
Meliga, P., Chomaz, J.-M. & Sipp, D. 2009 Global mode interaction and pattern selection in the wake of a disk: a weakly nonlinear expansion. J. Fluid Mech. 633, 159–189.CrossRefGoogle Scholar
Natarajan, R. & Acrivos, A. 1993 The instability of the steady flow past spheres and disks. J. Fluid Mech. 254, 323–344.CrossRefGoogle Scholar
Oxlade, A. R.2013 High-frequency periodic jet forcing of a turbulent axisymmetric wake. PhD thesis, Imperial College.Google Scholar
Pier, B. 2008 Local and global instabilities in the wake of a sphere. J. Fluid Mech. 603, 39–62.CrossRefGoogle Scholar
Qubain, A.2009 Active control of a turbulent bluff body wake. PhD thesis, Imperial College.Google Scholar
Sanmiguel-Rojas, E., Sevilla, A., Martínez-Bazán, C. & Chomaz, J.-M. 2009 Global mode analysis of axisymmetric bluff-body wakes: stabilization by base bleed. Phys. Fluids 21, 114102.CrossRefGoogle Scholar
Sevilla, A. & Martínez-Bazán, C. 2004 Vortex shedding in high Reynolds number axisymmetric bluff-body wakes: local linear instability and global bleed control. Phys. Fluids 16, 3460–3469.CrossRefGoogle Scholar
Taneda, S. 1978 Visual observations of the flow past a sphere at Reynolds numbers between $10^4$ and $10^6$ . J. Fluid Mech. 85, 187–192.CrossRefGoogle Scholar
Tomboulides, A. G. & Orszag, S. A. 2000 Numerical investigation of transitional and weak turbulent flow past a sphere. J. Fluid Mech. 416, 45–73.CrossRefGoogle Scholar