Article contents
Taylor dispersion in osmotically driven laminar flows in phloem
Published online by Cambridge University Press: 02 March 2021
Abstract
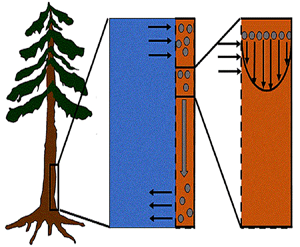
Sucrose is among the main products of photosynthesis that are deemed necessary for plant growth and survival. It is produced in the mesophyll cells of leaves and translocated to different parts of the plant through the phloem. Progress in understanding this transport process remains fraught with experimental difficulties, thereby prompting interest in theoretical approaches and laboratory studies. The Münch pressure and mass flow model is one of the accepted hypotheses describing the physics of sucrose transport in the phloem. It is based on osmosis creating an energy potential difference between the source and the sink. The flow responding to this energy potential is assumed laminar and described by the Hagen–Poiseuille equation. This study revisits such osmotically driven flows in tubes with membrane walls by including the effects of Taylor dispersion on mass transport. This effect has been overlooked in phloem flow studies. Taylor dispersion can increase the effective transport of solutes by increasing the apparent diffusion coefficient. It is shown that, in addition to the conventional diffusive correction derived for impermeable tube walls, a new adjustment to the mean advective terms arises because of osmotic effects. Because the molecular Schmidt number is very large for sucrose in water, the sucrose front speed and travel times have a direct dependence on the Péclet number for different ranges of the Münch number. This study establishes upper limits on expected Taylor dispersion enhancement of sucrose transport.
Information
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2021. Published by Cambridge University Press
References
REFERENCES
- 10
- Cited by


