Article contents
Universality of the energy-containing structures in wall-bounded turbulence
Published online by Cambridge University Press: 21 June 2017
Abstract
The scaling behaviour of the longitudinal velocity structure functions 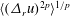 $\langle (\unicode[STIX]{x1D6E5}_{r}u)^{2p}\rangle ^{1/p}$ (where
$\langle (\unicode[STIX]{x1D6E5}_{r}u)^{2p}\rangle ^{1/p}$ (where 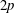 $2p$ represents the order) is studied for various wall-bounded turbulent flows. It has been known that for very large Reynolds numbers within the logarithmic region, the structure functions can be described by
$2p$ represents the order) is studied for various wall-bounded turbulent flows. It has been known that for very large Reynolds numbers within the logarithmic region, the structure functions can be described by 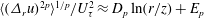 $\langle (\unicode[STIX]{x1D6E5}_{r}u)^{2p}\rangle ^{1/p}/U_{\unicode[STIX]{x1D70F}}^{2}\approx D_{p}\ln (r/z)+E_{p}$ (where
$\langle (\unicode[STIX]{x1D6E5}_{r}u)^{2p}\rangle ^{1/p}/U_{\unicode[STIX]{x1D70F}}^{2}\approx D_{p}\ln (r/z)+E_{p}$ (where  $r$ is the longitudinal distance,
$r$ is the longitudinal distance,  $z$ the distance from the wall,
$z$ the distance from the wall, 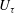 $U_{\unicode[STIX]{x1D70F}}$ the friction velocity and
$U_{\unicode[STIX]{x1D70F}}$ the friction velocity and 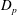 $D_{p}$ ,
$D_{p}$ , 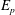 $E_{p}$ are constants) in accordance with Townsend’s attached eddy hypothesis. Here we show that the ratios
$E_{p}$ are constants) in accordance with Townsend’s attached eddy hypothesis. Here we show that the ratios 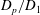 $D_{p}/D_{1}$ extracted from plots between structure functions – in the spirit of the extended self-similarity hypothesis – have further reaching universality for the energy containing range of scales. Specifically, we confirm that this description is universal across wall-bounded flows with different flow geometries, and also for both the longitudinal and transversal structure functions, where previously the scaling has been either difficult to discern or differences have been reported when examining the direct representation of
$D_{p}/D_{1}$ extracted from plots between structure functions – in the spirit of the extended self-similarity hypothesis – have further reaching universality for the energy containing range of scales. Specifically, we confirm that this description is universal across wall-bounded flows with different flow geometries, and also for both the longitudinal and transversal structure functions, where previously the scaling has been either difficult to discern or differences have been reported when examining the direct representation of  $\langle (\unicode[STIX]{x1D6E5}_{r}u)^{2p}\rangle ^{1/p}$ . In addition, we present evidence of this universality at much lower Reynolds numbers, which opens up avenues to examine structure functions that are not readily available from high Reynolds number databases.
$\langle (\unicode[STIX]{x1D6E5}_{r}u)^{2p}\rangle ^{1/p}$ . In addition, we present evidence of this universality at much lower Reynolds numbers, which opens up avenues to examine structure functions that are not readily available from high Reynolds number databases.
JFM classification
Information
- Type
- Papers
- Information
- Copyright
- © 2017 Cambridge University Press
References
- 14
- Cited by


