Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Polet, D T
and
Rival, D E
2015.
Rapid area change in pitch-up manoeuvres of small perching birds.
Bioinspiration & Biomimetics,
Vol. 10,
Issue. 6,
p.
066004.
Steele, S. C.
Dahl, J. M.
Weymouth, G. D.
and
Triantafyllou, M. S.
2016.
Shape of retracting foils that model morphing bodies controls shed energy and wake structure.
Journal of Fluid Mechanics,
Vol. 805,
Issue. ,
p.
355.
Steele, S. C.
Weymouth, G. D.
Dahl, J. M.
and
Triantafyllou, M. S.
2016.
Advances in Fluid-Structure Interaction.
Vol. 133,
Issue. ,
p.
15.
Fernando, J N
and
Rival, D E
2017.
On the dynamics of perching manoeuvres with low-aspect-ratio planforms.
Bioinspiration & Biomimetics,
Vol. 12,
Issue. 4,
p.
046007.
Muscutt, L. E.
Weymouth, G. D.
and
Ganapathisubramani, B.
2017.
Performance augmentation mechanism of in-line tandem flapping foils.
Journal of Fluid Mechanics,
Vol. 827,
Issue. ,
p.
484.
Xia, X.
and
Mohseni, K.
2017.
Unsteady aerodynamics and vortex-sheet formation of a two-dimensional airfoil.
Journal of Fluid Mechanics,
Vol. 830,
Issue. ,
p.
439.
Roderick, William R. T.
Cutkosky, Mark R.
and
Lentink, David
2017.
Touchdown to take-off: at the interface of flight and surface locomotion.
Interface Focus,
Vol. 7,
Issue. 1,
p.
20160094.
Gao, Amy
and
Triantafyllou, Michael S.
2018.
Independent caudal fin actuation enables high energy extraction and control in two-dimensional fish-like group swimming.
Journal of Fluid Mechanics,
Vol. 850,
Issue. ,
p.
304.
Leung, Jordan M.
Wong, Jaime G.
Weymouth, Gabriel D.
and
Rival, David E.
2018.
Modeling Transverse Gusts Using Pitching, Plunging, and Surging Airfoil Motions.
AIAA Journal,
Vol. 56,
Issue. 8,
p.
3271.
Limacher, Eric
Morton, Chris
and
Wood, David
2018.
Generalized derivation of the added-mass and circulatory forces for viscous flows.
Physical Review Fluids,
Vol. 3,
Issue. 1,
Jardin, T.
and
Doué, N.
2019.
Influence of pitch rate on freely translating perching airfoils.
Journal of Fluid Mechanics,
Vol. 873,
Issue. ,
p.
49.
Corkery, S. J.
Babinsky, H.
and
Graham, W. R.
2019.
Quantification of added-mass effects using particle image velocimetry data for a translating and rotating flat plate.
Journal of Fluid Mechanics,
Vol. 870,
Issue. ,
p.
492.
Limacher, Eric
Morton, Chris
and
Wood, David
2019.
On the calculation of force from PIV data using the generalized added-mass and circulatory force decomposition.
Experiments in Fluids,
Vol. 60,
Issue. 1,
Lagopoulos, N. S.
Weymouth, G. D.
and
Ganapathisubramani, B.
2019.
Universal scaling law for drag-to-thrust wake transition in flapping foils.
Journal of Fluid Mechanics,
Vol. 872,
Issue. ,
Johnson, Kyle C.
Thurow, Brian S.
Wabick, Kevin J.
Berdon, Randall L.
and
Buchholz, James H. J.
2020.
Vortex topology of a pitching and rolling wing in forward flight.
Experiments in Fluids,
Vol. 61,
Issue. 10,
Lagopoulos, N. S.
Weymouth, G. D.
and
Ganapathisubramani, B.
2020.
Deflected wake interaction of tandem flapping foils.
Journal of Fluid Mechanics,
Vol. 903,
Issue. ,
Fernando, J.N.
Weymouth, G.D.
and
Rival, D.E.
2020.
On the limits of added-mass theory in separated flows and with varying initial conditions.
Journal of Fluids and Structures,
Vol. 93,
Issue. ,
p.
102835.
Galler, Joshua N.
Rival, David E.
and
Weymouth, Gabriel D.
2020.
Application of the Energized-Mass Concept to Describe Gust-Body Interactions.
Ōtomo, Shūji
Henne, Sabrina
Mulleners, Karen
Ramesh, Kiran
and
Viola, Ignazio Maria
2021.
Unsteady lift on a high-amplitude pitching aerofoil.
Experiments in Fluids,
Vol. 62,
Issue. 1,
Limacher, Eric J.
2021.
Added-mass force on elliptic airfoils.
Journal of Fluid Mechanics,
Vol. 926,
Issue. ,
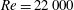 $\mathit{Re}=22\,000$ and two-dimensional simulations at
$\mathit{Re}=22\,000$ and two-dimensional simulations at  $\mathit{Re}=2000$ . Simulated vorticity fields agree with particle image velocimetry measurements, showing the same wake features and vorticity magnitudes. Peak lift and drag forces during rapid perching are measured to be more than 10 times the quasi-steady values. The majority of these forces can be attributed to added-mass energy transfer between the fluid and aerofoil, and to energy lost to the fluid by flow separation at the leading and trailing edges. Thus, despite the large angles of attack and decreasing flow velocity, this simple pitch-up manoeuvre provides a means through which a perching bird can maintain high lift and drag simultaneously while slowing to a controlled stop.
$\mathit{Re}=2000$ . Simulated vorticity fields agree with particle image velocimetry measurements, showing the same wake features and vorticity magnitudes. Peak lift and drag forces during rapid perching are measured to be more than 10 times the quasi-steady values. The majority of these forces can be attributed to added-mass energy transfer between the fluid and aerofoil, and to energy lost to the fluid by flow separation at the leading and trailing edges. Thus, despite the large angles of attack and decreasing flow velocity, this simple pitch-up manoeuvre provides a means through which a perching bird can maintain high lift and drag simultaneously while slowing to a controlled stop.

