Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Moise, Pradeep
2020.
An assessment of theories modeling vortex breakdown as a transition between cylindrical flow states.
Physics of Fluids,
Vol. 32,
Issue. 12,
Vignat, Guillaume
Durox, Daniel
Renaud, Antoine
Lancien, Théa
Vicquelin, Ronan
and
Candel, Sébastien
2021.
Investigation of transient PVC dynamics in a strongly swirled spray flame using high speed planar laser imaging of SnO2 microparticles.
Combustion and Flame,
Vol. 225,
Issue. ,
p.
305.
Rock, Nicholas
Stouffer, Scott D.
Hendershott, Tyler H.
Corporan, Edwin
and
Wrzesinski, Paul
2021.
Characterization of Coherent Flow Structures in a Swirl-Stabilized Spray Combustor.
Moise, Pradeep
and
Mathew, Joseph
2021.
Hysteresis and turbulent vortex breakdown in transitional swirling jets.
Journal of Fluid Mechanics,
Vol. 915,
Issue. ,
Chterev, Ianko
and
Boxx, Isaac
2021.
Effect of hydrogen enrichment on the dynamics of a lean technically premixed elevated pressure flame.
Combustion and Flame,
Vol. 225,
Issue. ,
p.
149.
Douglas, Christopher M.
Emerson, Benjamin L.
and
Lieuwen, Timothy C.
2021.
Nonlinear dynamics of fully developed swirling jets.
Journal of Fluid Mechanics,
Vol. 924,
Issue. ,
Litvinov, I.V.
Suslov, D.A.
Gorelikov, E.U.
and
Shtork, S.I.
2021.
Swirl number and nozzle confinement effects in a flat-vane axial swirler.
International Journal of Heat and Fluid Flow,
Vol. 91,
Issue. ,
p.
108812.
Douglas, Christopher M.
Emerson, Benjamin L.
Hemchandra, Santosh
and
Lieuwen, Timothy C.
2021.
Forced flow response analysis of a turbulent swirling annular jet flame.
Physics of Fluids,
Vol. 33,
Issue. 8,
Karmarkar, Ashwini
Gupta, Saarthak
Boxx, Isaac
Hemchandra, Santosh
and
O'Connor, Jacqueline
2022.
Impact of precessing vortex core dynamics on the thermoacoustic instabilities in a swirl-stabilized combustor.
Journal of Fluid Mechanics,
Vol. 946,
Issue. ,
Wang, Sirui
Zheng, Jianyi
Xu, Liangliang
An, Qiang
Han, Xiao
Zhang, Chi
Li, Lei
Xia, Xi
and
Qi, Fei
2022.
Experimental investigation of the helical mode in a stratified swirling flame.
Combustion and Flame,
Vol. 244,
Issue. ,
p.
112268.
Kumar, Sonu
and
Basu, Saptarshi
2022.
Insight into the flow dynamics of a high shear injector equipped with center-body: Suppression of precessing vortex core oscillations.
Physics of Fluids,
Vol. 34,
Issue. 11,
Douglas, Christopher M.
and
Lesshafft, Lutz
2022.
Confinement effects in laminar swirling jets.
Journal of Fluid Mechanics,
Vol. 945,
Issue. ,
Mardani, Amir
Asadi, Benyamin
and
Beige, Amir A.
2022.
Investigation of flame structure and precessing vortex core instability of a gas turbine model combustor with different swirler configurations.
Physics of Fluids,
Vol. 34,
Issue. 8,
Douglas, Christopher M.
Emerson, Benjamin L.
and
Lieuwen, Timothy C.
2022.
Dynamics and bifurcations of laminar annular swirling and non-swirling jets.
Journal of Fluid Mechanics,
Vol. 943,
Issue. ,
Datta, Anindya
Gupta, Saarthak
Chterev, Ianko
Boxx, Isaac
and
Hemchandra, Santosh
2022.
Impact of Hydrogen Addition on the Thermoacoustic Instability and Precessing Vortex Core Dynamics in a CH4/H2/Air Technically Premixed Combustor.
Journal of Engineering for Gas Turbines and Power,
Vol. 144,
Issue. 2,
Keeton, Benjamin W.
Carpio, Jaime
Nomura, Keiko K.
Sánchez, Antonio L.
and
Williams, Forman A.
2022.
Vortex breakdown in variable-density gaseous swirling jets.
Journal of Fluid Mechanics,
Vol. 936,
Issue. ,
Karmarkar, Ashwini
Boxx, Isaac
and
O'Connor, Jacqueline
2022.
Relative Effects of Velocity- and Mixture-Coupling in a Thermoacoustically Unstable, Partially Premixed Flame.
Journal of Engineering for Gas Turbines and Power,
Vol. 144,
Issue. 1,
Sahu, Toshan Lal
Chetan, Ujjwal
Mahato, Jagannath
Kar, Prabir Kumar
Das, Prasanta Kumar
and
Lakkaraju, Rajaram
2022.
Formation and breakup of twisting ligaments in a viscous swirling liquid jet.
Physics of Fluids,
Vol. 34,
Issue. 11,
Vignat, Guillaume
Durox, Daniel
and
Candel, Sébastien
2022.
The suitability of different swirl number definitions for describing swirl flows: Accurate, common and (over-) simplified formulations.
Progress in Energy and Combustion Science,
Vol. 89,
Issue. ,
p.
100969.
Gupta, Saarthak
Shanbhogue, Santosh
Shimura, Masayasu
Ghoniem, Ahmed
and
Hemchandra, Santosh
2022.
Impact of a Centerbody on the Unsteady Flow Dynamics of a Swirl Nozzle: Intermittency of Precessing Vortex Core Oscillations.
Journal of Engineering for Gas Turbines and Power,
Vol. 144,
Issue. 2,
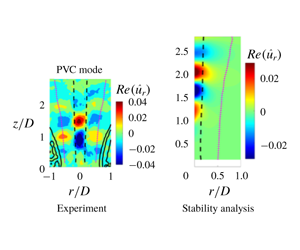
 $S$. Time-resolved velocity measurements in a radial–axial plane anchored at the jet exit for various
$S$. Time-resolved velocity measurements in a radial–axial plane anchored at the jet exit for various  $S$ values are obtained using stereoscopic particle image velocimetry. Spectral proper orthogonal decomposition and spatial cross-spectral analysis reveal the simultaneous emergence of a bubble-type vortex breakdown and a strong helical limit-cycle oscillation in the flow for
$S$ values are obtained using stereoscopic particle image velocimetry. Spectral proper orthogonal decomposition and spatial cross-spectral analysis reveal the simultaneous emergence of a bubble-type vortex breakdown and a strong helical limit-cycle oscillation in the flow for  $S>S_{c}$ where
$S>S_{c}$ where  $S_{c}=0.61$. The oscillation frequency,
$S_{c}=0.61$. The oscillation frequency,  $f_{PVC}$, and the square of the flow oscillation amplitudes vary linearly with
$f_{PVC}$, and the square of the flow oscillation amplitudes vary linearly with  $S-S_{c}$. A solution for the coherent unsteady field accurate up to
$S-S_{c}$. A solution for the coherent unsteady field accurate up to  $O(\unicode[STIX]{x1D716}^{3})$ (
$O(\unicode[STIX]{x1D716}^{3})$ ( $\unicode[STIX]{x1D716}\sim O((S-S_{c})^{1/2})$) is determined from the nonlinear Navier–Stokes equations, using the method of multiple scales. We show that onset of bubble type vortex breakdown at
$\unicode[STIX]{x1D716}\sim O((S-S_{c})^{1/2})$) is determined from the nonlinear Navier–Stokes equations, using the method of multiple scales. We show that onset of bubble type vortex breakdown at  $S_{c}$, results in a marginally stable, helical linear global hydrodynamic mode. This results in the stable limit-cycle precession of the breakdown bubble. The variation of
$S_{c}$, results in a marginally stable, helical linear global hydrodynamic mode. This results in the stable limit-cycle precession of the breakdown bubble. The variation of  $f_{LC}$ with
$f_{LC}$ with  $S-S_{c}$ is determined from the Stuart–Landau equation associated with the PVC. Reasonable agreement with the corresponding experimental result is observed, despite the highly turbulent nature of the flow in the present experiment. Further, amplitude saturation results from the time-averaged distortion imposed on the flow by the PVC, suggesting that linear stability analysis may predict PVC characteristics for
$S-S_{c}$ is determined from the Stuart–Landau equation associated with the PVC. Reasonable agreement with the corresponding experimental result is observed, despite the highly turbulent nature of the flow in the present experiment. Further, amplitude saturation results from the time-averaged distortion imposed on the flow by the PVC, suggesting that linear stability analysis may predict PVC characteristics for  $S>S_{c}$.
$S>S_{c}$.
