Hostname: page-component-76c49bb84f-lvrpm Total loading time: 0 Render date: 2025-07-07T08:15:18.887Z Has data issue: false hasContentIssue false
  • English
  • Français

Nonlinear effects in buoyancy-driven variable-density turbulence

Published online by Cambridge University Press:  25 November 2016

P. Rao ,
C. P. Caulfield  and
J. D. Gibbon
P. Rao*
Affiliation:
Department of Applied Mathematics and Statistics, State University of New York, Stony Brook, NY 11790, USA
C. P. Caulfield
Affiliation:
BP Institute, University of Cambridge, Madingley Rise, Madingley Road, Cambridge CB3 0EZ, UK Department of Applied Mathematics & Theoretical Physics, University of Cambridge, Centre for Mathematical Sciences, Wilberforce Road, Cambridge CB3 0WA, UK
J. D. Gibbon
Affiliation:
Department of Mathematics, Imperial College London, London SW7 2AZ, UK
*
†Email address for correspondence: prao@ams.sunysb.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

We consider the time dependence of a hierarchy of scaled 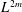$L^{2m}$-norms $D_{m,\unicode[STIX]{x1D714}}$ and 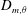$D_{m,\unicode[STIX]{x1D703}}$ of the vorticity 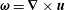$\unicode[STIX]{x1D74E}=\unicode[STIX]{x1D735}\times \boldsymbol{u}$ and the density gradient 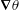$\unicode[STIX]{x1D735}\unicode[STIX]{x1D703}$, where 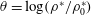$\unicode[STIX]{x1D703}=\log (\unicode[STIX]{x1D70C}^{\ast }/\unicode[STIX]{x1D70C}_{0}^{\ast })$, in a buoyancy-driven turbulent flow as simulated by Livescu & Ristorcelli (J. Fluid Mech., vol. 591, 2007, pp. 43–71). Here, 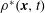$\unicode[STIX]{x1D70C}^{\ast }(\boldsymbol{x},t)$ is the composition density of a mixture of two incompressible miscible fluids with fluid densities $\unicode[STIX]{x1D70C}_{2}^{\ast }>\unicode[STIX]{x1D70C}_{1}^{\ast }$, and 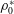$\unicode[STIX]{x1D70C}_{0}^{\ast }$ is a reference normalization density. Using data from the publicly available Johns Hopkins turbulence database, we present evidence that the 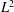$L^{2}$-spatial average of the density gradient 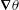$\unicode[STIX]{x1D735}\unicode[STIX]{x1D703}$ can reach extremely large values at intermediate times, even in flows with low Atwood number 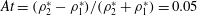$At=(\unicode[STIX]{x1D70C}_{2}^{\ast }-\unicode[STIX]{x1D70C}_{1}^{\ast })/(\unicode[STIX]{x1D70C}_{2}^{\ast }+\unicode[STIX]{x1D70C}_{1}^{\ast })=0.05$, implying that very strong mixing of the density field at small scales can arise in buoyancy-driven turbulence. This large growth raises the possibility that the density gradient 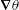$\unicode[STIX]{x1D735}\unicode[STIX]{x1D703}$ might blow up in a finite time.

JFM classification

Convection: Buoyancy-driven instability Mathematical Foundations: Mathematical Foundations

Information

Type
Papers
Information
Journal of Fluid Mechanics , Volume 810 , 10 January 2017 , pp. 362 - 377
Check for updates
Copyright
© 2016 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Andrews, M. J. & Dalziel, S. B. 2010 Small Atwood number Rayleigh–Taylor experiments. Phil. Trans. R. Soc. Lond. A 368 (1916), 1663–1679.Google Scholar
Cabot, W. H. & Cook, A. W. 2006 Reynolds number effects on Rayleigh–Taylor instability with possible implications for type Ia supernovae. Nat. Phys. 2 (8), 562–568.Google Scholar
Cook, A. W. & Dimotakis, P. E. 2001 Transition stages of Rayleigh–Taylor instability between miscible fluids. J. Fluid Mech. 443, 69–99.Google Scholar
Davies-Wykes, M. S. & Dalziel, S. B. 2014 Efficient mixing in stratified flows: experimental study of a Rayleigh–Taylor unstable interface within an otherwise stable stratification. J. Fluid Mech. 756, 1027–1057.CrossRefGoogle Scholar
Dimonte, G., Youngs, D. L., Dimits, A., Weber, S., Marinak, M., Wunsch, S., Garasi, C., Robinson, A., Andrews, M. J., Ramaprabhu, P. et al. 2004 A comparative study of the turbulent Rayleigh–Taylor instability using high-resolution three-dimensional numerical simulations: the Alpha-Group collaboration. Phys. Fluids 16, 1668–1693.Google Scholar
Dimotakis, P. E. 2005 Turbulent mixing. Annu. Rev. Fluid Mech. 37, 329–356.CrossRefGoogle Scholar
Donzis, D. A., Gibbon, J. D., Kerr, R. M., Gupta, A., Pandit, R. & Vincenzi, D. 2013 Vorticity moments in four numerical simulations of the 3D Navier–Stokes equations. J. Fluid Mech. 732, 316–331.Google Scholar
Gibbon, J. D. 2015 High–low frequency slaving and regularity issues in the 3D Navier–Stokes equations. IMA J. Appl. Maths 81, 308–320.CrossRefGoogle Scholar
Gibbon, J. D., Donzis, D. A., Kerr, R. M., Gupta, A., Pandit, R. & Vincenzi, D. 2014 Regimes of nonlinear depletion and regularity in the 3D Navier–Stokes equations. Nonlinearity 27, 1–19.Google Scholar
Glimm, J., Grove, J. W., Li, X. L., Oh, W. & Sharp, D. H. 2001 A critical analysis of Rayleigh–Taylor growth rates. J. Comput. Phys. 169 (2), 652–677.Google Scholar
Hyunsun, L., Hyeonseong, J., Yan, Y. & Glimm, J. 2008 On validation of turbulent mixing simulations for Rayleigh–Taylor instability. Phys. Fluids 20, 012102.Google Scholar
Lawrie, A. G. W. & Dalziel, S. B. 2011 Rayleigh–Taylor mixing in an otherwise stable stratification. J. Fluid Mech. 688, 507–527.Google Scholar
Lee, H., Jin, H., Yu, Y. & Glimm, J. 2008 On validation of turbulent mixing simulations for Rayleigh–Taylor instability. Phys. Fluids 20, 1–8.Google Scholar
Livescu, D. 2013 Numerical simulations of two-fluid mixing at large density ratios and applications to the Rayleigh–Taylor instability. Phil. Trans. R. Soc. Lond. A 371, 20120185.Google Scholar
Livescu, D., Canada, C., Kanov, K., Burns, R. & Pulido, J.2014 Homogeneous buoyancy driven turbulence data set. LA-UR-14-20669.Google Scholar
Livescu, D., Mohd-Yusof, J., Petersen, M. R. & Grove, J. W.2009 A computer code for direct numerical simulation of turbulent flows. Tech. Rep. LA-CC-09-100. Los Alamos National Laboratory.Google Scholar
Livescu, D. & Ristorcelli, J. R. 2007 Buoyancy-driven variable-density turbulence. J. Fluid Mech. 591, 43–71.Google Scholar
Livescu, D. & Ristorcelli, J. R. 2008 Variable-density mixing in buoyancy-driven turbulence. J. Fluid Mech. 605, 145–180.Google Scholar
Luo, G. & Hou, T. 2014a Potentially sinugular solutions of the 3D axisymmetric Euler equations. Proc. Natl Acad. Sci. USA 111, 12968–12973.Google Scholar
Luo, G. & Hou, T. 2014b Toward the finite time blow-up of the 3D incompressible Euler equations: a numerical investigation. Multiscale Model. Simul. 12, 1722–1776.Google Scholar
Petrasso, R. D. 1994 Rayleigh’s challenge endures. Nat. Phys. 367 (6460), 217–218.Google Scholar
Rayleigh, Lord 1900 Investigation of the character of the equilibrium of an incompressible heavy fluid of variable density. In Scientific Papers, vol. 2, p. 598. Cambridge University Press.Google Scholar
Sharp, D. H. 1984 An overview of Rayleigh–Taylor instability. Phys. D 12D, 3–18.Google Scholar
Tailleux, R. 2013 Available potential energy and exergy in stratified fluids. Annu. Rev. Fluid Mech. 45, 35–58.Google Scholar
Taylor, G. I. 1950 The instability of liquid surfaces when accelerated in a direction perpendicular to their planes. I. Proc. R. Soc. Lond A 201 (1065), 192–196.Google Scholar
Youngs, D. L. 1984 Numerical simulation of turbulent mixing by Rayleigh–Taylor instability. Phys. D 12 (1–3), 32–44.Google Scholar
Youngs, D. L. 1989 Modelling turbulent mixing by Rayleigh–Taylor instability. Phys. D 37, 270–287.Google Scholar