Article contents
Dynamics of poles in two-dimensional hydrodynamics with free surface: new constants of motion
Published online by Cambridge University Press: 12 July 2019
Abstract
We address the problem of the potential motion of an ideal incompressible fluid with a free surface and infinite depth in a two-dimensional geometry. We admit the presence of gravity forces and surface tension. A time-dependent conformal mapping 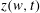 $z(w,t)$ of the lower complex half-plane of the variable
$z(w,t)$ of the lower complex half-plane of the variable 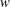 $w$ into the area filled with fluid is performed with the real line of
$w$ into the area filled with fluid is performed with the real line of 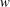 $w$ mapped into the free fluid’s surface. We study the dynamics of singularities of both
$w$ mapped into the free fluid’s surface. We study the dynamics of singularities of both 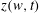 $z(w,t)$ and the complex fluid potential
$z(w,t)$ and the complex fluid potential 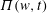 $\unicode[STIX]{x1D6F1}(w,t)$ in the upper complex half-plane of
$\unicode[STIX]{x1D6F1}(w,t)$ in the upper complex half-plane of  $w$. We show the existence of solutions with an arbitrary finite number
$w$. We show the existence of solutions with an arbitrary finite number 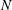 $N$ of complex poles in
$N$ of complex poles in  $z_{w}(w,t)$ and
$z_{w}(w,t)$ and 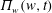 $\unicode[STIX]{x1D6F1}_{w}(w,t)$ which are the derivatives of
$\unicode[STIX]{x1D6F1}_{w}(w,t)$ which are the derivatives of  $z(w,t)$ and
$z(w,t)$ and 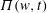 $\unicode[STIX]{x1D6F1}(w,t)$ over
$\unicode[STIX]{x1D6F1}(w,t)$ over 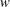 $w$. We stress that these solutions are not purely rational because they generally have branch points at other positions of the upper complex half-plane. The orders of poles can be arbitrary for zero surface tension while all orders are even for non-zero surface tension. We find that the residues of
$w$. We stress that these solutions are not purely rational because they generally have branch points at other positions of the upper complex half-plane. The orders of poles can be arbitrary for zero surface tension while all orders are even for non-zero surface tension. We find that the residues of 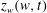 $z_{w}(w,t)$ at these
$z_{w}(w,t)$ at these 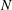 $N$ points are new, previously unknown, constants of motion, see also Zakharov & Dyachenko (2012, authors’ unpublished observations, arXiv:1206.2046) for the preliminary results. All these constants of motion commute with each other in the sense of the underlying Hamiltonian dynamics. In the absence of both gravity and surface tension, the residues of
$N$ points are new, previously unknown, constants of motion, see also Zakharov & Dyachenko (2012, authors’ unpublished observations, arXiv:1206.2046) for the preliminary results. All these constants of motion commute with each other in the sense of the underlying Hamiltonian dynamics. In the absence of both gravity and surface tension, the residues of 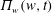 $\unicode[STIX]{x1D6F1}_{w}(w,t)$ are also the constants of motion while non-zero gravity
$\unicode[STIX]{x1D6F1}_{w}(w,t)$ are also the constants of motion while non-zero gravity 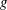 $g$ ensures a trivial linear dependence of these residues on time. A Laurent series expansion of both
$g$ ensures a trivial linear dependence of these residues on time. A Laurent series expansion of both  $z_{w}(w,t)$ and
$z_{w}(w,t)$ and 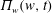 $\unicode[STIX]{x1D6F1}_{w}(w,t)$ at each poles position reveals the existence of additional integrals of motion for poles of the second order. If all poles are simple then the number of independent real integrals of motion is
$\unicode[STIX]{x1D6F1}_{w}(w,t)$ at each poles position reveals the existence of additional integrals of motion for poles of the second order. If all poles are simple then the number of independent real integrals of motion is 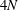 $4N$ for zero gravity and
$4N$ for zero gravity and  $4N-1$ for non-zero gravity. For the second-order poles we found
$4N-1$ for non-zero gravity. For the second-order poles we found  $6N$ motion integrals for zero gravity and
$6N$ motion integrals for zero gravity and  $6N-1$ for non-zero gravity. We suggest that the existence of these non-trivial constants of motion provides an argument in support of the conjecture of complete integrability of free surface hydrodynamics in deep water. Analytical results are solidly supported by high precision numerics.
$6N-1$ for non-zero gravity. We suggest that the existence of these non-trivial constants of motion provides an argument in support of the conjecture of complete integrability of free surface hydrodynamics in deep water. Analytical results are solidly supported by high precision numerics.
Information
- Type
- JFM Papers
- Information
- Copyright
- © 2019 Cambridge University Press
References
- 17
- Cited by


