2 The incompressible staggered hollow vortex street

For steady incompressible motion of an inviscid fluid, it is known (Saffman Reference Saffman1992) that, in a two-dimensional irrotational flow, a streamfunction $\unicode[STIX]{x1D713}(x,y)$ and a velocity potential $\unicode[STIX]{x1D719}(x,y)$ exist satisfying the Cauchy–Riemann equations

(2.1a,b ) $$\begin{eqnarray}u={\displaystyle \frac{\unicode[STIX]{x2202}\unicode[STIX]{x1D713}}{\unicode[STIX]{x2202}y}}={\displaystyle \frac{\unicode[STIX]{x2202}\unicode[STIX]{x1D719}}{\unicode[STIX]{x2202}x}}\quad \text{and}\quad v=-{\displaystyle \frac{\unicode[STIX]{x2202}\unicode[STIX]{x1D713}}{\unicode[STIX]{x2202}x}}={\displaystyle \frac{\unicode[STIX]{x2202}\unicode[STIX]{x1D719}}{\unicode[STIX]{x2202}y}},\end{eqnarray}$$

where the velocity field is denoted by $(u,v)$ . It follows that $\unicode[STIX]{x1D719}(x,y)$ and $\unicode[STIX]{x1D713}(x,y)$ are harmonic functions. One can introduce the complex potential $f(z)\equiv \unicode[STIX]{x1D719}+\text{i}\unicode[STIX]{x1D713}$ , which is an analytic function of the complex variable $z=x+\text{i}y$ . The (complex) velocity field is then given by its derivative:

(2.2) $$\begin{eqnarray}\unicode[STIX]{x1D709}(z)=u-\text{i}v={\displaystyle \frac{\text{d}f}{\text{d}z}}.\end{eqnarray}$$

Figure 1.

Conformal mapping $z_{0}(\unicode[STIX]{x1D701})$ from the preimage cut annulus $\unicode[STIX]{x1D70C}_{0}<|\unicode[STIX]{x1D701}|<1$ to a typical period window of a staggered hollow vortex street. The two sides of the branch cut joining $\unicode[STIX]{x1D6FC}_{0}$ and $\unicode[STIX]{x1D6FD}_{0}$ are mapped by $z_{0}(\unicode[STIX]{x1D701})$ to the two edges of the period window. The two circles $|\unicode[STIX]{x1D701}|=\unicode[STIX]{x1D70C}_{0},1$ each map to one of the hollow vortex boundaries. Shown on the right is the incompressible solution of Crowdy & Green (Reference Crowdy and Green2011) described by (2.4) with parameters $L=\unicode[STIX]{x1D6E4}=1,\unicode[STIX]{x1D70C}_{0}=0.28,\unicode[STIX]{x1D6FC}_{0}=0.347,\unicode[STIX]{x1D6FD}_{0}=-0.806,\unicode[STIX]{x1D6FE}_{1}=-0.615$ and $\unicode[STIX]{x1D6FE}_{2}=0.456$ , corresponding to a street travelling with speed $U=0.316$ .

The incompressible solutions for staggered hollow vortex streets, comprising vortices with period $L$ and circulation $\pm \unicode[STIX]{x1D6E4}$ travelling at speed $U$ , were found as a function of a parametric conformal mapping variable $\unicode[STIX]{x1D701}$ from the annulus shown in figure 1 by Crowdy & Green (Reference Crowdy and Green2011). The solutions for staggered streets travelling with speed $U\neq 0$ can be written

(2.3) $$\begin{eqnarray}\displaystyle & \displaystyle f=f_{0}(\unicode[STIX]{x1D701})=\frac{\text{i}LU}{2\unicode[STIX]{x03C0}}\log \left[\frac{|\unicode[STIX]{x1D6FC}_{0}|P(\unicode[STIX]{x1D701}/\unicode[STIX]{x1D6FC}_{0},\unicode[STIX]{x1D70C}_{0})P(\unicode[STIX]{x1D701}\unicode[STIX]{x1D6FD}_{0},\unicode[STIX]{x1D70C}_{0})}{|\unicode[STIX]{x1D6FD}_{0}|P(\unicode[STIX]{x1D701}\unicode[STIX]{x1D6FC}_{0},\unicode[STIX]{x1D70C}_{0})P(\unicode[STIX]{x1D701}/\unicode[STIX]{x1D6FD}_{0},\unicode[STIX]{x1D70C}_{0})}\right]-\frac{\text{i}\unicode[STIX]{x1D6E4}}{2\unicode[STIX]{x03C0}}\log \unicode[STIX]{x1D701}, & \displaystyle\end{eqnarray}$$

(2.4) $$\begin{eqnarray}\displaystyle & \displaystyle z=z_{0}(\unicode[STIX]{x1D701})=-\frac{\text{i}L}{2\unicode[STIX]{x03C0}}\left[\log \left[\frac{P(\unicode[STIX]{x1D701}/\unicode[STIX]{x1D6FC}_{0},\unicode[STIX]{x1D70C}_{0})}{P(\unicode[STIX]{x1D701}/\unicode[STIX]{x1D6FD}_{0},\unicode[STIX]{x1D70C}_{0})}\right]-\unicode[STIX]{x1D712}\log \left[\frac{P(\unicode[STIX]{x1D701}\unicode[STIX]{x1D6FC}_{0},\unicode[STIX]{x1D70C}_{0})}{P(\unicode[STIX]{x1D701}\unicode[STIX]{x1D6FD}_{0},\unicode[STIX]{x1D70C}_{0})}\right]\right]+\frac{\text{i}L}{4\unicode[STIX]{x03C0}}\log (\unicode[STIX]{x1D6FD}_{0}/\unicode[STIX]{x1D6FC}_{0}),\qquad & \displaystyle\end{eqnarray}$$

(2.5) $$\begin{eqnarray}\displaystyle & \displaystyle u-\text{i}v=\frac{\text{d}f}{\text{d}z}=\unicode[STIX]{x1D709}_{0}(\unicode[STIX]{x1D701})=-\frac{U\unicode[STIX]{x1D70C}_{0}}{\sqrt{\unicode[STIX]{x1D712}}\unicode[STIX]{x1D701}}\left[\frac{P(\unicode[STIX]{x1D701}/\unicode[STIX]{x1D6FE}_{1},\unicode[STIX]{x1D70C}_{0})P(\unicode[STIX]{x1D701}/\unicode[STIX]{x1D6FE}_{2},\unicode[STIX]{x1D70C}_{0})}{P(\unicode[STIX]{x1D701}\unicode[STIX]{x1D6FE}_{1},\unicode[STIX]{x1D70C}_{0})P(\unicode[STIX]{x1D701}\unicode[STIX]{x1D6FE}_{2},\unicode[STIX]{x1D70C}_{0})}\right], & \displaystyle\end{eqnarray}$$

where $f$ is the complex potential. In these formulae,

(2.6) $$\begin{eqnarray}\unicode[STIX]{x1D712}\equiv \frac{\unicode[STIX]{x1D70C}_{0}^{2}}{\unicode[STIX]{x1D6FC}_{0}^{2}}\left[\frac{P^{2}(\unicode[STIX]{x1D6FC}_{0}/\unicode[STIX]{x1D6FE}_{1},\unicode[STIX]{x1D70C}_{0})P^{2}(\unicode[STIX]{x1D6FC}_{0}/\unicode[STIX]{x1D6FE}_{2},\unicode[STIX]{x1D70C}_{0})}{P^{2}(\unicode[STIX]{x1D6FC}_{0}\unicode[STIX]{x1D6FE}_{1},\unicode[STIX]{x1D70C}_{0})P^{2}(\unicode[STIX]{x1D6FC}_{0}\unicode[STIX]{x1D6FE}_{2},\unicode[STIX]{x1D70C}_{0})}\right]\end{eqnarray}$$

and the real parameters $\unicode[STIX]{x1D70C}_{0},\unicode[STIX]{x1D6FC}_{0},\unicode[STIX]{x1D6FD}_{0},\unicode[STIX]{x1D6FE}_{1}$ and $\unicode[STIX]{x1D6FE}_{2}$ satisfy

(2.7) $$\begin{eqnarray}\unicode[STIX]{x1D6FC}_{0}\unicode[STIX]{x1D6FD}_{0}=-\unicode[STIX]{x1D70C}_{0}=\unicode[STIX]{x1D6FE}_{1}\unicode[STIX]{x1D6FE}_{2}\end{eqnarray}$$

as well as the two conditions

(2.8) $$\begin{eqnarray}\displaystyle & \displaystyle 0=-K(\unicode[STIX]{x1D6FE}_{1}\unicode[STIX]{x1D6FC}_{0},\unicode[STIX]{x1D70C}_{0})-\unicode[STIX]{x1D712}K(\unicode[STIX]{x1D6FC}_{0}/\unicode[STIX]{x1D6FE}_{1},\unicode[STIX]{x1D70C}_{0})+K(\unicode[STIX]{x1D6FE}_{1}\unicode[STIX]{x1D6FD}_{0},\unicode[STIX]{x1D70C}_{0})+\unicode[STIX]{x1D712}K(\unicode[STIX]{x1D6FD}_{0}/\unicode[STIX]{x1D6FE}_{1},\unicode[STIX]{x1D70C}_{0}), & \displaystyle\end{eqnarray}$$

(2.9) $$\begin{eqnarray}\displaystyle & \displaystyle \frac{\unicode[STIX]{x1D6E4}}{LU}=K(\unicode[STIX]{x1D6FE}_{1}/\unicode[STIX]{x1D6FC}_{0},\unicode[STIX]{x1D70C}_{0})-K(\unicode[STIX]{x1D6FE}_{1}/\unicode[STIX]{x1D6FD}_{0},\unicode[STIX]{x1D70C}_{0})-K(\unicode[STIX]{x1D6FE}_{1}\unicode[STIX]{x1D6FC}_{0},\unicode[STIX]{x1D70C}_{0})+K(\unicode[STIX]{x1D6FE}_{1}\unicode[STIX]{x1D6FD}_{0},\unicode[STIX]{x1D70C}_{0}). & \displaystyle\end{eqnarray}$$

The constant speed $q_{0}$ of the fluid on both hollow vortex boundaries can be shown to be

(2.10) $$\begin{eqnarray}q_{0}=\frac{U}{\sqrt{\unicode[STIX]{x1D712}}}.\end{eqnarray}$$

For given $\unicode[STIX]{x1D6E4},L$ and $U$ , these solutions depend on five mathematical parameters: $\unicode[STIX]{x1D70C}_{0},\unicode[STIX]{x1D6FC}_{0},\unicode[STIX]{x1D6FD}_{0},\unicode[STIX]{x1D6FE}_{1}$ and $\unicode[STIX]{x1D6FE}_{2}$ . We can fix one of these parameters, $\unicode[STIX]{x1D70C}_{0}$ say, and this corresponds to picking the area of the hollow vortices making up the street (note that they all have the same area). The four remaining parameters are then determined by solving the four equations (2.7)–(2.9). Constructed this way, the centroid of the vortices, and hence the aspect ratio of the street, will emerge from the solution. Alternatively, one can assign $\unicode[STIX]{x1D6E4}$ and $L$ and insist that the aspect ratio of the street takes a particular value; this geometrical condition replaces (2.9) as one of the equations to determine the four parameters $\unicode[STIX]{x1D6FC}_{0},\unicode[STIX]{x1D6FD}_{0},\unicode[STIX]{x1D6FE}_{1}$ and $\unicode[STIX]{x1D6FE}_{2}$ . In such cases, equation (2.9) is used a posteriori to determine the associated street speed $U$ .

The special functions $P(\unicode[STIX]{x1D701},\unicode[STIX]{x1D70C}_{0})$ and $K(\unicode[STIX]{x1D701},\unicode[STIX]{x1D70C}_{0})$ , which are related to the so-called Schottky–Klein prime function of the annulus $\unicode[STIX]{x1D70C}_{0}<|\unicode[STIX]{x1D701}|<1$ , are defined in appendix A, where their important properties are also given.

The expression for $z_{0}(\unicode[STIX]{x1D701})$ in (2.4) differs from that given by Crowdy & Green (Reference Crowdy and Green2011), where it was reported as an indefinite integral. During the course of this compressible flow analysis, it was realized that the latter integral can be calculated explicitly, leading to (2.4). Appendix B gives details of how to perform this integration. It requires knowledge of the properties of $P(\unicode[STIX]{x1D701},\unicode[STIX]{x1D70C}_{0})$ and $K(\unicode[STIX]{x1D701},\unicode[STIX]{x1D70C}_{0})$ given in appendix A.

The two boundary circles $|\unicode[STIX]{x1D701}|=\unicode[STIX]{x1D70C}_{0},1$ are transplanted under the mapping function $z=z_{0}(\unicode[STIX]{x1D701})$ to the two hollow vortex boundaries; see figure 1. Owing to the presence of the logarithmic branch points at $\unicode[STIX]{x1D6FC}_{0}$ and $\unicode[STIX]{x1D6FD}_{0}$ of the mapping $z_{0}(\unicode[STIX]{x1D701})$ , the latter function is required to be single-valued and one-to-one everywhere in the annulus $\unicode[STIX]{x1D70C}_{0}<|\unicode[STIX]{x1D701}|<1$ outside a branch cut between $\unicode[STIX]{x1D6FC}_{0}$ and $\unicode[STIX]{x1D6FD}_{0}$ . The images of the two sides of that branch cut under the mapping $z_{0}(\unicode[STIX]{x1D701})$ will be the two sides of the period window. For convenience we refer to the region in the annulus $\unicode[STIX]{x1D70C}_{0}<|\unicode[STIX]{x1D701}|<1$ exterior to this branch cut as the ‘cut annulus’. The mapping satisfies the functional identity

(2.11) $$\begin{eqnarray}z_{0}(-\unicode[STIX]{x1D70C}_{0}/\unicode[STIX]{x1D701})=-z_{0}(\unicode[STIX]{x1D701}),\end{eqnarray}$$

which ensures the rotational symmetry of the two vortices about the origin.

3 Compressible flow equations

When the flow becomes compressible, a new variable enters the analysis: the density of the fluid $\unicode[STIX]{x1D708}(z,\overline{z})$ , which is now a function of space (and, for unsteady flow, also of time). Conservation of mass, for a steady flow, requires that

(3.1) $$\begin{eqnarray}\frac{\unicode[STIX]{x2202}(\unicode[STIX]{x1D708}u)}{\unicode[STIX]{x2202}x}+\frac{\unicode[STIX]{x2202}(\unicode[STIX]{x1D708}v)}{\unicode[STIX]{x2202}y}=0,\end{eqnarray}$$

which is clearly invariant to a rescaling of the fluid density. If we let $\unicode[STIX]{x1D708}_{0}$ be the (constant) incompressible density, then we have the freedom to specify this at our convenience, and this will be done later.

As for the dynamics, it is known (Saffman Reference Saffman1992) that, in the absence of body forces, the steady vorticity equation for a barotropic fluid – defined to be one for which pressure is just a function of density, i.e. $p=p(\unicode[STIX]{x1D708})$ – admits an irrotational flow as a possible solution; this means that $\unicode[STIX]{x1D714}=\unicode[STIX]{x2202}v/\unicode[STIX]{x2202}x-\unicode[STIX]{x2202}u/\unicode[STIX]{x2202}y=0$ . Here we will seek a solution for a steady compressible flow that is free of vorticity except for non-zero circulations around hollow vortices. Since the flow is still two-dimensional and irrotational, we can introduce a velocity potential $\unicode[STIX]{x1D719}$ and a streamfunction $\unicode[STIX]{x1D713}$ , but these are now related by the modified system

(3.2a,b ) $$\begin{eqnarray}u={\displaystyle \frac{\unicode[STIX]{x1D708}_{0}}{\unicode[STIX]{x1D708}}}{\displaystyle \frac{\unicode[STIX]{x2202}\unicode[STIX]{x1D713}}{\unicode[STIX]{x2202}y}}={\displaystyle \frac{\unicode[STIX]{x2202}\unicode[STIX]{x1D719}}{\unicode[STIX]{x2202}x}}\quad \text{and}\quad v=-{\displaystyle \frac{\unicode[STIX]{x1D708}_{0}}{\unicode[STIX]{x1D708}}}{\displaystyle \frac{\unicode[STIX]{x2202}\unicode[STIX]{x1D713}}{\unicode[STIX]{x2202}x}}={\displaystyle \frac{\unicode[STIX]{x2202}\unicode[STIX]{x1D719}}{\unicode[STIX]{x2202}y}};\end{eqnarray}$$

$\unicode[STIX]{x1D719}(x,y)$ and $\unicode[STIX]{x1D713}(x,y)$ are no longer harmonic. We can still define the complex potential as $f(z,\overline{z})=\unicode[STIX]{x1D719}+\text{i}\unicode[STIX]{x1D713}$ , but it is not an analytic function of $z$ . Despite this loss of analyticity, we can continue to employ the two independent variables, $z$ and $\overline{z}$ , where the overbar denotes complex conjugation. On noting that

(3.3a,b ) $$\begin{eqnarray}\frac{\unicode[STIX]{x2202}}{\unicode[STIX]{x2202}z}=\frac{1}{2}\left[\frac{\unicode[STIX]{x2202}}{\unicode[STIX]{x2202}x}-\text{i}\frac{\unicode[STIX]{x2202}}{\unicode[STIX]{x2202}y}\right],\quad \frac{\unicode[STIX]{x2202}}{\unicode[STIX]{x2202}\overline{z}}=\frac{1}{2}\left[\frac{\unicode[STIX]{x2202}}{\unicode[STIX]{x2202}x}+\text{i}\frac{\unicode[STIX]{x2202}}{\unicode[STIX]{x2202}y}\right],\end{eqnarray}$$

it is readily shown from (3.2) that

(3.4a,b ) $$\begin{eqnarray}2\frac{\unicode[STIX]{x2202}f}{\unicode[STIX]{x2202}\overline{z}}=\left(1-\frac{\unicode[STIX]{x1D708}}{\unicode[STIX]{x1D708}_{0}}\right)(u+\text{i}v),\quad 2\frac{\unicode[STIX]{x2202}\overline{f}}{\unicode[STIX]{x2202}z}=\left(1+\frac{\unicode[STIX]{x1D708}}{\unicode[STIX]{x1D708}_{0}}\right)(u+\text{i}v).\end{eqnarray}$$

Division of these two equations leads to

(3.5) $$\begin{eqnarray}\frac{\unicode[STIX]{x2202}f}{\unicode[STIX]{x2202}\overline{z}}=B(\unicode[STIX]{x1D708})\frac{\unicode[STIX]{x2202}\overline{f}}{\unicode[STIX]{x2202}\overline{z}},\end{eqnarray}$$

where

(3.6) $$\begin{eqnarray}B(\unicode[STIX]{x1D708})=\frac{1-\unicode[STIX]{x1D708}/\unicode[STIX]{x1D708}_{0}}{1+\unicode[STIX]{x1D708}/\unicode[STIX]{x1D708}_{0}},\end{eqnarray}$$

which is a real-valued function of the density $\unicode[STIX]{x1D708}(z,\overline{z})$ . Clearly $B(\unicode[STIX]{x1D708})=0$ when the flow is incompressible, reducing $f(z,\overline{z})$ to an analytic function. The complex velocity field $\unicode[STIX]{x1D709}(z,\bar{z})\equiv u-\text{i}v$ can be written either in terms of the complex potential as

(3.7) $$\begin{eqnarray}\unicode[STIX]{x1D709}(z,\bar{z})=u-\text{i}v=\frac{\unicode[STIX]{x2202}\unicode[STIX]{x1D719}}{\unicode[STIX]{x2202}x}-\text{i}\frac{\unicode[STIX]{x2202}\unicode[STIX]{x1D719}}{\unicode[STIX]{x2202}y}=\frac{\unicode[STIX]{x2202}}{\unicode[STIX]{x2202}z}(f+\overline{f})\end{eqnarray}$$

or, on use of (3.5), in terms of the complex potential and fluid density as

(3.8) $$\begin{eqnarray}\unicode[STIX]{x1D709}(z,\bar{z})={\displaystyle \frac{\unicode[STIX]{x2202}f}{\unicode[STIX]{x2202}z}}(1+B(\unicode[STIX]{x1D708})).\end{eqnarray}$$

We consider the fluid to be in isentropic flow, meaning that the pressure and density are related by $p=k\unicode[STIX]{x1D708}^{\unicode[STIX]{x1D6FE}}$ , where $k$ and $\unicode[STIX]{x1D6FE}>1$ are constants determined by the thermodynamical properties of the fluid (Jacob Reference Jacob1959; Pai Reference Pai1959; von Mises Reference von Mises2004). For steady flow of an inviscid barotropic fluid in two dimensions, a Bernoulli equation can be established (Saffman Reference Saffman1992):

(3.9) $$\begin{eqnarray}{\displaystyle \frac{|\unicode[STIX]{x1D709}|^{2}}{2}}+\int ^{\unicode[STIX]{x1D708}}\frac{\text{d}p}{\text{d}\tilde{\unicode[STIX]{x1D708}}}\frac{\text{d}\tilde{\unicode[STIX]{x1D708}}}{\tilde{\unicode[STIX]{x1D708}}}=\text{const.},\end{eqnarray}$$

where $\tilde{\unicode[STIX]{x1D708}}$ is a dummy integration variable. On substitution of the assumed pressure–density relationship into the Bernoulli equation (3.9), we can eliminate the pressure to obtain an equation relating the velocity and the density of the fluid:

(3.10) $$\begin{eqnarray}{\displaystyle \frac{|\unicode[STIX]{x1D709}|^{2}}{2}}+{\displaystyle \frac{k\unicode[STIX]{x1D6FE}\unicode[STIX]{x1D708}^{\unicode[STIX]{x1D6FE}-1}}{\unicode[STIX]{x1D6FE}-1}}={\displaystyle \frac{k\unicode[STIX]{x1D6FE}\unicode[STIX]{x1D708}_{s}^{\unicode[STIX]{x1D6FE}-1}}{\unicode[STIX]{x1D6FE}-1}},\end{eqnarray}$$

where $\unicode[STIX]{x1D708}_{s}$ is the (constant) density at the stagnation point in the flow. Since $\unicode[STIX]{x1D708}(z,\overline{z})>0$ , we will have a maximum value $|\unicode[STIX]{x1D709}|_{max}$ for the speed:

(3.11) $$\begin{eqnarray}|\unicode[STIX]{x1D709}|^{2}<|\unicode[STIX]{x1D709}|_{max}^{2}={\displaystyle \frac{2k\unicode[STIX]{x1D6FE}\unicode[STIX]{x1D708}_{s}^{\unicode[STIX]{x1D6FE}-1}}{\unicode[STIX]{x1D6FE}-1}}={\displaystyle \frac{2c_{s}^{2}}{\unicode[STIX]{x1D6FE}-1}},\end{eqnarray}$$

where $c_{s}$ is the speed of sound at the stagnation point; the speed of sound is defined by

(3.12) $$\begin{eqnarray}c^{2}\equiv \frac{\text{d}p}{\text{d}\unicode[STIX]{x1D708}}=k\unicode[STIX]{x1D6FE}\unicode[STIX]{x1D708}^{\unicode[STIX]{x1D6FE}-1}.\end{eqnarray}$$

The presence of an upper limit on the speed of fluid flow immediately rules out point vortices as solutions of the compressible flow equations; this is one reason for employing the hollow vortex model since it is a desingularization of the point vortex that is particularly convenient for compressible flow analysis. Returning to the Bernoulli equation (3.10), in terms of $c_{s}$ , we find

(3.13) $$\begin{eqnarray}\left[{\displaystyle \frac{\unicode[STIX]{x1D6FE}-1}{2c_{s}^{2}}}\right]|\unicode[STIX]{x1D709}|^{2}=1-\left[{\displaystyle \frac{\unicode[STIX]{x1D708}}{\unicode[STIX]{x1D708}_{s}}}\right]^{\unicode[STIX]{x1D6FE}-1}.\end{eqnarray}$$

We see from this equation that the density has a maximum value, namely $\unicode[STIX]{x1D708}_{s}$ : $\unicode[STIX]{x1D708}(z,\overline{z})<\unicode[STIX]{x1D708}_{s}$ .

We now have a closed system of equations governing the steady, two-dimensional, compressible and irrotational flow of an inviscid isentropic fluid: equation (3.5), which is complex-valued, and the Bernoulli equation (3.10) or (3.13), which is real. These two equations are to be solved for the complex potential and the density field.

4 Small-Mach-number perturbation: the Rayleigh–Jansen expansion

Let $M$ be the Mach number, defined by $M=V_{0}/c_{s}$ , where $V_{0}$ is the fluid speed at some suitably chosen point in the flow. In the general theory of Rayleigh–Jansen expansions, one considers flows that are weakly compressible so that $M\ll 1$ (Jacob Reference Jacob1959; Pai Reference Pai1959). Rayleigh–Jansen expansions for the complex potential and the velocity field take the form

(4.1a,b ) $$\begin{eqnarray}f(z,\overline{z})=f_{0}(z)+M^{2}f_{1}(z,\overline{z})+\cdots \,,\quad \unicode[STIX]{x1D709}(z,\overline{z})=\unicode[STIX]{x1D709}_{0}(z)+M^{2}\unicode[STIX]{x1D709}_{1}(z,\overline{z})+\cdots \,,\end{eqnarray}$$

where we use dots to denote the higher-order terms in an expansion in powers of $M^{2}$ . The complex potential $f_{0}(z)$ and complex velocity $\unicode[STIX]{x1D709}_{0}(z)$ are those associated with some incompressible base-state flow of interest. From the Bernoulli equation (3.13) we find

(4.2) $$\begin{eqnarray}\displaystyle {\displaystyle \frac{\unicode[STIX]{x1D708}}{\unicode[STIX]{x1D708}_{s}}} & = & \displaystyle \left[1-\left({\displaystyle \frac{\unicode[STIX]{x1D6FE}-1}{2}}\right){\displaystyle \frac{|\unicode[STIX]{x1D709}|^{2}}{c_{s}^{2}}}\right]^{(1/\unicode[STIX]{x1D6FE}-1)}=\left[1-\left({\displaystyle \frac{\unicode[STIX]{x1D6FE}-1}{2}}\right)\,{\displaystyle \frac{|\unicode[STIX]{x1D709}|^{2}}{V_{0}^{2}}}\,M^{2}\right]^{(1/\unicode[STIX]{x1D6FE}-1)}\nonumber\\ \displaystyle & = & \displaystyle 1-{\displaystyle \frac{1}{2}}\,{\displaystyle \frac{|\unicode[STIX]{x1D709}_{0}|^{2}}{V_{0}^{2}}}\,M^{2}+\cdots \,.\end{eqnarray}$$

To find the Rayleigh–Jansen expansion of (3.5), consider the function $B(\unicode[STIX]{x1D708})$ defined in (3.6). Without loss of generality, we set $\unicode[STIX]{x1D708}_{0}=\unicode[STIX]{x1D708}_{s}$ , leading to

(4.3a,b ) $$\begin{eqnarray}1-{\displaystyle \frac{\unicode[STIX]{x1D708}}{\unicode[STIX]{x1D708}_{s}}}={\displaystyle \frac{1}{2}}\,{\displaystyle \frac{|\unicode[STIX]{x1D709}_{0}|^{2}}{V_{0}^{2}}}\,M^{2}+\cdots \,,\quad 1+{\displaystyle \frac{\unicode[STIX]{x1D708}}{\unicode[STIX]{x1D708}_{s}}}=2\left[1-{\displaystyle \frac{1}{4}}\,{\displaystyle \frac{|\unicode[STIX]{x1D709}_{0}|^{2}}{V_{0}^{2}}}\,M^{2}+\cdots \,\right],\end{eqnarray}$$

so that

(4.4) $$\begin{eqnarray}B(\unicode[STIX]{x1D708})={\displaystyle \frac{1}{4}}\,{\displaystyle \frac{|\unicode[STIX]{x1D709}_{0}|^{2}}{V_{0}^{2}}}\,M^{2}+\cdots \,.\end{eqnarray}$$

Substituting the Rayleigh–Jansen expansion (4.1) for the complex potential into (3.5) gives

(4.5) $$\begin{eqnarray}\frac{\unicode[STIX]{x2202}f_{0}}{\unicode[STIX]{x2202}\overline{z}}+M^{2}\frac{\unicode[STIX]{x2202}f_{1}}{\unicode[STIX]{x2202}\overline{z}}+\cdots =B(\unicode[STIX]{x1D708})\frac{\unicode[STIX]{x2202}\overline{f}_{0}}{\unicode[STIX]{x2202}\overline{z}}+M^{2}B(\unicode[STIX]{x1D708})\frac{\unicode[STIX]{x2202}\overline{f}_{1}}{\unicode[STIX]{x2202}\overline{z}}+\cdots \,.\end{eqnarray}$$

On use of (4.4), and on equating coefficients of the powers of $M^{2}$ , we find

(4.6a,b ) $$\begin{eqnarray}\frac{\unicode[STIX]{x2202}f_{0}}{\unicode[STIX]{x2202}\overline{z}}=0,\quad \frac{\unicode[STIX]{x2202}f_{1}}{\unicode[STIX]{x2202}\overline{z}}={\displaystyle \frac{1}{4}}\,{\displaystyle \frac{|\unicode[STIX]{x1D709}_{0}|^{2}}{V_{0}^{2}}}\frac{\unicode[STIX]{x2202}\overline{f}_{0}}{\unicode[STIX]{x2202}\overline{z}}.\end{eqnarray}$$

The first equation reminds us that the incompressible complex potential is an analytic function; the second equation gives us a relation between the first-order complex potential and the incompressible solution. We can integrate the latter equation with respect to $\overline{z}$ :

(4.7) $$\begin{eqnarray}f_{1}(z,\overline{z})={\displaystyle \frac{1}{4V_{0}^{2}}}\unicode[STIX]{x1D709}_{0}(z)\overline{I(z)}+g(z),\end{eqnarray}$$

where

(4.8) $$\begin{eqnarray}I(z)=\int ^{z}\unicode[STIX]{x1D709}_{0}(\tilde{z})^{2}\,\text{d}\tilde{z},\end{eqnarray}$$

and where $g(z)$ has to be found. We refer to (4.7) as the Imai–Lamla formula: it gives an explicit formula, up to an unknown function $g(z)$ , for the first-order correction to the complex potential in terms of the leading-order incompressible solution.

To find the Rayleigh–Jansen expansion for the velocity, we substitute the Rayleigh–Jansen expansion for the complex potential (4.1) and (4.4) into (3.7):

(4.9) $$\begin{eqnarray}\unicode[STIX]{x1D709}(z,\overline{z})=\frac{\text{d}f_{0}}{\text{d}z}+M^{2}\left[{\displaystyle \frac{1}{4V_{0}^{2}}}\left(\unicode[STIX]{x1D709}_{0}(z)\right)^{2}\overline{\unicode[STIX]{x1D709}_{0}(z)}+\frac{\unicode[STIX]{x2202}f_{1}}{\unicode[STIX]{x2202}z}\right]+\cdots \,,\end{eqnarray}$$

where we have used the fact, following immediately from (4.8), that $\text{d}I(z)/\text{d}z=\unicode[STIX]{x1D709}_{0}^{2}(z)$ . We recognize immediately, on comparison of (4.1) and (4.9), that

(4.10) $$\begin{eqnarray}\unicode[STIX]{x1D709}_{1}(z,\overline{z})={\displaystyle \frac{1}{4V_{0}^{2}}}\left(\unicode[STIX]{x1D709}_{0}(z)\right)^{2}\overline{\unicode[STIX]{x1D709}_{0}(z)}+\frac{\unicode[STIX]{x2202}f_{1}}{\unicode[STIX]{x2202}z}.\end{eqnarray}$$

It will be expedient to perform the Rayleigh–Jansen analysis about the incompressible hollow vortex solutions with all functions considered as functions of a subsidiary parametric $\unicode[STIX]{x1D701}$ -plane, where $z$ and $\unicode[STIX]{x1D701}$ are related by a conformal mapping, which we will denote by

(4.11) $$\begin{eqnarray}z=z_{0}(\unicode[STIX]{x1D701})+M^{2}z_{1}(\unicode[STIX]{x1D701})+\cdots \,,\end{eqnarray}$$

where, just as for the complex potential and complex velocity in (4.1), we seek the form of the compressible perturbation to the conformal mapping $z_{0}(\unicode[STIX]{x1D701})$ which is relevant to the incompressible solution. The first-order correction $z_{1}(\unicode[STIX]{x1D701})$ will encode the change in shape of the hollow vortices due to the effects of compressibility.

It is important to explain a notational convention adopted for the remainder of this paper. We continue to use the function names $f_{0},~f_{1},\unicode[STIX]{x1D709}_{0},\unicode[STIX]{x1D709}_{1}$ even though we now take them to be functions of the parametric $\unicode[STIX]{x1D701}$ variable rather than of $z$ . Strictly speaking, new function names should be introduced, but this abuse of notation should not cause confusion.

The Imai–Lamla integral (4.8), written as a function of $\unicode[STIX]{x1D701}$ , will be denoted by

(4.12) $$\begin{eqnarray}{\mathcal{I}}(\unicode[STIX]{x1D701})=\int ^{\unicode[STIX]{x1D701}}\frac{f_{0}^{\prime }(\tilde{\unicode[STIX]{x1D701}})^{2}}{z_{0}^{\prime }(\tilde{\unicode[STIX]{x1D701}})}\,\text{d}\tilde{\unicode[STIX]{x1D701}},\end{eqnarray}$$

where we will use the prime notation to denote derivatives of analytic functions with respect to their arguments. The first-order correction to the complex potential is then

(4.13) $$\begin{eqnarray}f_{1}=\frac{1}{4V_{0}^{2}}\left[\unicode[STIX]{x1D709}_{0}(\unicode[STIX]{x1D701})\overline{{\mathcal{I}}(\unicode[STIX]{x1D701})}\right]+G(\unicode[STIX]{x1D701}),\end{eqnarray}$$

where the function $G(\unicode[STIX]{x1D701})$ must be found. With this change of variables, it follows that

(4.14) $$\begin{eqnarray}\unicode[STIX]{x1D709}(\unicode[STIX]{x1D701},\overline{\unicode[STIX]{x1D701}})=\unicode[STIX]{x1D709}_{0}(\unicode[STIX]{x1D701})+M^{2}\left[\frac{1}{z_{0}^{\prime }(\unicode[STIX]{x1D701})}\frac{\unicode[STIX]{x2202}}{\unicode[STIX]{x2202}\unicode[STIX]{x1D701}}(f_{1}+\overline{f_{1}})-\unicode[STIX]{x1D709}_{0}(\unicode[STIX]{x1D701})\frac{z_{1}^{\prime }(\unicode[STIX]{x1D701})}{z_{0}^{\prime }(\unicode[STIX]{x1D701})}\right]+\cdots\end{eqnarray}$$

and, on comparison with (4.1),

(4.15) $$\begin{eqnarray}\unicode[STIX]{x1D709}_{1}(\unicode[STIX]{x1D701},\overline{\unicode[STIX]{x1D701}})=\unicode[STIX]{x1D709}_{0}(\unicode[STIX]{x1D701})\left[\,\frac{1}{f_{0}^{\prime }(\unicode[STIX]{x1D701})}\frac{\unicode[STIX]{x2202}}{\unicode[STIX]{x2202}\unicode[STIX]{x1D701}}(f_{1}+\overline{f_{1}})-\frac{z_{1}^{\prime }(\unicode[STIX]{x1D701})}{z_{0}^{\prime }(\unicode[STIX]{x1D701})}\right].\end{eqnarray}$$

As an instructive illustration of the new approach adopted here, supplementary material is available at https://doi.org/10.1017/jfm.2017.821, in which we use the Imai–Lamla method and the techniques of conformal mapping to rederive the Rayleigh–Jansen expansion about the single incompressible hollow vortex row found by Baker et al. (Reference Baker, Saffman and Sheffield1976). A different but equivalent analysis has been performed by Ardalan et al. (Reference Ardalan, Meiron and Pullin1995) using a hodograph formulation and Chaplygin’s equation. We check our results against the latter work and thus provide a conceptual check on the new approach. The analysis to follow is a natural extension, to the staggered hollow vortex street, of that given in the supplementary material for the single hollow vortex row.

5 Rayleigh–Jansen expansion: the staggered hollow vortex street

We now consider a Rayleigh–Jansen expansion about the incompressible solution of Crowdy & Green (Reference Crowdy and Green2011). We choose the velocity scale $V_{0}$ in the definition of the Mach number to be the speed of the incompressible hollow vortex street $U$ from § 2 (we assume that $U\neq 0$ , otherwise a different choice of Mach number would be more appropriate). The complex potential and complex velocity will be perturbed as in (4.1) and, for a fixed value of the street aspect ratio and area of the vortices, we expect the speed of the street to be altered to

(5.1) $$\begin{eqnarray}U+M^{2}\unicode[STIX]{x0394}U.\end{eqnarray}$$

The key objective of the analysis to follow is to determine $\unicode[STIX]{x0394}U$ .

5.1 Perturbation of the conformal mapping

Since the incompressible vortices are perturbed, we must assume that the conformally equivalent cut annulus to the compressible vortices is also perturbed to

(5.2a,b ) $$\begin{eqnarray}\unicode[STIX]{x1D70C}<|\unicode[STIX]{x1D701}|<1,\quad \unicode[STIX]{x1D70C}=\unicode[STIX]{x1D70C}_{0}+M^{2}\hat{\unicode[STIX]{x1D70C}}+\cdots \,,\end{eqnarray}$$

with the preimages of $\infty ^{+}$ and $\infty ^{-}$ becoming

(5.3a,b ) $$\begin{eqnarray}\unicode[STIX]{x1D6FC}=\unicode[STIX]{x1D6FC}_{0}+M^{2}\hat{\unicode[STIX]{x1D6FC}}+\cdots \,,\quad \unicode[STIX]{x1D6FD}=\unicode[STIX]{x1D6FD}_{0}+M^{2}\hat{\unicode[STIX]{x1D6FD}}+\cdots \,,\end{eqnarray}$$

for parameters $\hat{\unicode[STIX]{x1D6FC}}$ and $\hat{\unicode[STIX]{x1D6FD}}$ to be determined. In order that the perturbed mapping corresponds to an $L$ -periodic structure, we write it in the form

(5.4) $$\begin{eqnarray}z(\unicode[STIX]{x1D701})=-\frac{\text{i}L}{2\unicode[STIX]{x03C0}}\log \left[\frac{P(\unicode[STIX]{x1D701}/\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D70C}_{0})}{P(\unicode[STIX]{x1D701}/\unicode[STIX]{x1D6FD},\unicode[STIX]{x1D70C}_{0})}\right]+\tilde{z}_{0}(\unicode[STIX]{x1D701})+M^{2}\tilde{z}_{1}(\unicode[STIX]{x1D701})+\cdots \,,\end{eqnarray}$$

where we define $\tilde{z}_{0}(\unicode[STIX]{x1D701})$ via the relation

(5.5) $$\begin{eqnarray}z_{0}(\unicode[STIX]{x1D701})=-\frac{\text{i}L}{2\unicode[STIX]{x03C0}}\log \left[\frac{P(\unicode[STIX]{x1D701}/\unicode[STIX]{x1D6FC}_{0},\unicode[STIX]{x1D70C}_{0})}{P(\unicode[STIX]{x1D701}/\unicode[STIX]{x1D6FD}_{0},\unicode[STIX]{x1D70C}_{0})}\right]+\tilde{z}_{0}(\unicode[STIX]{x1D701}).\end{eqnarray}$$

The quantity $\tilde{z}_{1}(\unicode[STIX]{x1D701})$ is analytic and single-valued in the perturbed cut annulus (5.2). On linearizing in $M^{2}$ :

(5.6) $$\begin{eqnarray}P(\unicode[STIX]{x1D701}/\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D70C}_{0})=P(\unicode[STIX]{x1D701}/\unicode[STIX]{x1D6FC}_{0},\unicode[STIX]{x1D70C}_{0})\left[1-M^{2}\frac{\hat{\unicode[STIX]{x1D6FC}}}{\unicode[STIX]{x1D6FC}_{0}}K(\unicode[STIX]{x1D701}/\unicode[STIX]{x1D6FC}_{0},\unicode[STIX]{x1D70C}_{0})\right].\end{eqnarray}$$

Therefore

(5.7) $$\begin{eqnarray}\displaystyle -\frac{\text{i}L}{2\unicode[STIX]{x03C0}}\log \left[\frac{P(\unicode[STIX]{x1D701}/\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D70C}_{0})}{P(\unicode[STIX]{x1D701}/\unicode[STIX]{x1D6FD},\unicode[STIX]{x1D70C}_{0})}\right] & = & \displaystyle -\frac{\text{i}L}{2\unicode[STIX]{x03C0}}\log \left[\frac{P(\unicode[STIX]{x1D701}/\unicode[STIX]{x1D6FC}_{0},\unicode[STIX]{x1D70C}_{0})}{P(\unicode[STIX]{x1D701}/\unicode[STIX]{x1D6FD}_{0},\unicode[STIX]{x1D70C}_{0})}\right]\nonumber\\ \displaystyle & & \displaystyle +\,M^{2}\left(\frac{\text{i}L}{2\unicode[STIX]{x03C0}}\right)\left[\frac{\hat{\unicode[STIX]{x1D6FC}}}{\unicode[STIX]{x1D6FC}_{0}}K(\unicode[STIX]{x1D701}/\unicode[STIX]{x1D6FC}_{0},\unicode[STIX]{x1D70C}_{0})-\frac{\hat{\unicode[STIX]{x1D6FD}}}{\unicode[STIX]{x1D6FD}_{0}}K(\unicode[STIX]{x1D701}/\unicode[STIX]{x1D6FD}_{0},\unicode[STIX]{x1D70C}_{0})\right]+\cdots \nonumber\\ \displaystyle & & \displaystyle\end{eqnarray}$$

implying that

(5.8) $$\begin{eqnarray}z=z_{0}(\unicode[STIX]{x1D701})+M^{2}\left(\frac{\text{i}L}{2\unicode[STIX]{x03C0}}\right)\left[\frac{\hat{\unicode[STIX]{x1D6FC}}}{\unicode[STIX]{x1D6FC}_{0}}K(\unicode[STIX]{x1D701}/\unicode[STIX]{x1D6FC}_{0},\unicode[STIX]{x1D70C}_{0})-\frac{\hat{\unicode[STIX]{x1D6FD}}}{\unicode[STIX]{x1D6FD}_{0}}K(\unicode[STIX]{x1D701}/\unicode[STIX]{x1D6FD}_{0},\unicode[STIX]{x1D70C}_{0})\right]+M^{2}\tilde{z}_{1}(\unicode[STIX]{x1D701})+\cdots \,.\end{eqnarray}$$

On comparison with

(5.9) $$\begin{eqnarray}z(\unicode[STIX]{x1D701})=z_{0}(\unicode[STIX]{x1D701})+M^{2}z_{1}(\unicode[STIX]{x1D701})+\cdots \,,\end{eqnarray}$$

we infer that

(5.10) $$\begin{eqnarray}z_{1}(\unicode[STIX]{x1D701})={\mathcal{Z}}_{s}(\unicode[STIX]{x1D701})+\tilde{z}_{1}(\unicode[STIX]{x1D701}),\end{eqnarray}$$

where

(5.11) $$\begin{eqnarray}{\mathcal{Z}}_{s}(\unicode[STIX]{x1D701})\equiv \left(\frac{\text{i}L}{2\unicode[STIX]{x03C0}}\right)\left[\frac{\hat{\unicode[STIX]{x1D6FC}}}{\unicode[STIX]{x1D6FC}_{0}}K(\unicode[STIX]{x1D701}/\unicode[STIX]{x1D6FC}_{0},\unicode[STIX]{x1D70C}_{0})-\frac{\hat{\unicode[STIX]{x1D6FD}}}{\unicode[STIX]{x1D6FD}_{0}}K(\unicode[STIX]{x1D701}/\unicode[STIX]{x1D6FD}_{0},\unicode[STIX]{x1D70C}_{0})\right].\end{eqnarray}$$

This first-order correction $z_{1}(\unicode[STIX]{x1D701})$ to the conformal mapping has simple poles at $\unicode[STIX]{x1D6FC}_{0}$ and  $\unicode[STIX]{x1D6FD}_{0}$ .

5.2 The Imai–Lamla integral

For the leading-order solution, we have assumed that the origin is at the centre of the period window of interest, and that the staggered vortices are rotations of each other by angle $\unicode[STIX]{x03C0}$ . It is natural to suppose that it will be possible to find a compressible staggered vortex street solution with this same symmetry.

We need to evaluate the integral ${\mathcal{I}}(\unicode[STIX]{x1D701})$ defined in (4.12). Remarkably, it is possible to do this in closed form. Appendix C explains how to derive the following expression:

(5.12) $$\begin{eqnarray}{\mathcal{I}}(\unicode[STIX]{x1D701})=-\frac{\text{i}LU^{2}}{2\unicode[STIX]{x03C0}}\left[\log \left[\frac{P(\unicode[STIX]{x1D701}/\unicode[STIX]{x1D6FC}_{0},\unicode[STIX]{x1D70C}_{0})}{P(\unicode[STIX]{x1D701}/\unicode[STIX]{x1D6FD}_{0},\unicode[STIX]{x1D70C}_{0})}\right]-\frac{1}{\unicode[STIX]{x1D712}}\log \left[\frac{P(\unicode[STIX]{x1D701}\unicode[STIX]{x1D6FC}_{0},\unicode[STIX]{x1D70C}_{0})}{P(\unicode[STIX]{x1D701}\unicode[STIX]{x1D6FD}_{0},\unicode[STIX]{x1D70C}_{0})}\right]\right].\end{eqnarray}$$

Now $f_{1}$ takes the form (4.13), where $G(\unicode[STIX]{x1D701})$ is to be determined. To ensure that the velocity field is $L$ -periodic, we can choose

(5.13) $$\begin{eqnarray}G(\unicode[STIX]{x1D701})=-\frac{1}{4U^{2}}\unicode[STIX]{x1D709}_{0}(\unicode[STIX]{x1D701}){\mathcal{I}}(\unicode[STIX]{x1D701})+{\mathcal{G}}(\unicode[STIX]{x1D701}),\end{eqnarray}$$

where ${\mathcal{G}}(\unicode[STIX]{x1D701})$ is single-valued in the cut annulus (but it may have isolated singularities). Note from (5.12) that

(5.14) $$\begin{eqnarray}{\mathcal{I}}(\unicode[STIX]{x1D701})=U^{2}z_{0}(\unicode[STIX]{x1D701})+\frac{\text{i}LU^{2}}{2\unicode[STIX]{x03C0}}\left[\frac{1}{\unicode[STIX]{x1D712}}-\unicode[STIX]{x1D712}\right]\log \left[\frac{P(\unicode[STIX]{x1D701}\unicode[STIX]{x1D6FC}_{0},\unicode[STIX]{x1D70C}_{0})}{P(\unicode[STIX]{x1D701}\unicode[STIX]{x1D6FD}_{0},\unicode[STIX]{x1D70C}_{0})}\right],\end{eqnarray}$$

so that, since $\unicode[STIX]{x1D709}_{0}\rightarrow -U$ as $z\rightarrow \infty$ ,

(5.15) $$\begin{eqnarray}-\frac{1}{4U^{2}}\unicode[STIX]{x1D709}_{0}(\unicode[STIX]{x1D701}){\mathcal{I}}(\unicode[STIX]{x1D701})\sim \frac{U}{4}z,\quad \text{as }z\rightarrow \infty .\end{eqnarray}$$

Hence, from (4.15), any first-order correction to the speed at infinity will come only from ${\mathcal{G}}(\unicode[STIX]{x1D701})$ . With the choice (5.13),

(5.16) $$\begin{eqnarray}f_{1}=\frac{1}{4U^{2}}\unicode[STIX]{x1D709}_{0}(\unicode[STIX]{x1D701})\left\{\overline{{\mathcal{I}}(\unicode[STIX]{x1D701})}-{\mathcal{I}}(\unicode[STIX]{x1D701})\right\}+{\mathcal{G}}(\unicode[STIX]{x1D701}),\end{eqnarray}$$

where

(5.17) $$\begin{eqnarray}\overline{{\mathcal{I}}(\unicode[STIX]{x1D701})}-{\mathcal{I}}(\unicode[STIX]{x1D701})=\frac{\text{i}LU^{2}}{2\unicode[STIX]{x03C0}}\left[\log \left|\frac{P(\unicode[STIX]{x1D701}/\unicode[STIX]{x1D6FC}_{0},\unicode[STIX]{x1D70C}_{0})}{P(\unicode[STIX]{x1D701}/\unicode[STIX]{x1D6FD}_{0},\unicode[STIX]{x1D70C}_{0})}\right|^{2}-\frac{1}{\unicode[STIX]{x1D712}}\log \left|\frac{P(\unicode[STIX]{x1D701}\unicode[STIX]{x1D6FC}_{0},\unicode[STIX]{x1D70C}_{0})}{P(\unicode[STIX]{x1D701}\unicode[STIX]{x1D6FD}_{0},\unicode[STIX]{x1D70C}_{0})}\right|^{2}\right],\end{eqnarray}$$

whence it can be checked that the associated velocity field is $L$ -periodic. For the perturbed problem, we expect, in the far field, the effects of compressibility to have decayed, and the flow to be irrotational, except for the change in speed of the street. Hence as $z\rightarrow \infty$ ,

(5.18) $$\begin{eqnarray}f_{0}+M^{2}f_{1}\rightarrow -(U+M^{2}\unicode[STIX]{x0394}U)z=-(U+M^{2}\unicode[STIX]{x0394}U)(z_{0}+M^{2}z_{1})+\cdots \,,\end{eqnarray}$$

where we have used (5.1). But since $f_{1}\sim {\mathcal{G}}(\unicode[STIX]{x1D701})$ as $z\rightarrow \infty$ , then we identify, at $O(M^{2})$ ,

(5.19) $$\begin{eqnarray}{\mathcal{G}}\sim -\unicode[STIX]{x0394}Uz_{0}-Uz_{1},\quad \text{as }z\rightarrow \infty .\end{eqnarray}$$

Motivated by this we make the choice

(5.20) $$\begin{eqnarray}{\mathcal{G}}(\unicode[STIX]{x1D701})=\unicode[STIX]{x1D709}_{0}{\mathcal{Z}}_{s}+\frac{\unicode[STIX]{x0394}U}{U}\left[f_{0}+\frac{\text{i}\unicode[STIX]{x1D6E4}}{2\unicode[STIX]{x03C0}}\log \unicode[STIX]{x1D701}\right]+\tilde{{\mathcal{G}}}(\unicode[STIX]{x1D701}),\end{eqnarray}$$

where $\tilde{{\mathcal{G}}}(\unicode[STIX]{x1D701})$ is analytic and single-valued in the annulus, which, given that $f_{0}\rightarrow -Uz_{0}$ , $z_{1}={\mathcal{Z}}_{s}+\tilde{z}_{1}$ and $\unicode[STIX]{x1D709}_{0}\rightarrow -U$ , has precisely the expected far-field asymptotics (5.19). The term in square brackets in (5.20) warrants some explanation. It includes a logarithmic term that negates a similar term appearing in $f_{0}$ and hence contributes no additional circulation around either of the vortices. This is necessary because we want the circulation of the compressible vortices to be the same as the incompressible solution. From (4.14) the $O(M^{2})$ change in circulation around the vortex that is the image of $|\unicode[STIX]{x1D701}|=1$ due to the perturbation from the first term on the right-hand side of (5.16) is

(5.21) $$\begin{eqnarray}\text{Re}\left[\oint _{|\unicode[STIX]{x1D701}|=1}(\unicode[STIX]{x1D709}_{1}z_{0}^{\prime }(\unicode[STIX]{x1D701})+\unicode[STIX]{x1D709}_{0}z_{1}^{\prime }(\unicode[STIX]{x1D701}))\,\text{d}\unicode[STIX]{x1D701}\right]=\text{Re}\left[\oint _{|\unicode[STIX]{x1D701}|=1}\text{d}\unicode[STIX]{x1D709}_{0}\,(\overline{{\mathcal{I}}(\unicode[STIX]{x1D701})}-{\mathcal{I}}(\unicode[STIX]{x1D701}))+(\overline{\unicode[STIX]{x1D709}_{0}}-\unicode[STIX]{x1D709}_{0})\,\text{d}{\mathcal{I}}\right]=0,\end{eqnarray}$$

where the last equality follows because the integral can be shown to be an integral of a total derivative of a single-valued function around the closed curve $|\unicode[STIX]{x1D701}|=1$ .

5.3 Streamline condition for ${\mathcal{G}}(\unicode[STIX]{x1D701})$

The leading-order kinematic boundary condition that each hollow vortex boundary is a streamline is

(5.22) $$\begin{eqnarray}\text{Im}[f_{0}]=\left\{\begin{array}{@{}ll@{}}0, & \text{ on }|\unicode[STIX]{x1D701}|=1,\\ c_{0}, & \text{ on }|\unicode[STIX]{x1D701}|=\unicode[STIX]{x1D70C}_{0},\end{array}\right.\end{eqnarray}$$

where

(5.23) $$\begin{eqnarray}c_{0}=\frac{LU}{2\unicode[STIX]{x03C0}}\log \biggl|\frac{\unicode[STIX]{x1D6FC}_{0}}{\unicode[STIX]{x1D6FD}_{0}}\biggr|-\frac{\unicode[STIX]{x1D6E4}}{2\unicode[STIX]{x03C0}}\log \unicode[STIX]{x1D70C}_{0}\end{eqnarray}$$

is a constant. For the compressible vortices, this condition is perturbed to

(5.24) $$\begin{eqnarray}\text{Im}[f_{0}+M^{2}f_{1}+\cdots \,]=\left\{\begin{array}{@{}ll@{}}0, & \text{ on }|\unicode[STIX]{x1D701}|=1,\\ c_{0}+M^{2}c_{1}, & \text{ on }|\unicode[STIX]{x1D701}|=\unicode[STIX]{x1D70C}_{0}+M^{2}\hat{\unicode[STIX]{x1D70C}},\end{array}\right.\end{eqnarray}$$

where the value of $c_{1}$ is to be determined. On $|\unicode[STIX]{x1D701}|=\unicode[STIX]{x1D70C}=\unicode[STIX]{x1D70C}_{0}+M^{2}\hat{\unicode[STIX]{x1D70C}}$ ,

(5.25) $$\begin{eqnarray}f_{0}(\unicode[STIX]{x1D701})=f_{0}(\unicode[STIX]{x1D701})\biggl|_{|\unicode[STIX]{x1D701}|=\unicode[STIX]{x1D70C}_{0}}+~M^{2}\frac{\hat{\unicode[STIX]{x1D70C}}}{\unicode[STIX]{x1D70C}_{0}}\unicode[STIX]{x1D701}f_{0}^{\prime }(\unicode[STIX]{x1D701})\biggr|_{|\unicode[STIX]{x1D701}|=\unicode[STIX]{x1D70C}_{0}}+\cdots \,,\end{eqnarray}$$

which, on use of the leading-order boundary conditions (5.22), gives

(5.26) $$\begin{eqnarray}\text{Im}[f_{1}]=\left\{\begin{array}{@{}ll@{}}0, & \text{ on }|\unicode[STIX]{x1D701}|=1,\\ c_{1}-\displaystyle \frac{\hat{\unicode[STIX]{x1D70C}}}{\unicode[STIX]{x1D70C}_{0}}\text{Im}[\unicode[STIX]{x1D701}f_{0}^{\prime }(\unicode[STIX]{x1D701})], & \text{ on }|\unicode[STIX]{x1D701}|=\unicode[STIX]{x1D70C}_{0}.\end{array}\right.\end{eqnarray}$$

However, on substitution of (5.20) into (5.16) and the result into (5.26), we arrive at the modified Schwarz problem (Crowdy Reference Crowdy2008b ) in the concentric annulus $\unicode[STIX]{x1D70C}_{0}<|\unicode[STIX]{x1D701}|<1$ ,

(5.27) $$\begin{eqnarray}\text{Re}[-\text{i}\tilde{{\mathcal{G}}}(\unicode[STIX]{x1D701})]=\left\{\begin{array}{@{}ll@{}}\displaystyle \frac{\unicode[STIX]{x0394}U}{U}g_{0}-\text{Im}[S(\unicode[STIX]{x1D701},\overline{\unicode[STIX]{x1D701}})]-\text{Im}[\unicode[STIX]{x1D709}_{0}{\mathcal{Z}}_{s}], & \text{ on }|\unicode[STIX]{x1D701}|=1,\\ c_{1}+\displaystyle \frac{\unicode[STIX]{x0394}U}{U}g_{1}-\displaystyle \frac{\hat{\unicode[STIX]{x1D70C}}}{\unicode[STIX]{x1D70C}_{0}}\text{Im}[\unicode[STIX]{x1D701}f_{0}^{\prime }(\unicode[STIX]{x1D701})]-\text{Im}[S(\unicode[STIX]{x1D701},\overline{\unicode[STIX]{x1D701}})]-\text{Im}[\unicode[STIX]{x1D709}_{0}{\mathcal{Z}}_{s}], & \text{ on }|\unicode[STIX]{x1D701}|=\unicode[STIX]{x1D70C}_{0},\end{array}\right.\end{eqnarray}$$

where we define

(5.28) $$\begin{eqnarray}S(\unicode[STIX]{x1D701},\overline{\unicode[STIX]{x1D701}})\equiv \frac{1}{4U^{2}}[\unicode[STIX]{x1D709}_{0}(\unicode[STIX]{x1D701})\{\overline{{\mathcal{I}}(\unicode[STIX]{x1D701})}-{\mathcal{I}}(\unicode[STIX]{x1D701})\}]\end{eqnarray}$$

and

(5.29) $$\begin{eqnarray}\text{Im}\left[f_{0}+\frac{\text{i}\unicode[STIX]{x1D6E4}}{2\unicode[STIX]{x03C0}}\log \unicode[STIX]{x1D701}\right]=\left\{\begin{array}{@{}ll@{}}0, & \text{ on }|\unicode[STIX]{x1D701}|=1,\\ g_{1}, & \text{ on }|\unicode[STIX]{x1D701}|=\unicode[STIX]{x1D70C}_{0},\end{array}\right.\end{eqnarray}$$

where

(5.30) $$\begin{eqnarray}g_{1}=\frac{LU}{2\unicode[STIX]{x03C0}}\log \biggl|\frac{\unicode[STIX]{x1D6FC}_{0}}{\unicode[STIX]{x1D6FD}_{0}}\biggr|.\end{eqnarray}$$

It is convenient to use the linearity of the problem and write the solution in the form

(5.31) $$\begin{eqnarray}\tilde{{\mathcal{G}}}(\unicode[STIX]{x1D701})=V_{1}(\unicode[STIX]{x1D701})+\frac{\hat{\unicode[STIX]{x1D6FC}}}{\unicode[STIX]{x1D6FC}_{0}}V_{2}(\unicode[STIX]{x1D701})+\frac{\hat{\unicode[STIX]{x1D6FD}}}{\unicode[STIX]{x1D6FD}_{0}}V_{3}(\unicode[STIX]{x1D701})+\frac{\unicode[STIX]{x0394}U}{U}V_{4}(\unicode[STIX]{x1D701})+\frac{\hat{\unicode[STIX]{x1D70C}}}{\unicode[STIX]{x1D70C}_{0}}V_{5}(\unicode[STIX]{x1D701}).\end{eqnarray}$$

Here $\{V_{j}(\unicode[STIX]{x1D701})\mid j=1,\ldots ,5\}$ are single-valued functions in the annulus $\unicode[STIX]{x1D70C}_{0}<|\unicode[STIX]{x1D701}|<1$ and are defined, up to an additive real constant, by the modified Schwarz problems in the concentric annulus $\unicode[STIX]{x1D70C}_{0}<|\unicode[STIX]{x1D701}|<1$ :

(5.32) $$\begin{eqnarray}\left.\begin{array}{@{}c@{}}\begin{array}{@{}ll@{}}\text{Re}[-\text{i}V_{1}(\unicode[STIX]{x1D701})] & =\left\{\begin{array}{@{}ll@{}}-\text{Im}[S(\unicode[STIX]{x1D701},\overline{\unicode[STIX]{x1D701}})], & \text{ on }|\unicode[STIX]{x1D701}|=1,\\ -\text{Im}[S(\unicode[STIX]{x1D701},\overline{\unicode[STIX]{x1D701}})]+v_{1}, & \text{ on }|\unicode[STIX]{x1D701}|=\unicode[STIX]{x1D70C}_{0},\end{array}\right.\\ \text{Re}[-\text{i}V_{2}(\unicode[STIX]{x1D701})] & =\left\{\begin{array}{@{}ll@{}}-\displaystyle \text{Im}\left[\frac{\text{i}L}{2\unicode[STIX]{x03C0}}\unicode[STIX]{x1D709}_{0}(\unicode[STIX]{x1D701})K(\unicode[STIX]{x1D701}/\unicode[STIX]{x1D6FC}_{0},\unicode[STIX]{x1D70C}_{0})\right], & \text{ on }|\unicode[STIX]{x1D701}|=1,\\ -\displaystyle \text{Im}\left[\frac{\text{i}L}{2\unicode[STIX]{x03C0}}\unicode[STIX]{x1D709}_{0}(\unicode[STIX]{x1D701})K(\unicode[STIX]{x1D701}/\unicode[STIX]{x1D6FC}_{0},\unicode[STIX]{x1D70C}_{0})\right]+v_{2}, & \text{ on }|\unicode[STIX]{x1D701}|=\unicode[STIX]{x1D70C}_{0},\end{array}\right.\\ \text{Re}[-\text{i}V_{3}(\unicode[STIX]{x1D701})] & =\left\{\begin{array}{@{}ll@{}}\displaystyle \text{Im}\left[\frac{\text{i}L}{2\unicode[STIX]{x03C0}}\unicode[STIX]{x1D709}_{0}(\unicode[STIX]{x1D701})K(\unicode[STIX]{x1D701}/\unicode[STIX]{x1D6FD}_{0},\unicode[STIX]{x1D70C}_{0})\right], & \text{ on }|\unicode[STIX]{x1D701}|=1,\\ \displaystyle \text{Im}\left[\frac{\text{i}L}{2\unicode[STIX]{x03C0}}\unicode[STIX]{x1D709}_{0}(\unicode[STIX]{x1D701})K(\unicode[STIX]{x1D701}/\unicode[STIX]{x1D6FD}_{0},\unicode[STIX]{x1D70C}_{0})\right]+v_{3}, & \text{ on }|\unicode[STIX]{x1D701}|=\unicode[STIX]{x1D70C}_{0},\end{array}\right.\\ \text{Re}[-\text{i}V_{4}(\unicode[STIX]{x1D701})] & =\left\{\begin{array}{@{}ll@{}}0, & \text{ on }|\unicode[STIX]{x1D701}|=1,\\ g_{1}+v_{4}, & \text{ on }|\unicode[STIX]{x1D701}|=\unicode[STIX]{x1D70C}_{0},\end{array}\right.\\ \text{Re}[-\text{i}V_{5}(\unicode[STIX]{x1D701})] & =\left\{\begin{array}{@{}ll@{}}0, & \text{ on }|\unicode[STIX]{x1D701}|=1,\\ -\text{Im}[\unicode[STIX]{x1D701}f_{0}^{\prime }(\unicode[STIX]{x1D701})]+v_{5}, & \text{ on }|\unicode[STIX]{x1D701}|=\unicode[STIX]{x1D70C}_{0}.\end{array}\right.\end{array}\end{array}\right\}\end{eqnarray}$$

The real constants $\{v_{j}\mid j=1,\ldots ,5\}$ are determined by solvability conditions for each modified Schwarz problem. Appendix D contains a discussion of what is meant by the designation modified Schwarz problem in this case, and the solvability condition just mentioned; it also discusses various solution schemes. In this case, the solvability condition for $\tilde{G}(\unicode[STIX]{x1D701})$ is that

(5.33) $$\begin{eqnarray}c_{1}=v_{1}+\frac{\hat{\unicode[STIX]{x1D6FC}}}{\unicode[STIX]{x1D6FC}_{0}}v_{2}+\frac{\hat{\unicode[STIX]{x1D6FD}}}{\unicode[STIX]{x1D6FD}_{0}}v_{3}+\frac{\unicode[STIX]{x0394}U}{U}v_{4}+\frac{\hat{\unicode[STIX]{x1D70C}}}{\unicode[STIX]{x1D70C}_{0}}v_{5},\end{eqnarray}$$

which merely determines $c_{1}$ and does not constitute any constraint on the as yet unknown parameters $\hat{\unicode[STIX]{x1D6FC}},\hat{\unicode[STIX]{x1D6FD}},\unicode[STIX]{x0394}U/U$ and $\hat{\unicode[STIX]{x1D70C}}$ .

5.4 Bernoulli condition

It follows from (5.16) that

(5.34) $$\begin{eqnarray}\frac{\unicode[STIX]{x2202}}{\unicode[STIX]{x2202}\unicode[STIX]{x1D701}}(f_{1}+\overline{f_{1}})=R(\unicode[STIX]{x1D701},\overline{\unicode[STIX]{x1D701}})+{\mathcal{G}}^{\prime }(\unicode[STIX]{x1D701}),\end{eqnarray}$$

where

(5.35) $$\begin{eqnarray}R(\unicode[STIX]{x1D701},\overline{\unicode[STIX]{x1D701}})\equiv \frac{1}{4U^{2}}\left[\unicode[STIX]{x1D709}_{0}^{\prime }(\unicode[STIX]{x1D701})\left\{\overline{{\mathcal{I}}(\unicode[STIX]{x1D701})}-{\mathcal{I}}(\unicode[STIX]{x1D701})\right\}+\left(\overline{\unicode[STIX]{x1D709}_{0}(\unicode[STIX]{x1D701})}-\unicode[STIX]{x1D709}_{0}(\unicode[STIX]{x1D701})\right){\mathcal{I}}^{\prime }(\unicode[STIX]{x1D701})\right].\end{eqnarray}$$

Use of this in (4.15) then implies

(5.36) $$\begin{eqnarray}\unicode[STIX]{x1D709}_{1}(\unicode[STIX]{x1D701},\overline{\unicode[STIX]{x1D701}})=\unicode[STIX]{x1D709}_{0}(\unicode[STIX]{x1D701})\left[\,\frac{1}{f_{0}^{\prime }(\unicode[STIX]{x1D701})}[R(\unicode[STIX]{x1D701},\overline{\unicode[STIX]{x1D701}})+{\mathcal{G}}^{\prime }(\unicode[STIX]{x1D701})]-\frac{z_{1}^{\prime }(\unicode[STIX]{x1D701})}{z_{0}^{\prime }(\unicode[STIX]{x1D701})}\right].\end{eqnarray}$$

Now from (5.20),

(5.37) $$\begin{eqnarray}{\mathcal{G}}^{\prime }(\unicode[STIX]{x1D701})=\unicode[STIX]{x1D709}_{0}^{\prime }{\mathcal{Z}}_{s}+\unicode[STIX]{x1D709}_{0}{\mathcal{Z}}_{s}^{\prime }+\unicode[STIX]{x1D6FE}\left[f_{0}^{\prime }+\frac{\text{i}\unicode[STIX]{x1D6E4}}{2\unicode[STIX]{x03C0}\unicode[STIX]{x1D701}}\right]+\tilde{{\mathcal{G}}}^{\prime }(\unicode[STIX]{x1D701}).\end{eqnarray}$$

On substitution into (5.36) this becomes

(5.38) $$\begin{eqnarray}\unicode[STIX]{x1D709}_{1}=\unicode[STIX]{x1D709}_{0}\left[\frac{R(\unicode[STIX]{x1D701},\overline{\unicode[STIX]{x1D701}})}{f_{0}^{\prime }(\unicode[STIX]{x1D701})}+\frac{\unicode[STIX]{x1D709}_{0}^{\prime }(\unicode[STIX]{x1D701})}{f_{0}^{\prime }(\unicode[STIX]{x1D701})}{\mathcal{Z}}_{s}(\unicode[STIX]{x1D701})+\unicode[STIX]{x1D6FE}\left[1+\frac{\text{i}\unicode[STIX]{x1D6E4}}{2\unicode[STIX]{x03C0}\unicode[STIX]{x1D701}f_{0}^{\prime }(\unicode[STIX]{x1D701})}\right]+\frac{\tilde{{\mathcal{G}}}^{\prime }(\unicode[STIX]{x1D701})}{f_{0}^{\prime }(\unicode[STIX]{x1D701})}-\frac{\tilde{z}_{1}^{\prime }(\unicode[STIX]{x1D701})}{z_{0}^{\prime }(\unicode[STIX]{x1D701})}\right],\end{eqnarray}$$

where we have also used (5.10) and a term involving ${\mathcal{Z}}_{s}^{\prime }$ cancels out.

The base-state boundary conditions take the form

(5.39) $$\begin{eqnarray}\unicode[STIX]{x1D709}_{0}\overline{\unicode[STIX]{x1D709}_{0}}=\left\{\begin{array}{@{}ll@{}}q_{0}^{2}, & \text{ on }|\unicode[STIX]{x1D701}|=1,\\ q_{0}^{2}, & \text{ on }|\unicode[STIX]{x1D701}|=\unicode[STIX]{x1D70C}_{0},\end{array}\right.\end{eqnarray}$$

for some constant $q_{0}$ . The perturbed form, assuming we seek a solution where both vortices have the same boundary velocity perturbation, is

(5.40) $$\begin{eqnarray}|\unicode[STIX]{x1D709}|^{2}=\unicode[STIX]{x1D709}_{0}\overline{\unicode[STIX]{x1D709}_{0}}+2M^{2}\,\text{Re}[\unicode[STIX]{x1D709}_{1}\overline{\unicode[STIX]{x1D709}_{0}}]+\cdots =\left\{\begin{array}{@{}ll@{}}q_{0}^{2}+2M^{2}q_{0}\unicode[STIX]{x0394}q+\cdots \,, & \text{ on }|\unicode[STIX]{x1D701}|=1,\\ q_{0}^{2}+2M^{2}q_{0}\unicode[STIX]{x0394}q+\cdots \,, & \text{ on }|\unicode[STIX]{x1D701}|=\unicode[STIX]{x1D70C}_{0}+M^{2}\hat{\unicode[STIX]{x1D70C}},\end{array}\right.\end{eqnarray}$$

for some constant $\unicode[STIX]{x0394}q$ representing the first-order change in the speed of the fluid on the vortex boundary. This form of the perturbed Bernoulli condition is natural given the assumed symmetry of the compressible vortex street solution we seek. On $|\unicode[STIX]{x1D701}|=\unicode[STIX]{x1D70C}_{0}+M^{2}\hat{\unicode[STIX]{x1D70C}}$ ,

(5.41) $$\begin{eqnarray}\unicode[STIX]{x1D709}_{0}(\unicode[STIX]{x1D701})=\unicode[STIX]{x1D709}_{0}(\unicode[STIX]{x1D701})\biggl|_{|\unicode[STIX]{x1D701}|=\unicode[STIX]{x1D70C}_{0}}+~M^{2}\frac{\hat{\unicode[STIX]{x1D70C}}}{\unicode[STIX]{x1D70C}_{0}}\unicode[STIX]{x1D701}\unicode[STIX]{x1D709}_{0}^{\prime }(\unicode[STIX]{x1D701})\biggr|_{|\unicode[STIX]{x1D701}|=\unicode[STIX]{x1D70C}_{0}}+\cdots \,,\end{eqnarray}$$

which, on use of (5.39) in (5.40), implies that, at $O(M^{2})$ ,

(5.42) $$\begin{eqnarray}\text{Re}\left[\frac{\unicode[STIX]{x1D709}_{1}\overline{\unicode[STIX]{x1D709}_{0}}}{q_{0}^{2}}\right]=\left\{\begin{array}{@{}ll@{}}\displaystyle \frac{\unicode[STIX]{x0394}q}{q_{0}}, & \text{ on }|\unicode[STIX]{x1D701}|=1,\\ \displaystyle \frac{\unicode[STIX]{x0394}q}{q_{0}}-\displaystyle \frac{\hat{\unicode[STIX]{x1D70C}}}{\unicode[STIX]{x1D70C}_{0}}\,\text{Re}\left[\frac{\unicode[STIX]{x1D701}\unicode[STIX]{x1D709}_{0}^{\prime }(\unicode[STIX]{x1D701})\overline{\unicode[STIX]{x1D709}_{0}(\unicode[STIX]{x1D701})}}{q_{0}^{2}}\right], & \text{ on }|\unicode[STIX]{x1D701}|=\unicode[STIX]{x1D70C}_{0},\end{array}\right.\end{eqnarray}$$

where we have also divided by $2q_{0}^{2}$ . On use of (5.38), on both circles $|\unicode[STIX]{x1D701}|=\unicode[STIX]{x1D70C}_{0},1$ , we can write

(5.43) $$\begin{eqnarray}\unicode[STIX]{x1D709}_{1}\overline{\unicode[STIX]{x1D709}_{0}}=q_{0}^{2}\left[\frac{R(\unicode[STIX]{x1D701},\overline{\unicode[STIX]{x1D701}})}{f_{0}^{\prime }(\unicode[STIX]{x1D701})}+\frac{\unicode[STIX]{x1D709}_{0}^{\prime }(\unicode[STIX]{x1D701})}{f_{0}^{\prime }(\unicode[STIX]{x1D701})}{\mathcal{Z}}_{s}(\unicode[STIX]{x1D701})+\unicode[STIX]{x1D6FE}\left[1+\frac{\text{i}\unicode[STIX]{x1D6E4}}{2\unicode[STIX]{x03C0}\unicode[STIX]{x1D701}f_{0}^{\prime }(\unicode[STIX]{x1D701})}\right]+\frac{\tilde{{\mathcal{G}}}^{\prime }(\unicode[STIX]{x1D701})}{f_{0}^{\prime }(\unicode[STIX]{x1D701})}-\frac{\tilde{z}_{1}^{\prime }(\unicode[STIX]{x1D701})}{z_{0}^{\prime }(\unicode[STIX]{x1D701})}\right].\end{eqnarray}$$

On use of this in (5.42) we arrive at the boundary value problem:

(5.44) $$\begin{eqnarray}\text{Re}\left[\frac{\tilde{z}_{1}^{\prime }(\unicode[STIX]{x1D701})}{z_{0}^{\prime }(\unicode[STIX]{x1D701})}\right]=\left\{\begin{array}{@{}l@{}}\text{Re}\left[\displaystyle \frac{R}{f_{0}^{\prime }}+\frac{\unicode[STIX]{x1D709}_{0}^{\prime }}{f_{0}^{\prime }}{\mathcal{Z}}_{s}+\unicode[STIX]{x1D6FE}\left[1+\frac{\text{i}\unicode[STIX]{x1D6E4}}{2\unicode[STIX]{x03C0}\unicode[STIX]{x1D701}f_{0}^{\prime }}\right]+\frac{\tilde{{\mathcal{G}}}^{\prime }}{f_{0}^{\prime }}\right]-\displaystyle \frac{\unicode[STIX]{x0394}q}{q_{0}},\qquad |\unicode[STIX]{x1D701}|=1,\\ \text{Re}\left[\displaystyle \frac{R}{f_{0}^{\prime }}+\frac{\unicode[STIX]{x1D709}_{0}^{\prime }}{f_{0}^{\prime }}{\mathcal{Z}}_{s}+\unicode[STIX]{x1D6FE}\left[1+\frac{\text{i}\unicode[STIX]{x1D6E4}}{2\unicode[STIX]{x03C0}\unicode[STIX]{x1D701}f_{0}^{\prime }}\right]+\frac{\tilde{{\mathcal{G}}}^{\prime }}{f_{0}^{\prime }}\right]-\displaystyle \frac{\unicode[STIX]{x0394}q}{q_{0}}+\displaystyle \frac{\hat{\unicode[STIX]{x1D70C}}}{\unicode[STIX]{x1D70C}_{0}}\,\text{Re}\left[\frac{\unicode[STIX]{x1D701}\unicode[STIX]{x1D709}_{0}^{\prime }\overline{\unicode[STIX]{x1D709}_{0}}}{q_{0}^{2}}\right],\\ |\unicode[STIX]{x1D701}|=\unicode[STIX]{x1D70C}_{0}.\end{array}\right.\end{eqnarray}$$

By separating out terms proportional to the unknown parameters $\hat{\unicode[STIX]{x1D6FC}},\hat{\unicode[STIX]{x1D6FD}},\unicode[STIX]{x0394}U/U,\hat{\unicode[STIX]{x1D70C}}$ and $\unicode[STIX]{x0394}q/q_{0}$ , and on making use of the solution (5.31) for $\tilde{G}(\unicode[STIX]{x1D701})$ derived earlier, this can be written as

(5.45) $$\begin{eqnarray}\text{Re}\left[\frac{\tilde{z}_{1}^{\prime }(\unicode[STIX]{x1D701})}{z_{0}^{\prime }(\unicode[STIX]{x1D701})}\right]=\left\{\begin{array}{@{}l@{}}\text{Re}[T(\unicode[STIX]{x1D701},\overline{\unicode[STIX]{x1D701}})]+\displaystyle \frac{\hat{\unicode[STIX]{x1D6FC}}}{\unicode[STIX]{x1D6FC}_{0}}\text{Re}[A(\unicode[STIX]{x1D701})]+\displaystyle \frac{\hat{\unicode[STIX]{x1D6FD}}}{\unicode[STIX]{x1D6FD}_{0}}\text{Re}[B(\unicode[STIX]{x1D701})]+\frac{\unicode[STIX]{x0394}U}{U}\text{Re}[C(\unicode[STIX]{x1D701})]\\ \quad +\,\displaystyle \frac{\hat{\unicode[STIX]{x1D70C}}}{\unicode[STIX]{x1D70C}_{0}}\text{Re}[D_{0}(\unicode[STIX]{x1D701})]-\displaystyle \frac{\unicode[STIX]{x0394}q}{q_{0}},\qquad \text{on }|\unicode[STIX]{x1D701}|=1,\\ \text{Re}[T(\unicode[STIX]{x1D701},\overline{\unicode[STIX]{x1D701}})]+\displaystyle \frac{\hat{\unicode[STIX]{x1D6FC}}}{\unicode[STIX]{x1D6FC}_{0}}\text{Re}[A(\unicode[STIX]{x1D701})]+\displaystyle \frac{\hat{\unicode[STIX]{x1D6FD}}}{\unicode[STIX]{x1D6FD}_{0}}\text{Re}[B(\unicode[STIX]{x1D701})]+\frac{\unicode[STIX]{x0394}U}{U}\text{Re}[C(\unicode[STIX]{x1D701})]\\ \quad +\,\displaystyle \frac{\hat{\unicode[STIX]{x1D70C}}}{\unicode[STIX]{x1D70C}_{0}}\text{Re}[D_{1}(\unicode[STIX]{x1D701})]-\displaystyle \frac{\unicode[STIX]{x0394}q}{q_{0}},\qquad \text{on }|\unicode[STIX]{x1D701}|=\unicode[STIX]{x1D70C}_{0},\end{array}\right.\end{eqnarray}$$

with

(5.46) $$\begin{eqnarray}\left.\begin{array}{@{}c@{}}T(\unicode[STIX]{x1D701},\overline{\unicode[STIX]{x1D701}})\equiv \frac{\displaystyle R(\unicode[STIX]{x1D701},\overline{\unicode[STIX]{x1D701}})}{\displaystyle f_{0}^{\prime }(\unicode[STIX]{x1D701})}+\frac{\displaystyle V_{1}^{\prime }(\unicode[STIX]{x1D701})}{\displaystyle f_{0}^{\prime }(\unicode[STIX]{x1D701})},\quad A(\unicode[STIX]{x1D701})\equiv \frac{\displaystyle \text{i}L}{\displaystyle 2\unicode[STIX]{x03C0}}\frac{\displaystyle \unicode[STIX]{x1D709}_{0}^{\prime }(\unicode[STIX]{x1D701})K(\unicode[STIX]{x1D701}/\unicode[STIX]{x1D6FC}_{0},\unicode[STIX]{x1D70C}_{0})\displaystyle }{\displaystyle f_{0}^{\prime }(\unicode[STIX]{x1D701})}+\frac{\displaystyle V_{2}^{\prime }(\unicode[STIX]{x1D701})\displaystyle }{\displaystyle f_{0}^{\prime }(\unicode[STIX]{x1D701})},\\ B(\unicode[STIX]{x1D701})\equiv -\frac{\displaystyle \text{i}L}{\displaystyle 2\unicode[STIX]{x03C0}}\frac{\displaystyle \unicode[STIX]{x1D709}_{0}^{\prime }(\unicode[STIX]{x1D701})K(\unicode[STIX]{x1D701}/\unicode[STIX]{x1D6FD}_{0},\unicode[STIX]{x1D70C}_{0})}{\displaystyle f_{0}^{\prime }(\unicode[STIX]{x1D701})}+\frac{\displaystyle V_{3}^{\prime }(\unicode[STIX]{x1D701})}{\displaystyle f_{0}^{\prime }(\unicode[STIX]{x1D701})},\quad C(\unicode[STIX]{x1D701})\equiv \left[1+\frac{\displaystyle \text{i}\unicode[STIX]{x1D6E4}}{\displaystyle 2\unicode[STIX]{x03C0}\unicode[STIX]{x1D701}f_{0}^{\prime }}\right]+\frac{\displaystyle V_{4}^{\prime }(\unicode[STIX]{x1D701})}{\displaystyle f_{0}^{\prime }(\unicode[STIX]{x1D701})},\\ D_{0}(\unicode[STIX]{x1D701})\equiv \frac{\displaystyle V_{5}^{\prime }(\unicode[STIX]{x1D701})}{\displaystyle f_{0}^{\prime }(\unicode[STIX]{x1D701})},\quad D_{1}(\unicode[STIX]{x1D701})\equiv \frac{\displaystyle V_{5}^{\prime }(\unicode[STIX]{x1D701})}{\displaystyle f_{0}^{\prime }(\unicode[STIX]{x1D701})}+\frac{\displaystyle \unicode[STIX]{x1D701}\unicode[STIX]{x1D709}_{0}^{\prime }(\unicode[STIX]{x1D701})\overline{\unicode[STIX]{x1D709}_{0}(\unicode[STIX]{x1D701})}}{\displaystyle q_{0}^{2}}.\end{array}\right\}\end{eqnarray}$$

All functions in (5.46) are independent of $\hat{\unicode[STIX]{x1D6FC}},\hat{\unicode[STIX]{x1D6FD}},\unicode[STIX]{x0394}U/U,\hat{\unicode[STIX]{x1D70C}}$ and $\unicode[STIX]{x0394}q/q_{0}$ . What remains is to determine the latter set of unknown parameters.

It is helpful to introduce the five functions $\{V_{j}(\unicode[STIX]{x1D701})\mid j=6,\ldots ,10\}$ , which are single-valued in the annulus and defined, up to an additive imaginary constant, as the solutions to the modified Schwarz problems in the concentric annulus $\unicode[STIX]{x1D70C}_{0}<|\unicode[STIX]{x1D701}|<1$ :

(5.47) $$\begin{eqnarray}\left.\begin{array}{@{}c@{}}\text{Re}[V_{6}(\unicode[STIX]{x1D701})]=\left\{\begin{array}{@{}ll@{}}\text{Re}[T(\unicode[STIX]{x1D701},\overline{\unicode[STIX]{x1D701}})], & \text{ on }|\unicode[STIX]{x1D701}|=1,\\ \text{Re}[T(\unicode[STIX]{x1D701},\overline{\unicode[STIX]{x1D701}})]+v_{6}, & \text{ on }|\unicode[STIX]{x1D701}|=\unicode[STIX]{x1D70C}_{0},\end{array}\right.\\ \text{Re}[V_{7}(\unicode[STIX]{x1D701})]=\left\{\begin{array}{@{}ll@{}}\text{Re}[A(\unicode[STIX]{x1D701})], & \text{ on }|\unicode[STIX]{x1D701}|=1,\\ \text{Re}[A(\unicode[STIX]{x1D701})]+v_{7}, & \text{ on }|\unicode[STIX]{x1D701}|=\unicode[STIX]{x1D70C}_{0},\end{array}\right.\\ \text{Re}[V_{8}(\unicode[STIX]{x1D701})]=\left\{\begin{array}{@{}ll@{}}\text{Re}[B(\unicode[STIX]{x1D701})], & \text{ on }|\unicode[STIX]{x1D701}|=1,\\ \text{Re}[B(\unicode[STIX]{x1D701})]+v_{8}, & \text{ on }|\unicode[STIX]{x1D701}|=\unicode[STIX]{x1D70C}_{0},\end{array}\right.\\ \text{Re}[V_{9}(\unicode[STIX]{x1D701})]=\left\{\begin{array}{@{}ll@{}}\text{Re}[C(\unicode[STIX]{x1D701})], & \text{ on }|\unicode[STIX]{x1D701}|=1,\\ \text{Re}[C(\unicode[STIX]{x1D701})]+v_{9}, & \text{ on }|\unicode[STIX]{x1D701}|=\unicode[STIX]{x1D70C}_{0},\end{array}\right.\\ \text{Re}[V_{10}(\unicode[STIX]{x1D701})]=\left\{\begin{array}{@{}ll@{}}\text{Re}[D_{0}(\unicode[STIX]{x1D701})], & \text{ on }|\unicode[STIX]{x1D701}|=1,\\ \text{Re}[D_{1}(\unicode[STIX]{x1D701})]+v_{10}, & \text{ on }|\unicode[STIX]{x1D701}|=\unicode[STIX]{x1D70C}_{0}.\end{array}\right.\end{array}\right\}\end{eqnarray}$$

Here the constants $\{v_{j}\mid j=6,\ldots ,10\}$ are all determined by solvability conditions (Crowdy Reference Crowdy2008b ); see appendix D. Then

(5.48) $$\begin{eqnarray}\tilde{z}_{1}^{\prime }(\unicode[STIX]{x1D701})=z_{0}^{\prime }(\unicode[STIX]{x1D701})\left[V_{6}(\unicode[STIX]{x1D701})+\frac{\hat{\unicode[STIX]{x1D6FC}}}{\unicode[STIX]{x1D6FC}_{0}}V_{7}(\unicode[STIX]{x1D701})+\frac{\hat{\unicode[STIX]{x1D6FD}}}{\unicode[STIX]{x1D6FD}_{0}}V_{8}(\unicode[STIX]{x1D701})+\frac{\unicode[STIX]{x0394}U}{U}V_{9}(\unicode[STIX]{x1D701})+\frac{\hat{\unicode[STIX]{x1D70C}}}{\unicode[STIX]{x1D70C}_{0}}V_{10}(\unicode[STIX]{x1D701})-\displaystyle \frac{\unicode[STIX]{x0394}q}{q_{0}}\right],\end{eqnarray}$$

which satisfies the boundary value problem (5.45) provided the condition

(5.49) $$\begin{eqnarray}v_{6}+\frac{\hat{\unicode[STIX]{x1D6FC}}}{\unicode[STIX]{x1D6FC}_{0}}v_{7}+\frac{\hat{\unicode[STIX]{x1D6FD}}}{\unicode[STIX]{x1D6FD}_{0}}v_{8}+\frac{\unicode[STIX]{x0394}U}{U}v_{9}+\frac{\hat{\unicode[STIX]{x1D70C}}}{\unicode[STIX]{x1D70C}_{0}}v_{10}=0\end{eqnarray}$$

holds; this is a constraint on the parameters $\hat{\unicode[STIX]{x1D6FC}},\hat{\unicode[STIX]{x1D6FD}},\unicode[STIX]{x0394}U/U$ and $\hat{\unicode[STIX]{x1D70C}}$ . We also require the two conditions

(5.50) $$\begin{eqnarray}\left.\begin{array}{@{}c@{}}V_{6}(\unicode[STIX]{x1D6FC}_{0})+\frac{\displaystyle \hat{\unicode[STIX]{x1D6FC}}}{\displaystyle \unicode[STIX]{x1D6FC}_{0}}V_{7}(\unicode[STIX]{x1D6FC}_{0})+\frac{\displaystyle \hat{\unicode[STIX]{x1D6FD}}}{\displaystyle \unicode[STIX]{x1D6FD}_{0}}V_{8}(\unicode[STIX]{x1D6FC}_{0})+\frac{\displaystyle \unicode[STIX]{x0394}U}{\displaystyle U}V_{9}(\unicode[STIX]{x1D6FC}_{0})+\frac{\displaystyle \hat{\unicode[STIX]{x1D70C}}}{\displaystyle \unicode[STIX]{x1D70C}_{0}}V_{10}(\unicode[STIX]{x1D6FC}_{0})-\displaystyle \frac{\displaystyle \unicode[STIX]{x0394}q\displaystyle }{q_{0}}=0,\\ V_{6}(\unicode[STIX]{x1D6FD}_{0})+\frac{\displaystyle \hat{\unicode[STIX]{x1D6FC}}}{\displaystyle \unicode[STIX]{x1D6FC}_{0}}V_{7}(\unicode[STIX]{x1D6FD}_{0})+\frac{\displaystyle \hat{\unicode[STIX]{x1D6FD}}}{\displaystyle \unicode[STIX]{x1D6FD}_{0}}V_{8}(\unicode[STIX]{x1D6FD}_{0})+\frac{\displaystyle \unicode[STIX]{x0394}U}{\displaystyle U}V_{9}(\unicode[STIX]{x1D6FD}_{0})+\frac{\displaystyle \hat{\unicode[STIX]{x1D70C}}}{\displaystyle \unicode[STIX]{x1D70C}_{0}}V_{10}(\unicode[STIX]{x1D6FD}_{0})-\displaystyle \frac{\displaystyle \unicode[STIX]{x0394}q}{\displaystyle q_{0}}=0\end{array}\right\}\end{eqnarray}$$

to hold so that the term in square brackets in (5.48) removes the simple pole singularities of $z_{0}^{\prime }(\unicode[STIX]{x1D701})$ at $\unicode[STIX]{x1D701}=\unicode[STIX]{x1D6FC}_{0}$ and $\unicode[STIX]{x1D6FD}_{0}$ , making $\tilde{z}_{1}^{\prime }(\unicode[STIX]{x1D701})$ analytic everywhere in the annulus $\unicode[STIX]{x1D70C}_{0}<|\unicode[STIX]{x1D701}|<1$ . If we denote the coefficients of $\unicode[STIX]{x1D701}^{-1}$ in the Laurent expansion of $V_{j}(\unicode[STIX]{x1D701})z_{0}^{\prime }(\unicode[STIX]{x1D701})$ , for $j=6,\ldots ,10$ , valid on $|\unicode[STIX]{x1D701}|=1$ and on $|\unicode[STIX]{x1D701}|=\unicode[STIX]{x1D70C}_{0}$ by $d_{j}$ and $e_{j}$ , respectively, then we must have

(5.51a,b ) $$\begin{eqnarray}d_{6}+\frac{\hat{\unicode[STIX]{x1D6FC}}}{\unicode[STIX]{x1D6FC}_{0}}d_{7}+\frac{\hat{\unicode[STIX]{x1D6FD}}}{\unicode[STIX]{x1D6FD}_{0}}d_{8}+\frac{\unicode[STIX]{x0394}U}{U}d_{9}+\frac{\hat{\unicode[STIX]{x1D70C}}}{\unicode[STIX]{x1D70C}_{0}}d_{10}=0,\quad e_{6}+\frac{\hat{\unicode[STIX]{x1D6FC}}}{\unicode[STIX]{x1D6FC}_{0}}e_{7}+\frac{\hat{\unicode[STIX]{x1D6FD}}}{\unicode[STIX]{x1D6FD}_{0}}e_{8}+\frac{\unicode[STIX]{x0394}U}{U}e_{9}+\frac{\hat{\unicode[STIX]{x1D70C}}}{\unicode[STIX]{x1D70C}_{0}}e_{10}=0,\end{eqnarray}$$

in order that the primitive function $\tilde{z}_{1}(\unicode[STIX]{x1D701})$ is also single-valued in the annulus $\unicode[STIX]{x1D70C}_{0}<|\unicode[STIX]{x1D701}|<1$ . The latter condition is required in order that the image of the two boundary circles of the annulus under the perturbed mapping function describes the boundaries of two closed curves.

We can write

(5.52) $$\begin{eqnarray}\tilde{z}_{1}(\unicode[STIX]{x1D701})=\tilde{W}_{6}(\unicode[STIX]{x1D701})+\frac{\hat{\unicode[STIX]{x1D6FC}}}{\unicode[STIX]{x1D6FC}_{0}}\tilde{W}_{7}(\unicode[STIX]{x1D701})+\frac{\hat{\unicode[STIX]{x1D6FD}}}{\unicode[STIX]{x1D6FD}_{0}}\tilde{W}_{8}(\unicode[STIX]{x1D701})+\frac{\unicode[STIX]{x0394}U}{U}\tilde{W}_{9}(\unicode[STIX]{x1D701})+\frac{\hat{\unicode[STIX]{x1D70C}}}{\unicode[STIX]{x1D70C}_{0}}\tilde{W}_{10}(\unicode[STIX]{x1D701})-\displaystyle \frac{\unicode[STIX]{x0394}q}{q_{0}}\tilde{W}_{11}(\unicode[STIX]{x1D701})+c_{0},\end{eqnarray}$$

where we set $V_{11}(\unicode[STIX]{x1D701})=1$ and define

(5.53) $$\begin{eqnarray}\tilde{W}_{j}(\unicode[STIX]{x1D701})=\int ^{\unicode[STIX]{x1D701}}V_{j}(\unicode[STIX]{x1D701})z_{0}^{\prime }(\unicode[STIX]{x1D701})\,\text{d}\unicode[STIX]{x1D701},~~j=6,\ldots ,11,\end{eqnarray}$$

where the constant of integration in these functions is chosen so that the constant term in the Laurent series expansions of each on $|\unicode[STIX]{x1D701}|=1$ is zero. The constant $c_{0}$ is chosen to ensure that the perturbed mapping satisfies the symmetry condition

(5.54) $$\begin{eqnarray}z_{0}(-\unicode[STIX]{x1D70C}/\unicode[STIX]{x1D701})+M^{2}z_{1}(-\unicode[STIX]{x1D70C}/\unicode[STIX]{x1D701})=-[z_{0}(\unicode[STIX]{x1D701})+M^{2}z_{1}(\unicode[STIX]{x1D701})]+\cdots \,,\end{eqnarray}$$

where $\unicode[STIX]{x1D70C}=\unicode[STIX]{x1D70C}_{0}+M^{2}\hat{\unicode[STIX]{x1D70C}}$ ; this is the analogue, for the perturbed mapping, of (2.11). On expanding (5.54) in powers of $M^{2}$ we find, at first order in $M^{2}$ , the condition

(5.55) $$\begin{eqnarray}-\left(\frac{\hat{\unicode[STIX]{x1D70C}}}{\unicode[STIX]{x1D70C}_{0}}\right)\frac{\unicode[STIX]{x1D70C}_{0}}{\unicode[STIX]{x1D701}}z_{0}^{\prime }(-\unicode[STIX]{x1D70C}_{0}/\unicode[STIX]{x1D701})+z_{1}(-\unicode[STIX]{x1D70C}_{0}/\unicode[STIX]{x1D701})=-z_{1}(\unicode[STIX]{x1D701}).\end{eqnarray}$$

On substitution of (5.10),

(5.56) $$\begin{eqnarray}\frac{\hat{\unicode[STIX]{x1D70C}}}{\unicode[STIX]{x1D70C}_{0}}\unicode[STIX]{x1D701}z_{0}^{\prime }(\unicode[STIX]{x1D701})+{\mathcal{Z}}_{s}(-\unicode[STIX]{x1D70C}_{0}/\unicode[STIX]{x1D701})+\tilde{z}_{1}(-\unicode[STIX]{x1D70C}_{0}/\unicode[STIX]{x1D701})=-{\mathcal{Z}}_{s}(\unicode[STIX]{x1D701})-\tilde{z}_{1}(\unicode[STIX]{x1D701}),\end{eqnarray}$$

where we have used the identity $-(\unicode[STIX]{x1D70C}_{0}/\unicode[STIX]{x1D701})z_{0}^{\prime }(-\unicode[STIX]{x1D70C}_{0}/\unicode[STIX]{x1D701})=\unicode[STIX]{x1D701}z_{0}^{\prime }(\unicode[STIX]{x1D701})$ following on differentiation of (2.11). But since $\tilde{z}_{1}$ is analytic inside the entire annulus, so that its Laurent series on $|\unicode[STIX]{x1D701}|=1$ of $\tilde{z}_{1}$ is the same as that on $|\unicode[STIX]{x1D701}|=\unicode[STIX]{x1D70C}$ , we can deduce from (5.56) that if, on $|\unicode[STIX]{x1D701}|=1$ , we have

(5.57) $$\begin{eqnarray}-{\mathcal{Z}}_{s}(\unicode[STIX]{x1D701})-{\mathcal{Z}}_{s}(-\unicode[STIX]{x1D70C}_{0}/\unicode[STIX]{x1D701})-\frac{\hat{\unicode[STIX]{x1D70C}}}{\unicode[STIX]{x1D70C}_{0}}\unicode[STIX]{x1D701}z_{0}^{\prime }(\unicode[STIX]{x1D701})=\mathop{\sum }_{n=-\infty }^{\infty }\left[\frac{\hat{\unicode[STIX]{x1D6FC}}}{\unicode[STIX]{x1D6FC}_{0}}t_{2n}+\frac{\hat{\unicode[STIX]{x1D6FD}}}{\unicode[STIX]{x1D6FD}_{0}}t_{3n}+\frac{\hat{\unicode[STIX]{x1D70C}}}{\unicode[STIX]{x1D70C}_{0}}t_{4n}\right]\unicode[STIX]{x1D701}^{n},\end{eqnarray}$$

then

(5.58) $$\begin{eqnarray}c_{0}=\frac{1}{2}\left[\frac{\hat{\unicode[STIX]{x1D6FC}}}{\unicode[STIX]{x1D6FC}_{0}}t_{20}+\frac{\hat{\unicode[STIX]{x1D6FD}}}{\unicode[STIX]{x1D6FD}_{0}}t_{30}+\frac{\hat{\unicode[STIX]{x1D70C}}}{\unicode[STIX]{x1D70C}_{0}}t_{40}\right].\end{eqnarray}$$

As in the incompressible case, we expect to be able to specify the area and the centroid location of the compressible vortices; the speed of the street will then follow. The vortex area denoted by ${\mathcal{A}}$ can be written as

(5.59) $$\begin{eqnarray}{\mathcal{A}}=\frac{1}{2\text{i}}\oint _{|\unicode[STIX]{x1D701}|=1}\overline{z(\unicode[STIX]{x1D701})}z^{\prime }(\unicode[STIX]{x1D701})\,\text{d}\unicode[STIX]{x1D701}={\mathcal{A}}_{0}+M^{2}{\mathcal{A}}_{1}+\cdots \,,\end{eqnarray}$$

where

(5.60a,b ) $$\begin{eqnarray}{\mathcal{A}}_{0}=\frac{1}{2\text{i}}\oint _{|\unicode[STIX]{x1D701}|=1}\overline{z_{0}}z_{0}^{\prime }(\unicode[STIX]{x1D701})\,\text{d}\unicode[STIX]{x1D701},\quad {\mathcal{A}}_{1}=\frac{1}{2\text{i}}\oint _{|\unicode[STIX]{x1D701}|=1}[\overline{z_{1}(\unicode[STIX]{x1D701})}z_{0}^{\prime }(\unicode[STIX]{x1D701})+\overline{z_{0}(\unicode[STIX]{x1D701})}z_{1}^{\prime }(\unicode[STIX]{x1D701})]\,\text{d}\unicode[STIX]{x1D701}.\end{eqnarray}$$

The centroid ${\mathcal{C}}$ of the vortex that is the image of $|\unicode[STIX]{x1D701}|=1$ is given by

(5.61a-c ) $$\begin{eqnarray}{\mathcal{C}}=\frac{\displaystyle \oint _{|\unicode[STIX]{x1D701}|=1}z(\unicode[STIX]{x1D701})|z^{\prime }(\unicode[STIX]{x1D701})|\,\frac{\text{d}\unicode[STIX]{x1D701}}{\unicode[STIX]{x1D701}}}{\displaystyle \oint _{|\unicode[STIX]{x1D701}|=1}|z^{\prime }(\unicode[STIX]{x1D701})|\,\frac{\text{d}\unicode[STIX]{x1D701}}{\unicode[STIX]{x1D701}}}={\mathcal{C}}_{0}+M^{2}{\mathcal{C}}_{1}+\cdots \,,\quad {\mathcal{C}}_{0}=\frac{N_{0}}{N_{2}},\quad {\mathcal{C}}_{1}=\frac{N_{1}}{N_{2}}-\frac{N_{0}N_{3}}{N_{2}^{2}},~~\end{eqnarray}$$

with

(5.62) $$\begin{eqnarray}\left.\begin{array}{@{}c@{}}N_{0}=\displaystyle \oint _{|\unicode[STIX]{x1D701}|=1}z_{0}(\unicode[STIX]{x1D701})|z_{0}^{\prime }(\unicode[STIX]{x1D701})|\,\frac{\text{d}\unicode[STIX]{x1D701}}{\unicode[STIX]{x1D701}},\quad N_{1}=\displaystyle \oint _{|\unicode[STIX]{x1D701}|=1}\left\{z_{1}(\unicode[STIX]{x1D701})+z_{0}(\unicode[STIX]{x1D701})\,\text{Re}\left[\frac{\displaystyle z_{1}^{\prime }(\unicode[STIX]{x1D701})}{\displaystyle z_{0}^{\prime }(\unicode[STIX]{x1D701})}\right]\right\}|z_{0}^{\prime }(\unicode[STIX]{x1D701})|\,\frac{\displaystyle \text{d}\unicode[STIX]{x1D701}}{\displaystyle \unicode[STIX]{x1D701}},\\ N_{2}=\displaystyle \oint _{|\unicode[STIX]{x1D701}|=1}|z_{0}^{\prime }(\unicode[STIX]{x1D701})|\,\frac{\displaystyle \text{d}\unicode[STIX]{x1D701}}{\displaystyle \unicode[STIX]{x1D701}},\quad N_{3}=\displaystyle \oint _{|\unicode[STIX]{x1D701}|=1}\text{Re}\left[\frac{z_{1}^{\prime }(\unicode[STIX]{x1D701})}{z_{0}^{\prime }(\unicode[STIX]{x1D701})}\right]|z_{0}^{\prime }(\unicode[STIX]{x1D701})|\,\frac{\text{d}\unicode[STIX]{x1D701}}{\unicode[STIX]{x1D701}},\end{array}\right\}\end{eqnarray}$$

where we have made use of the fact that

(5.63) $$\begin{eqnarray}|z_{0}^{\prime }(\unicode[STIX]{x1D701})+M^{2}z_{1}^{\prime }(\unicode[STIX]{x1D701})|=|z_{0}^{\prime }(\unicode[STIX]{x1D701})|+M^{2}|z_{0}^{\prime }(\unicode[STIX]{x1D701})|\,\text{Re}\left[\frac{z_{1}^{\prime }(\unicode[STIX]{x1D701})}{z_{0}^{\prime }(\unicode[STIX]{x1D701})}\right].\end{eqnarray}$$

Since the circulation and perimeter ${\mathcal{P}}$ of each vortex satisfy

(5.64) $$\begin{eqnarray}q{\mathcal{P}}=\unicode[STIX]{x1D6E4},\end{eqnarray}$$

where $q=q_{0}+M^{2}\unicode[STIX]{x0394}q$ and where $\unicode[STIX]{x1D6E4}$ is fixed, then it is easy to show that we must have

(5.65) $$\begin{eqnarray}\frac{\unicode[STIX]{x0394}q}{q_{0}}=-\frac{N_{3}}{N_{2}},\end{eqnarray}$$

and this provides a check on the solution.

We assume that all five unknown quantities $\hat{\unicode[STIX]{x1D6FC}},\hat{\unicode[STIX]{x1D6FD}},\hat{\unicode[STIX]{x1D70C}},\unicode[STIX]{x0394}U/U$ and $\unicode[STIX]{x0394}q/q_{0}$ are real; this is checked for consistency later. It turns out that the four parameters $\hat{\unicode[STIX]{x1D6FC}},\hat{\unicode[STIX]{x1D6FD}},\hat{\unicode[STIX]{x1D70C}}$ and $\unicode[STIX]{x0394}U/U$ can be found first, with the value of $\unicode[STIX]{x0394}q/q_{0}$ following a posteriori, from either of the two equations (5.50), which are found to be linearly dependent in all cases (by the symmetry). Two equations for the four parameters $\hat{\unicode[STIX]{x1D6FC}},\hat{\unicode[STIX]{x1D6FD}},\hat{\unicode[STIX]{x1D70C}}$ and $\unicode[STIX]{x0394}U/U$ are provided by our choices

(5.66a,b ) $$\begin{eqnarray}{\mathcal{A}}_{1}=0,\quad \text{Im}[{\mathcal{C}}_{1}]=0,\end{eqnarray}$$

which ensures the area of the vortices and the aspect ratio of the street are unchanged to first order in $M^{2}$ . Equation (5.49) provides a third equation, and is found to be satisfied provided we choose

(5.67) $$\begin{eqnarray}\frac{\hat{\unicode[STIX]{x1D6FC}}}{\unicode[STIX]{x1D6FC}_{0}}+\frac{\hat{\unicode[STIX]{x1D6FD}}}{\unicode[STIX]{x1D6FD}_{0}}=\frac{\hat{\unicode[STIX]{x1D70C}}}{\unicode[STIX]{x1D70C}_{0}}.\end{eqnarray}$$

The latter is equivalent to the condition $\unicode[STIX]{x1D6FC}\unicode[STIX]{x1D6FD}=-\unicode[STIX]{x1D70C}$ not just being satisfied at leading order, but also being correct to first order in $M^{2}$ . This might have been enforced a priori based on symmetry requirements of the perturbed vortices. Finally, either choice of the two equations in (5.51) provides the fourth and final equation for $\hat{\unicode[STIX]{x1D6FC}},\hat{\unicode[STIX]{x1D6FD}},\hat{\unicode[STIX]{x1D70C}}$ and $\unicode[STIX]{x0394}U/U$ . Conditions (5.50) also force all as yet undetermined purely imaginary constants in the definitions of $\{V_{j}\mid j=1,\ldots ,5\}$ to vanish.

6 Characterization of the weakly compressible vortex streets

We set length and time scales with the choices $\unicode[STIX]{x1D6E4}=L=1$ . We define the street aspect ratio $\unicode[STIX]{x1D705}$ to be

(6.1) $$\begin{eqnarray}\unicode[STIX]{x1D705}=\frac{h}{L},\end{eqnarray}$$

where $h$ denotes the vertical separation of the vortex centroids of the two vortices in each period window.

The effect of vortex area on the speed of the street is shown in figure 2 for a selection of street aspect ratios. An important check on the foregoing analysis is provided by the small-area limit, where we expect the results here to tend to those given by an independent analysis of the weakly compressible point vortex streets recently calculated by Crowdy & Krishnamurthy (Reference Crowdy and Krishnamurthy2017), who find formula (1.1) for the first-order relative change in the speed of a staggered street. This is found to be the case. The values of $\unicode[STIX]{x0394}U/U$ predicted by (1.1) are indicated by solid dots on the vertical axis of figure 2. This reassures us that the numerical scheme described in previous sections is faithfully computing the finite-area continuations of the compressible point vortex result (1.1). Table 1 provides some typical parameter values obtained using our method.

Figure 2.

Graph of the relative change in the speed of the vortex street $\unicode[STIX]{x0394}U/U$ against vortex area for fixed street aspect ratio $\unicode[STIX]{x1D705}=0.2,0.28$ and $0.34$ . The dots on the vertical axis at zero area show the results of the analysis of the point vortex street of Crowdy & Krishnamurthy (Reference Crowdy and Krishnamurthy2017).

Table 1.

A representative table of parameters values for $\unicode[STIX]{x1D705}=0.28$ . For the given $\unicode[STIX]{x1D70C}_{0}$ , the incompressible parameters $\{\unicode[STIX]{x1D6FC}_{0},\unicode[STIX]{x1D6FD}_{0},\unicode[STIX]{x1D6FE}_{1},\unicode[STIX]{x1D6FE}_{2},U\}$ are found, using the prescription described in § 2. The parameters $\{\unicode[STIX]{x1D70C}_{1},\unicode[STIX]{x1D6FC}_{1},\unicode[STIX]{x1D6FD}_{1},\unicode[STIX]{x0394}U\}$ associated with the first-order compressible correction are then calculated using the prescription described in § 5.

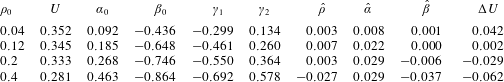
We focus on aspect ratios around the value $0.28$ ; this is close to the isolated value for neutral linear stability of the von Kármán point vortex streets, and it has been established (Jimenez Reference Jimenez1987) that the same isolated neutral stability point exists even for finite-area incompressible vortex street models with a Hamiltonian structure. Given these special stability features, to study the properties of steady compressible streets close to $\unicode[STIX]{x1D705}=0.28$ would seem to be of particular interest (although it must be emphasized that we say nothing here on the stability of these compressible vortex equilibria). Moreover, as $\unicode[STIX]{x1D705}\rightarrow 0$ , the staggered street tends to an arrangement comprising a single periodic row of counter-rotating vortices. A study of compressibility effects on such a vortex configuration – albeit within a different model of the vortex cores – has already been carried out by O’Reilly & Pullin (Reference O’Reilly and Pullin2005), so we do not focus on it here.

We find that compressibility tends to increase the speed of streets of small vortices but that there is a critical vortex area at which no change in the speed of the street at first order in $M^{2}$ occurs. For streets with vortices of area greater than this critical size, compressibility decreases the speed of the street.

Figure 3.

Critical value of the normalized effective core radius $R_{c}/L$ as a function of $\unicode[STIX]{x1D705}/2$ , at which $\unicode[STIX]{x0394}U=0$ , so that compressibility does not alter the speed of the street at first order in $M^{2}$ . The linear approximation (6.3) is also shown (dotted line).

The presence of a critical vortex core size for which compressibility does not affect the street speed at first order in Mach number is interesting. For aspect ratio $\unicode[STIX]{x1D705}=0.28$ , we find the critical area to be $0.0655$ . The associated vortices are not circular but we can nevertheless infer an effective core radius $R_{c}$ associated with this critical area by assuming a circular shape and setting

(6.2) $$\begin{eqnarray}\unicode[STIX]{x03C0}\left(\frac{R_{c}}{L}\right)^{2}=0.0665\quad \text{implying}~R_{c}\approx 0.14L.\end{eqnarray}$$

In this case, the normalized effective radius $R_{c}$ corresponds approximately to the vertical distance of each vortex from the centreline $y=0$ of the two vortex rows, i.e. $\unicode[STIX]{x1D705}/2$ , for this aspect ratio. A graph of $R_{c}/L$ against $\unicode[STIX]{x1D705}/2$ is shown in figure 3 and shows a near-linear behaviour closely approximated by

(6.3) $$\begin{eqnarray}\frac{R_{c}}{L}=0.22\left(\frac{\unicode[STIX]{x1D705}}{2}\right)+0.11,\end{eqnarray}$$

which may be useful in providing ready estimates of the critical vortex area at which vortices are unaffected by compressibility to leading order in $M^{2}$ .

Figure 4.

Graph of the first-order relative change in the fluid speed on the vortex boundary $\unicode[STIX]{x0394}q/q_{0}$ against vortex area for fixed street aspect ratio $\unicode[STIX]{x1D705}=0.2,0.28$ and $0.34$ .

Figure 5.

Graph of the relative change in the speed of the vortex street $\unicode[STIX]{x0394}U/U$ against street aspect ratio $\unicode[STIX]{x1D705}$ for fixed values of vortex area $0.04,0.1$ and $0.2$ .

Figure 6.

Effect of compressibility on the vortex shapes: (a) for $\unicode[STIX]{x1D705}=0.28$ and $\unicode[STIX]{x1D70C}=0.122$ , for which the vortex area is at its critical value $0.0654$ ; and (b) for $\unicode[STIX]{x1D705}=0.2$ and $\unicode[STIX]{x1D70C}=0.5$ , for which the vortex area is $0.2485$ . The incompressible vortices (dashed line) and the compressible analogues (solid line) having the same area are superposed. The value $M^{2}=0.4$ is taken in order to exaggerate the qualitative difference in shapes for the compressible vortices.

Figure 4 shows the first-order relative change in the fluid speed on the boundary $\unicode[STIX]{x0394}q/q_{0}$ as a function of vortex area for the fixed street aspect ratios $\unicode[STIX]{x1D705}=0.2,0.28$ and $0.34$ . It is found to be negative, which means, since the circulation of the vortices is fixed to be the same as in the incompressible case, that the perimeter of the perturbed compressible vortices increases relative to their incompressible counterparts.

Figure 5 shows the first-order relative change in the speed of the street $\unicode[STIX]{x0394}U/U$ as a function of street aspect ratio for three fixed values of the vortex area $0.04,0.1$ and $0.2$ . A notable feature of both figures 2 and 5 is that, as the aspect ratio of the street decreases towards zero, the magnitude of the first-order relative change in the compressible speed becomes large. This singular-looking behaviour is to be expected, however, since, as pointed out earlier, our choice of Mach number based on the speed of translation of the incompressible street tends to zero as $\unicode[STIX]{x1D705}\rightarrow 0$ (that is, our choice of expansion parameter $M$ is tending to zero). A vortex street with $\unicode[STIX]{x1D705}=0$ reduces to the situation of a single row of vortices of alternating sign and this arrangement is not of particular interest to us here given our focus on vortex wakes. A Rayleigh–Jansen analysis of this scenario would require a different choice of Mach number as the expansion parameter.

Figure 5 shows that the relative change in speed of the street due to compressibility tends to zero as $\unicode[STIX]{x1D705}$ increases in all cases. For vortex area $0.04$ , the relative change in compressible street speed is around 7 % for $\unicode[STIX]{x1D705}=0.28$ and it gets smaller as $\unicode[STIX]{x1D705}$ increases further.

Finally, we document the effect of compressibility on the vortex shapes, and this is represented in figure 6 for two choices of vortex street of different aspect ratio and vortex areas. The same qualitative trend appears to be true in all cases: compressibility leads to an elongation of the vortices in the cross-stream direction, with the vortices becoming more slender in the streamwise direction. These effects become more pronounced, for a given Mach number, for vortices of larger area, as shown in figure 6. As already pointed out with reference to figure 4, the perimeter of the perturbed vortices generally increases at first order in $M^{2}$ relative to the incompressible counterparts of the same area, circulation and aspect ratio. These observations on the shape deformations due to weak compressibility are consistent with analogous observations made by O’Reilly & Pullin (Reference O’Reilly and Pullin2005) in their numerical examination of the compressible analogues of the smooth counter-rotating vortex pair found by Mallier & Maslowe (Reference Mallier and Maslowe1993). O’Reilly & Pullin (Reference O’Reilly and Pullin2005) noticed that compressibility acts to increase the strain rates in the smooth vortex cores, causing substantial stretching of the vortices in the transverse direction to the axis of the vortex row.