Hostname: page-component-cb9f654ff-65tv2 Total loading time: 0 Render date: 2025-08-29T22:37:27.408Z Has data issue: false hasContentIssue false
  • English
  • Français

Effects of flexibility on the aerodynamic performance of flapping wings

Published online by Cambridge University Press:  23 November 2011

C.-K. Kang ,
H. Aono ,
C. E. S. Cesnik  and
W. Shyy
C.-K. Kang
Affiliation:
Department of Aerospace Engineering, University of Michigan, Ann Arbor, MI 48109, USA
H. Aono
Affiliation:
Department of Aerospace Engineering, University of Michigan, Ann Arbor, MI 48109, USA
C. E. S. Cesnik
Affiliation:
Department of Aerospace Engineering, University of Michigan, Ann Arbor, MI 48109, USA
W. Shyy*
Affiliation:
Department of Aerospace Engineering, University of Michigan, Ann Arbor, MI 48109, USA Department of Mechanical Engineering, Hong Kong University of Science and Technology, Kowloon, Hong Kong
*
†Email address for correspondence: weishyy@ust.hk
Get access Rights & Permissions [Opens in a new window]

Abstract

Effects of chordwise, spanwise, and isotropic flexibility on the force generation and propulsive efficiency of flapping wings are elucidated. For a moving body immersed in viscous fluid, different types of forces, as a function of the Reynolds number, reduced frequency (k), and Strouhal number (St), acting on the moving body are identified based on a scaling argument. 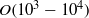In particular, at the Reynolds number regime of and the reduced frequency of 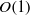, the added mass force, related to the acceleration of the wing, is important. Based on the order of magnitude and energy balance arguments, a relationship between the propulsive force and the maximum relative wing-tip deformation parameter (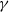) is established. The parameter depends on the density ratio, St, k, natural and flapping frequency ratio, and flapping amplitude. The lift generation, and the propulsive efficiency can be deduced by the same scaling procedures. It seems that the maximum propulsive force is obtained when flapping near the resonance, whereas the optimal propulsive efficiency is reached when flapping at about half of the natural frequency; both are supported by the reported studies. The established scaling relationships can offer direct guidance for micro air vehicle design and performance analysis.

JFM classification

Biological Fluid Dynamics: Propulsion Biological Fluid Dynamics: Swimming/flying

Information

Type
Papers
Information
Journal of Fluid Mechanics , Volume 689 , 25 December 2011 , pp. 32 - 74
Copyright
Copyright © Cambridge University Press 2011

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

1. Aono, H., Chimakurthi, S. K., Wu, P., Sällström, E., Stanford, B. K., Cesnik, C. E. S., Ifju, P., Ukeiley, L. & Shyy, W. 2010 A computational and experimental study of flexible flapping wing aerodynamics. AIAA Paper 2010–554.CrossRefGoogle Scholar
2. Azuma, A. 2006 The Biokinetics of Flying and Swimming, 2nd edn. AIAA.CrossRefGoogle Scholar
3. Balay, S., Brown, J., Buschelman, K., Eijkhout, V., Gropp, W. D., Kaushik, D., Knepley, M. G., McInnes, L. C., Smith, B. F. & Zhang, H. 2010 PETSc users manual. Tech. Rep. Argonne National Laboratory.Google Scholar
4. Balay, S., Brown, J., Buschelman, K., Gropp, W. D., Kaushik, D., Knepley, M. G., McInnes, L. C., Smith, B. F. & Zhang, H. 2011 PETSc web page.http://www.mcs.anl.gov/petsc.Google Scholar
5. Balay, S., Gropp, W. D., McInnes, L. C. & Smith, B. F. 1997 Efficient management of parallelism in object oriented numerical software libraries. In Modern Software Tools for Scientific Computing, pp. 163–202. Birkhäuser.Google Scholar
6. Barenblatt, G. I. 2003 Scaling. Cambridge University Press.Google Scholar
7. Biewener, A. A. 2003 Animal Locomotion. Oxford University Press.Google Scholar
8. Bisplinghoff, R. L., Ashley, H. & Halfman, R. L. 1996 Aeroelasticity. Dover.Google Scholar
9. de Boer, A., van der Schoot, M. S. & Bijl, H. 2007 Mesh deformation based on radial basis function interpolation. Comput. Struct. 85 (11–14), 784–795.CrossRefGoogle Scholar
10. Bos, F. M., Lentink, D., van Oudheusden, B. W. & Bijl, H. 2008 Influence of wing kinematics on aerodynamic performance in hovering insect flight. J. Fluid Mech. 594, 341–368.CrossRefGoogle Scholar
11. Buchwald, R. & Dudley, R. 2010 Limits to vertical force and power production in bumblebees (Hymenoptera: Bombus impatiens). J. Expl Biol. 213, 426–432.CrossRefGoogle ScholarPubMed
12. Chen, J.-S., Chen, J.-Y. & Chou, Y.-F. 2008 On the natural frequencies and mode shapes of dragonfly wings. J. Sound Vib. 313, 643–654.CrossRefGoogle Scholar
13. Chimakurthi, S. K., Cesnik, C. E. S. & Stanford, B. 2011 Flapping-wing structural dynamics formulation based on a corotational shell finite element. AIAA J. 49 (1), 128–142.CrossRefGoogle Scholar
14. Chimakurthi, S. K., Tang, J., Palacios, R., Cesnik, C. E. S. & Shyy, W. 2009 Computational aeroelasticity framework for analysing flapping wing micro air vehicles. AIAA J. 47, 1865–1878.Google Scholar
15. Combes, S. A. & Daniel, T. L. 2003a Flexural stiffness in insect wings. Part I. Scaling and the influence of wing venation. J. Expl Biol. 206 (17), 2979–2987.Google Scholar
16. Combes, S. A. & Daniel, T. L. 2003b Into thin air: contributions of aerodynamic and inertial-elastic forces to wing bending in the hawkmoth Manduca sexta. J. Expl Biol. 206 (17), 2999–3006.Google Scholar
17. Daniel, T. L. & Combes, S. A. 2002 Flexible wings and fins: bending by inertial or fluid-dynamic forces? Integr. Compar. Biol. 42 (5), 1044–1049.CrossRefGoogle ScholarPubMed
18. Dudley, R. 2002 The Biomechanics of Insect Flight: Form, Function, Evolution. Princeton University Press.Google Scholar
19. Falgout, R. & Yang, U. 2002 hypre: a library of high performance preconditioners. In Computational Science ICCS 2002 (ed. Sloot, P., Hoekstra, A., Tan, C. & Dongarra, J. ), pp. 632–641. Springer.CrossRefGoogle Scholar
20. Ferreira de Sousa, P. J. S. A. & Allen, J. J. 2011 Thrust efficiency of harmonically oscillating flexible flat plates. J. Fluid Mech. 674, 43–66.CrossRefGoogle Scholar
21. Garrick, I. E. 1937 Propulsion of a flapping and oscillating aerofoil. NACA report 567, 419–427.Google Scholar
22. Gogulapati, A. & Friedmann, P. 2011 Approximate aerodynamic and aeroelastic modeling of flapping wings in hover and forward flight. AIAA Paper 2011–2008.Google Scholar
23. Gordnier, R. E., Attar, P. J., Chimakurthi, S. K. & Cesnik, C. E. S. 2010 Implicit LES simulations of a flexible flapping wing. AIAA Paper 2010–2960.Google Scholar
24. Graebel, W. 2007 Advanced Fluid Mechanics. Academic Press.Google Scholar
25. Heathcote, S. & Gursul, I. 2007 Flexible flapping aerofoil propulsion at low Reynolds numbers. AIAA J. 45 (5), 1066–1079.CrossRefGoogle Scholar
26. Heathcote, S., Wang, Z. & Gursul, I. 2008 Effect of spanwise flexibility on flapping wing propulsion. J. Fluids Struct. 24 (2), 183–199.Google Scholar
27. Ishihara, D., Horie, T. & Denda, M. 2009a A two-dimensional computational study on the fluid-structure interaction cause of wing pitch changes in dipteran flapping flight. J. Expl Biol. 212 (1), 1–10.CrossRefGoogle Scholar
28. Ishihara, D., Yamashita, Y., Horie, T., Yoshida, S. & Niho, T. 2009b Passive maintenance of high angle of attack and its generation during flapping translation in crane fly wing. J. Expl Biol. 212, 3882–3891.CrossRefGoogle ScholarPubMed
29. Kamakoti, R. & Shyy, W. 2004 Evaluation of geometric conservation law using pressure-based fluid solver and moving grid technique. Intl J. Heat Fluid Flow 14 (7), 851–865.CrossRefGoogle Scholar
30. Kamakoti, R., Thakur, S., Wright, J. & Shyy, W. 2006 Validation of a new parallel all-speed CFD code in a rule-based framework for multidisciplinary applications. AIAA Paper 2006–3063.Google Scholar
31. Kang, C.-K., Aono, H., Cesnik, C. E. S. & Shyy, W. 2011 A scaling parameter for thrust generation of flapping flexible wings. AIAA Paper 2011–1313.CrossRefGoogle Scholar
32. Katz, J. & Plotkin, A. 2001 Low-Speed Aerodynamics. Cambridge University Press.CrossRefGoogle Scholar
33. Khosravi, P., Ganesan, R. & Sedaghati, R. 2007 Corotational nonlinear analysis of thin plates and shells using a new shell element. Intl J. Numer. Meth. Engng 69 (4), 859–885.Google Scholar
34. Kim, D. & Gharib, M. 2011 Flexibility effects on vortex formation of translating plates. J. Fluid Mech. 677, 255–271.Google Scholar
35. Küttler, U. & Wall, W. A. 2008 Fixed-point fluid-structure interaction solvers with dynamic relaxation. Comput. Mech. 43, 61–72.CrossRefGoogle Scholar
36. Luke, E. A. & George, T. 2005 Loci: a rule-based framework for parallel multi-disciplinary simulation synthesis. J. Funct. Program 15 (03), 477–502.Google Scholar
37. Masoud, H. & Alexeev, A. 2010 Resonance of flexible flapping wings at low Reynolds number. Phys. Rev. E 81, 056304.Google Scholar
38. Maxworthy, T. 1981 The fluid dynamics of insect flight. Annu. Rev. Fluid. Mech. 13, 329–350.CrossRefGoogle Scholar
39. Michelin, S. & Llewellyn Smith, S. G. 2009 Resonance and propulsion performance of a heaving flexible wing. Phys. Fluids 21, 071902.CrossRefGoogle Scholar
40. Noca, F. 1997 On the evaluation of time-dependent fluid-dynamic forces on bluff bodies. PhD thesis, California Institute of Technology.Google Scholar
41. Norberg, U. M. 1990 Vertebrate Flight: Mechanics, Physiology, Morphology, Ecology, and Evolution. Springer.CrossRefGoogle Scholar
42. Pennycuick, C. J. 1996 Wingbeat frequency of birds in steady cruising flight: new data and improved predictions. J. Expl Biol. 199, 1613–1618.CrossRefGoogle ScholarPubMed
43. Ramananarivo, S., Godoy-Diana, R. & Thiria, B. 2011 Rather than resonance, flapping wing flyers may play on aerodynamics to improve performance. Proc. Natl. Acad. Sci. USA 108 (15), 5964–5969.CrossRefGoogle ScholarPubMed
44. Saffman, P. G. 1995 Vortex Dynamics. Cambridge University Press.Google Scholar
45. Sane, S. P 2003 The aerodynamics of insect flight. J. Expl Biol. 206, 4191–4208.Google Scholar
46. Shevtsova, E., Hansson, C., Janzen, D. H. & Kjaerandsen, J. 2011 Stable structural colour patterns displayed on transparent insect wings. Proc. Natl. Acad. Sci. USA 108 (213), 668–673.CrossRefGoogle ScholarPubMed
47. Shyy, W., Aono, H., Chimakurthi, S. K., Trizila, P., Kang, C.-K., Cesnik, C. E. S. & Liu, H. 2010 Recent progress in flapping wing aerodynamics and aeroelasticity. Prog. Aerosp. Sci. 46 (7), 284–327.Google Scholar
48. Shyy, W., Berg, M. & Ljungqvist, D. 1999 Flapping and flexible wings for biological and micro air vehicles. Prog. Aerosp. Sci. 35 (5), 455–505.Google Scholar
49. Shyy, W., Lian, L., Tang, J., Liu, H., Trizila, P., Stanford, B., Bernal, L. P., Cesnik, C. E. S., Friedmann, P. & Ifju, P. 2008a Computational aerodynamics of low Reynolds number plunging, pitching and flexible wings for MAV applications. Acta Mechanica Sin. 24, 351–373.CrossRefGoogle Scholar
50. Shyy, W., Lian, Y., Tang, J., Viieru, D. & Liu, H. 2008b Aerodynamics of Low Reynolds Number Flyers. Cambridge University Press.Google Scholar
51. Shyy, W., Trizila, P., Kang, C.-K. & Aono, H. 2009 Can tip vortices enhance lift of a flapping wing? AIAA 47, 289–293.Google Scholar
52. Smith, R. W. & Wright, J. A. 2003 An implicit edge-based ale method for the incompressible Navier–Stokes equations. Intl J. Numer. Meth. Fluids 43, 253–279.CrossRefGoogle Scholar
53. Spagnolie, S. E., Moret, L., Shelley, M. J. & Zhang, J. 2010 Surprising behaviours in flapping locomotion with passive pitching. Phys. Fluids 22, 041903.CrossRefGoogle Scholar
54. Sunada, S., Zeng, L. & Kawachi, K. 1998 The relationship between dragonfly wing structure and torsional deformation. J. Theor. Biol. 193, 39–45.CrossRefGoogle Scholar
55. Theodorsen, T. 1935 General theory of aerodynamic instability and the mechanism of flutter. Tech. Rep. NACA report.Google Scholar
56. Thiria, B. & Godoy-Diana, R. 2010 How wing compliance drives the efficiency of self-propelled flapping flyers. Phys. Rev. E 82 (1), 015303.Google Scholar
57. Thomas, P. D. & Lombard, C. K. 1979 Geometric conservation law and its application to flow computations on moving grids. AIAA J. 17 (10), 1030–1037.Google Scholar
58. Timoshenko, S., Young, D. H. & Weaver, J. R. W. 1974 Vibration Problems in Engineering. Wiley.Google Scholar
59. Tobalske, B. W. 2007 Biomechanics of bird flight. J. Expl Biol. 210, 3135–3146.Google Scholar
60. Triantafyllou, M. S., Triantafyllou, G. S. & Yue, D. K. P. 2000 Hydrodynamics of fishlike swimming. Annu. Rev. Fluid. Mech. 32, 33–53.CrossRefGoogle Scholar
61. Trizila, P., Kang, C.-K., Aono, H., Visbal, M. & Shyy, W. 2011 Low-Reynolds-number aerodynamics of a flapping rigid flat plate. AIAA J. 49 (4), 806–823.Google Scholar
62. Vanella, M., Fitzgerald, T., Preidikman, S., Balaras, E. & Balachandran, B. 2009 Influence of flexibility on the aerodynamic performance of a hovering wing. J. Expl Biol. 212, 95–105.Google Scholar
63. Visbal, M. R., Gordnier, R. E. & Galbraith, M. C. 2009 High-fidelity simulations of moving and flexible aerofoils at low Reynolds numbers. Exp. Fluids 46, 903–922.Google Scholar
64. Vogel, S. 1966 Flight in Drosophila. Part I. Flight performance of tethered flies. J. Expl Biol. 44, 567–578.CrossRefGoogle Scholar
65. Wang, Z. J. 2008 Aerodynamic efficiency of flapping flight: analysis of a two-stroke model. J. Expl Biol. 211, 234–238.CrossRefGoogle ScholarPubMed
66. Whitney, J. P. & Wood, R. J. 2010 Aeromechanics of passive rotation in flapping flight. J. Fluid Mech. 660, 197–220.Google Scholar
67. Willmott, A. P. & Ellington, C. P. 1997a The mechanics of flight in the hawkmoth Manduca sexta. Part I. Kinematics of hovering and forward flight. J. Expl Biol. 200, 2705–2722.CrossRefGoogle Scholar
68. Willmott, A. P. & Ellington, C. P. 1997b The mechanics of flight in the hawkmoth Manduca sexta. Part II. Aerodynamic consequences of kinematic and morphological variation. J. Expl Biol. 200, 2723–2745.Google Scholar
69. Wright, J. A. & Smith, R. W 2001 An edge-based method for the incompressible Navier–Stokes equations on polygonal meshes. J. Comput. Phys. 169, 24–43.Google Scholar
70. Wu, P., Ijfu, P. & Stanford, B. 2010 Flapping wing structural deformation and thrust correlation study with flexible membrane wings. AIAA J. 48 (9), 2111–2122.Google Scholar
71. Yin, B. & Luo, H. 2010 Effect of wing inertia on hovering performance of flexible flapping wings. Phys. Fluids 22, 111902.Google Scholar
72. Zhang, J., Liu, N.-S. & Lu, X.-Y. 2010 Locomotion of a passively flapping flat plate. J. Fluid Mech. 659, 43–68.CrossRefGoogle Scholar