Article contents
Flow and heat transfer in convectively unstable turbulent channel flow with solid-wall heat conduction
Published online by Cambridge University Press: 19 September 2014
Abstract
Most turbulent coherent structures in a convectively unstable atmospheric boundary layer are caused by or manifested in ascending warm fluid and descending cold fluids. These structures not only cause ramps in the air temperature timeseries, but also imprint on the underlying solid surface as surface temperature fluctuations. The coupled flow and heat transport mechanism was examined through direct numerical simulation (DNS) of a channel flow allowing for realistic solid–fluid thermal coupling. The thermal activity ratio (TAR; the ratio of thermal inertias of fluid and solid), and the thickness of the solid domain were found to affect the solid–fluid interfacial temperature variations. The solid–fluid interface with large (small) thermal activity ration behaves as an isoflux (isothermal) boundary. For the range of parameters considered here (Grashof number, 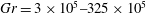 $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}\mathit{Gr} = 3\times 10^5\text {--} 325\times 10^5$ ;
$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}\mathit{Gr} = 3\times 10^5\text {--} 325\times 10^5$ ; 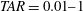 $\textit {TAR} = 0.01\text {--}1$ ; solid thickness normalized by heat penetration
$\textit {TAR} = 0.01\text {--}1$ ; solid thickness normalized by heat penetration 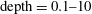 $\text {depth} = 0.1\text {--}10$ ), the solid thermal properties and thickness influence the fluid temperature only in the viscous or conduction region while the convective forcing influences the turbulent flow. Flow structures influence the interfacial temperature more effectively with increasing TAR and solid thickness compared with a constant temperature boundary condition. The change of channel flow structures with increasing convective instability is examined and the concomitant change of thermal patterns is quantified. Despite large differences in friction Reynolds and Richardson number between the DNS and atmospheric observations, similarities in the flow features were observed.
$\text {depth} = 0.1\text {--}10$ ), the solid thermal properties and thickness influence the fluid temperature only in the viscous or conduction region while the convective forcing influences the turbulent flow. Flow structures influence the interfacial temperature more effectively with increasing TAR and solid thickness compared with a constant temperature boundary condition. The change of channel flow structures with increasing convective instability is examined and the concomitant change of thermal patterns is quantified. Despite large differences in friction Reynolds and Richardson number between the DNS and atmospheric observations, similarities in the flow features were observed.
Information
- Type
- Papers
- Information
- Copyright
- © 2014 Cambridge University Press
Footnotes
Present address: NASA Ames Research Center, Moffett Field, CA 94035, USA.
References
- 16
- Cited by

