Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Weymouth, G. D.
and
Triantafyllou, M. S.
2012.
Global vorticity shedding for a shrinking cylinder.
Journal of Fluid Mechanics,
Vol. 702,
Issue. ,
p.
470.
Triantafyllou, M. S.
2012.
Survival hydrodynamics.
Journal of Fluid Mechanics,
Vol. 698,
Issue. ,
p.
1.
Weymouth, G. D.
and
Triantafyllou, M. S.
2013.
Ultra-fast escape of a deformable jet-propelled body.
Journal of Fluid Mechanics,
Vol. 721,
Issue. ,
p.
367.
Kriegseis, Jochen
Kinzel, Matthias
and
Rival, David E.
2013.
On the persistence of memory: do initial conditions impact vortex formation?.
Journal of Fluid Mechanics,
Vol. 736,
Issue. ,
p.
91.
Kriegseis, Jochen
Kinzel, Matthias
and
Rival, David
2013.
A Lagrangian Perspective on Vortex Formation for Unsteady Flat Plates.
Polet, Delyle T.
Christensen, Tyler
Rival, David E.
and
Weymouth, Gabriel
2014.
On the Effect of Rapid Area Change in Perching-Like Maneuvers.
Weymouth, G. D.
2014.
Chaotic rotation of a towed elliptical cylinder.
Journal of Fluid Mechanics,
Vol. 743,
Issue. ,
p.
385.
Maertens, Audrey P.
and
Triantafyllou, Michael S.
2014.
The boundary layer instability of a gliding fish helps rather than prevents object identification.
Journal of Fluid Mechanics,
Vol. 757,
Issue. ,
p.
179.
Polet, Delyle T.
Rival, David E.
and
Weymouth, Gabriel D.
2015.
Unsteady dynamics of rapid perching manoeuvres.
Journal of Fluid Mechanics,
Vol. 767,
Issue. ,
p.
323.
Maertens, Audrey P.
and
Weymouth, Gabriel D.
2015.
Accurate Cartesian-grid simulations of near-body flows at intermediate Reynolds numbers.
Computer Methods in Applied Mechanics and Engineering,
Vol. 283,
Issue. ,
p.
106.
Camassa, R.
Falqui, G.
Ortenzi, G.
and
Pedroni, M.
2015.
Topological effects on vorticity evolution in confined stratified fluids.
Journal of Fluid Mechanics,
Vol. 776,
Issue. ,
p.
109.
Steele, S. C.
Dahl, J. M.
Weymouth, G. D.
and
Triantafyllou, M. S.
2016.
Shape of retracting foils that model morphing bodies controls shed energy and wake structure.
Journal of Fluid Mechanics,
Vol. 805,
Issue. ,
p.
355.
Steele, S. C.
Weymouth, G. D.
Dahl, J. M.
and
Triantafyllou, M. S.
2016.
Advances in Fluid-Structure Interaction.
Vol. 133,
Issue. ,
p.
15.
Steele, S. C.
Weymouth, G. D.
and
Triantafyllou, M. S.
2017.
Added mass energy recovery of octopus-inspired shape change.
Journal of Fluid Mechanics,
Vol. 810,
Issue. ,
p.
155.
Maertens, Audrey P.
Gao, Amy
and
Triantafyllou, Michael S.
2017.
Optimal undulatory swimming for a single fish-like body and for a pair of interacting swimmers.
Journal of Fluid Mechanics,
Vol. 813,
Issue. ,
p.
301.
Gao, Amy
and
Triantafyllou, Michael S.
2018.
Independent caudal fin actuation enables high energy extraction and control in two-dimensional fish-like group swimming.
Journal of Fluid Mechanics,
Vol. 850,
Issue. ,
p.
304.
Chowdhury, Juhi
Smith, Cameron
and
Ringuette, Matthew J.
2020.
The Lift Force Produced by an Unsteady Translating Plate with a Rotating Tip.
Chowdhury, Juhi
and
Ringuette, Matthew J.
2021.
Effect of a Rotating and Swept Wingtip on Streamwise Gust Alleviation.
AIAA Journal,
Vol. 59,
Issue. 3,
p.
800.
Shah, Karan A.
and
Ringuette, Matthew J.
2024.
Unsteady Lift Produced by a Flat-Plate Wing Translating Past Finite Obstacles.
AIAA Journal,
Vol. 62,
Issue. 6,
p.
2222.
Adhikari, Dibya R.
and
Bhattacharya, Samik
2024.
Rapidly pitching plates in decelerating motion near the ground.
Journal of Fluid Mechanics,
Vol. 986,
Issue. ,
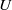 , and constant angle of attack, then rapidly pulled out of the fluid in the spanwise direction. Viewed within a fixed plane perpendicular to the span, the cross-sectional area of the foil seemingly disappears. The rapid spanwise motion results in the nearly instantaneous shedding of the boundary layer into the surrounding fluid. Particle image velocimetry measurements show that the shed layers quickly transition from free shear layers to form two strong, unequal-strength vortices, formed within non-dimensional time
, and constant angle of attack, then rapidly pulled out of the fluid in the spanwise direction. Viewed within a fixed plane perpendicular to the span, the cross-sectional area of the foil seemingly disappears. The rapid spanwise motion results in the nearly instantaneous shedding of the boundary layer into the surrounding fluid. Particle image velocimetry measurements show that the shed layers quickly transition from free shear layers to form two strong, unequal-strength vortices, formed within non-dimensional time 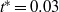 , based on the foil chord and forward velocity. These vortices are connected to, and interact with, the foil’s tip vortex through additional streamwise vorticity formed during the rapid pulling of the foil. Numerical simulations show that two strong spanwise vortices form from the shed vorticity of the boundary layer. The three-dimensional effects of the foil removal process are restricted to the tip of the foil. This method of vorticity transfer may be used for quickly introducing circulation to a fluid to provide forcing for biologically inspired flow control.
, based on the foil chord and forward velocity. These vortices are connected to, and interact with, the foil’s tip vortex through additional streamwise vorticity formed during the rapid pulling of the foil. Numerical simulations show that two strong spanwise vortices form from the shed vorticity of the boundary layer. The three-dimensional effects of the foil removal process are restricted to the tip of the foil. This method of vorticity transfer may be used for quickly introducing circulation to a fluid to provide forcing for biologically inspired flow control.