Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Daniel, Don
Riaz, Amir
and
Tchelepi, Hamdi A.
2015.
Onset of natural convection in layered aquifers.
Journal of Fluid Mechanics,
Vol. 767,
Issue. ,
p.
763.
Guerrero-Martínez, Fernando J.
Younger, Paul L.
Karimi, Nader
and
Kyriakis, Sotirios
2017.
Three-dimensional numerical simulations of free convection in a layered porous enclosure.
International Journal of Heat and Mass Transfer,
Vol. 106,
Issue. ,
p.
1005.
Nield, Donald A.
and
Bejan, Adrian
2017.
Convection in Porous Media.
p.
241.
Xu, Tibing
and
Jin, Yee-Chung
2018.
Simulation the convective mixing of CO2 in geological formations with a meshless model.
Chemical Engineering Science,
Vol. 192,
Issue. ,
p.
187.
Salibindla, Ashwanth K. R.
Subedi, Rabin
Shen, Victor C.
Masuk, Ashik U. M.
and
Ni, Rui
2018.
Dissolution-driven convection in a heterogeneous porous medium.
Journal of Fluid Mechanics,
Vol. 857,
Issue. ,
p.
61.
Soboleva, E.B.
2018.
Density-driven convection in an inhomogeneous geothermal reservoir.
International Journal of Heat and Mass Transfer,
Vol. 127,
Issue. ,
p.
784.
Holzbecher, Ekkehard
2019.
Convection pattern formation in a domain with a horizontal interface.
Physics of Fluids,
Vol. 31,
Issue. 5,
Hewitt, Duncan R.
Peng, Gunnar G.
and
Lister, John R.
2020.
Buoyancy-driven plumes in a layered porous medium.
Journal of Fluid Mechanics,
Vol. 883,
Issue. ,
Le Reun, Thomas
and
Hewitt, Duncan R.
2020.
Internally Heated Porous Convection: An Idealized Model for Enceladus' Hydrothermal Activity.
Journal of Geophysical Research: Planets,
Vol. 125,
Issue. 7,
Hewitt, D. R.
2020.
Vigorous convection in porous media.
Proceedings of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 476,
Issue. 2239,
p.
20200111.
Lasser, Jana
Ernst, Marcel
and
Goehring, Lucas
2021.
Stability and dynamics of convection in dry salt lakes.
Journal of Fluid Mechanics,
Vol. 917,
Issue. ,
Liu, Fanli
and
Wang, Moran
2022.
Phase diagram for preferential flow in dual permeable media.
Journal of Fluid Mechanics,
Vol. 948,
Issue. ,
Bedrikovetsky, Pavel
and
Borazjani, Sara
2022.
Exact Solutions for Gravity-Segregated Flows in Porous Media.
Mathematics,
Vol. 10,
Issue. 14,
p.
2455.
Zubova, N.A.
and
Lyubimova, T.P.
2022.
Nonlinear regimes of binary mixture convection in a two-layer porous medium of different configurations.
Computational Continuum Mechanics,
Vol. 15,
Issue. 2,
p.
145.
Hewitt, Duncan R.
2022.
Evolution of convection in a layered porous medium.
Journal of Fluid Mechanics,
Vol. 941,
Issue. ,
Soboleva, Elena
2023.
Instability Problems and Density-Driven Convection in Saturated Porous Media Linking to Hydrogeology: A Review.
Fluids,
Vol. 8,
Issue. 2,
p.
36.
Lasser, Jana
Nield, Joanna M.
Ernst, Marcel
Karius, Volker
Wiggs, Giles F. S.
Threadgold, Matthew R.
Beaume, Cédric
and
Goehring, Lucas
2023.
Salt Polygons and Porous Media Convection.
Physical Review X,
Vol. 13,
Issue. 1,
Zhang, Lianping
Luo, Gang
and
Wang, Shimin
2024.
The effects of fractures on porous flow and heat transfer in a reservoir of a U-shaped closed geothermal system.
Applied Thermal Engineering,
Vol. 246,
Issue. ,
p.
122852.
Cao, Yining
Niu, Weisheng
and
Wang, Xiaoming
2025.
Well-posedness and regularity of the darcy-boussinesq system in layered porous media.
Discrete and Continuous Dynamical Systems - B,
Vol. 30,
Issue. 12,
p.
4764.
Lyubimova, Tatyana
Shubenkov, Ivan
and
Ozhgibesova, Nadezhda
2025.
Soret‐Induced Convection in a Layered Porous Medium Simulating an Anticlinal Geological Fold Under the Action of a Geothermal Temperature Gradient.
Heat Transfer,
Vol. 54,
Issue. 3,
p.
2251.
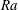 $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}\mathit{Ra}$ is investigated numerically in a two-dimensional porous medium that is heated at the lower boundary and cooled at the upper, and contains a thin, horizontal, low-permeability interior layer. In the limit that both the dimensionless thickness
$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}\mathit{Ra}$ is investigated numerically in a two-dimensional porous medium that is heated at the lower boundary and cooled at the upper, and contains a thin, horizontal, low-permeability interior layer. In the limit that both the dimensionless thickness 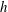 $h$ and permeability
$h$ and permeability  $\Pi $ of the low-permeability layer are small, the flow is described solely by the impedance of the layer
$\Pi $ of the low-permeability layer are small, the flow is described solely by the impedance of the layer 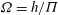 $\Omega = h/\Pi $ and by
$\Omega = h/\Pi $ and by 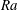 $\mathit{Ra}$ . In the limit
$\mathit{Ra}$ . In the limit 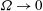 $\Omega \to 0$ (i.e.
$\Omega \to 0$ (i.e. 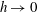 $h \to 0$ ), the system reduces to a homogeneous Rayleigh–Darcy (porous Rayleigh–Bénard) cell. Two notable features are observed as
$h \to 0$ ), the system reduces to a homogeneous Rayleigh–Darcy (porous Rayleigh–Bénard) cell. Two notable features are observed as 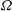 $\Omega $ is increased: the dominant horizontal length scale of the flow increases; and the heat flux, as measured by the Nusselt number
$\Omega $ is increased: the dominant horizontal length scale of the flow increases; and the heat flux, as measured by the Nusselt number 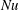 $\mathit{Nu}$ , can increase. For larger values of
$\mathit{Nu}$ , can increase. For larger values of 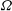 $\Omega $ ,
$\Omega $ , 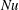 $\mathit{Nu}$ always decreases. The dependence of the flow on
$\mathit{Nu}$ always decreases. The dependence of the flow on 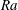 $\mathit{Ra}$ is explored, over the range
$\mathit{Ra}$ is explored, over the range 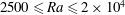 $2500 \leqslant \mathit{Ra} \leqslant 2\times 10^4$ . Simple one-dimensional models are developed to describe some of the observed features of the relationship
$2500 \leqslant \mathit{Ra} \leqslant 2\times 10^4$ . Simple one-dimensional models are developed to describe some of the observed features of the relationship 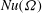 $\mathit{Nu}(\Omega )$ .
$\mathit{Nu}(\Omega )$ .
