Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Haines, P. E.
Hewitt, R. E.
and
Hazel, A. L.
2011.
The Jeffery–Hamel similarity solution and its relation to flow in a diverging channel.
Journal of Fluid Mechanics,
Vol. 687,
Issue. ,
p.
404.
Peixinho, Jorge
and
Besnard, Hugues
2013.
Transition to turbulence in slowly divergent pipe flow.
Physics of Fluids,
Vol. 25,
Issue. 11,
Dijkstra, Henk A.
Wubs, Fred W.
Cliffe, Andrew K.
Doedel, Eusebius
Dragomirescu, Ioana F.
Eckhardt, Bruno
Gelfgat, Alexander Yu.
Hazel, Andrew L.
Lucarini, Valerio
Salinger, Andy G.
Phipps, Erik T.
Sanchez-Umbria, Juan
Schuttelaars, Henk
Tuckerman, Laurette S.
and
Thiele, Uwe
2014.
Numerical Bifurcation Methods and their Application to Fluid Dynamics: Analysis beyond Simulation.
Communications in Computational Physics,
Vol. 15,
Issue. 1,
p.
1.
FUJIKAWA, Toshihide
EGASHIRA, Ryu
FUJIKAWA, Shigeo
TAKEDA, Kazu
and
KODAMA, Tetsuya
2016.
Extended Bernoulli equation, friction loss, and friction coefficient for microscopic Jeffery-Hamel flow with small Reynolds number up to O(1).
Journal of Fluid Science and Technology,
Vol. 11,
Issue. 3,
p.
JFST0019.
Khan, Najeeb Alam
Sultan, Faqiha
Shaikh, Amber
Ara, Asmat
and
Rubbab, Qammar
2016.
Haar wavelet solution of the MHD Jeffery-Hamel flow and heat transfer in Eyring-Powell fluid.
AIP Advances,
Vol. 6,
Issue. 11,
Matyas, Alpar
and
Nagy, Botond
2017.
Numerical study of bipolar coordinate Jeffery-Hamel flow.
Vol. 1863,
Issue. ,
p.
560077.
Jotkar, Mamta R.
and
Govindarajan, Rama
2017.
Non-modal stability of Jeffery-Hamel flow.
Physics of Fluids,
Vol. 29,
Issue. 6,
Egashira, R
Fujikawa, T
Yaguchi, H
and
Fujikawa, S
2018.
Microscopic and low Reynolds number flows between two intersecting permeable walls.
Fluid Dynamics Research,
Vol. 50,
Issue. 3,
p.
035502.
Peterson, John W.
Lindsay, Alexander D.
and
Kong, Fande
2018.
Overview of the incompressible Navier–Stokes simulation capabilities in the MOOSE framework.
Advances in Engineering Software,
Vol. 119,
Issue. ,
p.
68.
Parker, J. P.
Caulfield, C. P.
and
Kerswell, R. R.
2019.
Kelvin–Helmholtz billows above Richardson number .
Journal of Fluid Mechanics,
Vol. 879,
Issue. ,
Kant, Ravi
and
Vinod, Narayanan
2019.
Control of optimal growth of instabilities in Jeffery-Hamel flow.
AIP Advances,
Vol. 9,
Issue. 3,
Egashira, R
Fujikawa, T
Yaguchi, H
and
Fujikawa, S
2019.
Low Reynolds number flows in a microscopic and tapered tube with a permeability.
Fluid Dynamics Research,
Vol. 51,
Issue. 2,
p.
025504.
Fedyushkin, Alexey I.
Puntus, Artur A.
and
Volkov, Evgeny V.
2019.
Symmetry of the flows of Newtonian and non-Newtonian fluids in the diverging and converging plane channels.
Vol. 2181,
Issue. ,
p.
020016.
Vittal Shenoy, Dhanush
Safdari Shadloo, Mostafa
Peixinho, Jorge
and
Hadjadj, Abdellah
2020.
Direct numerical simulations of laminar and transitional flows in diverging pipes.
International Journal of Numerical Methods for Heat & Fluid Flow,
Vol. 30,
Issue. 1,
p.
75.
Banerjee, Astick
Mahato, Sanat Kumar
Bhattacharyya, Krishnendu
and
Chamkha, Ali J.
2021.
Divergent channel flow of Casson fluid and heat transfer with suction/blowing and viscous dissipation: Existence of boundary layer.
Partial Differential Equations in Applied Mathematics,
Vol. 4,
Issue. ,
p.
100172.
Kant, Ravi
SHARMA, VISHAL
BHORANIYA, RAMESH
and
Vinod, Narayanan
2022.
Receptivity and sensitivity analysis of Jeffery-Hamel flow.
Sādhanā,
Vol. 47,
Issue. 3,
Rehman, Sohail
Almubaddel, Fahad S.
Mahrous, Y.M.
Alsadoun, Fares A.
Abouzied, Amr S.
and
Hashim
2023.
A generalization of Jeffrey-Hamel problem to Reiner-Rivlin model for energy and thermodynamic analysis using Keller-Box computational framework.
Case Studies in Thermal Engineering,
Vol. 50,
Issue. ,
p.
103462.
Choudhary, Sushila
Choudhary, Prasun
Pattanaik, Balachandra
and
Shekar, Saranya
2023.
Tangent Hyperbolic Fluid Flow under Condition of Divergent Channel in the Presence of Porous Medium with Suction/Blowing and Heat Source: Emergence of the Boundary Layer.
International Journal of Mathematics and Mathematical Sciences,
Vol. 2023,
Issue. 1,
Rezaee, Danial
2024.
Linear temporal stability of Jeffery–Hamel flow of nanofluids.
European Journal of Mechanics - B/Fluids,
Vol. 107,
Issue. ,
p.
1.
Mohana, C.M.
Kumar, B. Rushi
Nagarathnam, Sunitha
and
Shivakumara, I.S.
2024.
Thermal performance analysis of magnetohydrodynamic Al2O3-SiO2-TiO2/water ternary hybrid nanofluid in converging and diverging channels with nanoparticle shape effects.
Case Studies in Thermal Engineering,
Vol. 64,
Issue. ,
p.
105429.
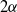 , bounded by circular arcs at the inlet/outlet and for a net radial outflow of fluid. In particular, we show that in a finite domain there is a sequence of nested neutral curves in the
, bounded by circular arcs at the inlet/outlet and for a net radial outflow of fluid. In particular, we show that in a finite domain there is a sequence of nested neutral curves in the 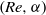 plane, each corresponding to a midplane symmetry-breaking (pitchfork) bifurcation, where
plane, each corresponding to a midplane symmetry-breaking (pitchfork) bifurcation, where 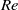 is a Reynolds number based on the radial mass flux. For small wedge angles we demonstrate that the first pitchfork bifurcation in the finite domain occurs at a critical Reynolds number that is in agreement with the only pitchfork bifurcation in the infinite-domain similarity solution, but that the criticality of the bifurcation differs (in general). We explain this apparent contradiction by demonstrating that, for
is a Reynolds number based on the radial mass flux. For small wedge angles we demonstrate that the first pitchfork bifurcation in the finite domain occurs at a critical Reynolds number that is in agreement with the only pitchfork bifurcation in the infinite-domain similarity solution, but that the criticality of the bifurcation differs (in general). We explain this apparent contradiction by demonstrating that, for 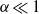 , superposition of two (infinite-domain) eigenmodes can be used to construct a leading-order finite-domain eigenmode. These constructed modes accurately predict the multiple symmetry-breaking bifurcations of the finite-domain flow without recourse to computation of the full field equations. Our computational results also indicate that temporally stable, isolated, steady solutions may exist. These states are finite-domain analogues of the steady waves recently presented by Kerswell, Tutty, & Drazin (J. Fluid Mech., vol. 501, 2004, pp. 231–250) for an infinite domain. Moreover, we demonstrate that there is non-uniqueness of stable solutions in certain parameter regimes. Our numerical results tie together, in a consistent framework, the disparate results in the existing literature.
, superposition of two (infinite-domain) eigenmodes can be used to construct a leading-order finite-domain eigenmode. These constructed modes accurately predict the multiple symmetry-breaking bifurcations of the finite-domain flow without recourse to computation of the full field equations. Our computational results also indicate that temporally stable, isolated, steady solutions may exist. These states are finite-domain analogues of the steady waves recently presented by Kerswell, Tutty, & Drazin (J. Fluid Mech., vol. 501, 2004, pp. 231–250) for an infinite domain. Moreover, we demonstrate that there is non-uniqueness of stable solutions in certain parameter regimes. Our numerical results tie together, in a consistent framework, the disparate results in the existing literature.
