Hostname: page-component-76fb5796d-dfsvx Total loading time: 0 Render date: 2024-04-26T19:24:53.345Z Has data issue: false hasContentIssue false
  • English
  • Français

A kinetic-based hyperbolic two-fluid model for binary hard-sphere mixtures

Published online by Cambridge University Press:  19 August 2019

Rodney O. Fox Open the ORCID record for Rodney O. Fox [Opens in a new window]
Rodney O. Fox*
Affiliation:
Department of Chemical and Biological Engineering, 618 Bissell Road, Iowa State University, Ames, IA 50011-1098, USA Center for Multiphase Flow Research and Education, Iowa State University, Ames, IA 50011-1096, USA
*
†Email address for correspondence: rofox@iastate.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

Starting from coupled Boltzmann–Enskog (BE) kinetic equations for a two-particle system consisting of hard spheres, a hyperbolic two-fluid model for binary, hard-sphere mixtures is derived with separate mean velocities and energies for each phase. In addition to spatial transport, the BE kinetic equations account for particle–particle collisions, using an elastic hard-sphere collision model, and the Archimedes (buoyancy) force due to spatial gradients of the pressure in each phase, as well as other forces involving spatial gradients (e.g. lift). In the derivation, the particles in a given phase have identical mass and volume, and have no internal degrees of freedom (i.e. the particles are adiabatic). The ‘hard-sphere-fluid’ phase is obtained in the limit where the particle diameter in one phase tends to zero with fixed phase density so that the number of fluid particles tends to infinity. The moment system resulting from the two BE kinetic equations is closed at second order by invoking the anisotropic Gaussian closure. The resulting two-fluid model for a binary, hard-sphere mixture therefore consists (for each phase 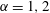$\unicode[STIX]{x1D6FC}=1,2$) of transport equations for the mass 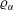$\unicode[STIX]{x1D71A}_{\unicode[STIX]{x1D6FC}}$, mean momentum 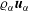$\unicode[STIX]{x1D71A}_{\unicode[STIX]{x1D6FC}}\boldsymbol{u}_{\unicode[STIX]{x1D6FC}}$ (where 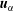$\boldsymbol{u}_{\unicode[STIX]{x1D6FC}}$ is the velocity) and a symmetric, second-order, kinetic energy tensor 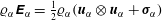$\unicode[STIX]{x1D71A}_{\unicode[STIX]{x1D6FC}}\unicode[STIX]{x1D640}_{\unicode[STIX]{x1D6FC}}=\frac{1}{2}\unicode[STIX]{x1D71A}_{\unicode[STIX]{x1D6FC}}(\boldsymbol{u}_{\unicode[STIX]{x1D6FC}}\otimes \boldsymbol{u}_{\unicode[STIX]{x1D6FC}}+\unicode[STIX]{x1D748}_{\unicode[STIX]{x1D6FC}})$. The trace of the fluctuating energy tensor $\unicode[STIX]{x1D748}_{\unicode[STIX]{x1D6FC}}$ is 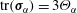$\text{tr}(\unicode[STIX]{x1D748}_{\unicode[STIX]{x1D6FC}})=3\unicode[STIX]{x1D6E9}_{\unicode[STIX]{x1D6FC}}$ where 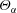$\unicode[STIX]{x1D6E9}_{\unicode[STIX]{x1D6FC}}$ is the phase temperature (or granular temperature). Thus, 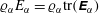$\unicode[STIX]{x1D71A}_{\unicode[STIX]{x1D6FC}}E_{\unicode[STIX]{x1D6FC}}=\unicode[STIX]{x1D71A}_{\unicode[STIX]{x1D6FC}}\text{tr}(\unicode[STIX]{x1D640}_{\unicode[STIX]{x1D6FC}})$ is the total kinetic energy, the sum over $\unicode[STIX]{x1D6FC}$ of which is the total kinetic energy of the system, a conserved quantity. From the analysis, it is found that the BE finite-size correction leads to exact phase pressure (or stress) tensors that depend on the mean-slip velocity 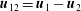$\boldsymbol{u}_{12}=\boldsymbol{u}_{1}-\boldsymbol{u}_{2}$, as well as the phase temperatures for both phases. These pressure tensors also appear in the momentum-exchange terms in the mean momentum equations that produce the Archimedes force, as well as drag contributions due to fluid compressibility and a lift force due to mean fluid-velocity gradients. The closed BE energy flux tensors show a similar dependence on the mean-slip velocity. The characteristic polynomial of the flux matrix from the one-dimensional model is computed symbolically and depends on five parameters: the particle volume fractions 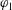$\unicode[STIX]{x1D711}_{1}$, 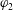$\unicode[STIX]{x1D711}_{2}$, the phase density ratio 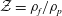${\mathcal{Z}}=\unicode[STIX]{x1D70C}_{f}/\unicode[STIX]{x1D70C}_{p}$, the phase temperature ratio 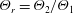$\unicode[STIX]{x1D6E9}_{r}=\unicode[STIX]{x1D6E9}_{2}/\unicode[STIX]{x1D6E9}_{1}$ and the mean-slip Mach number 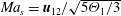$Ma_{s}=\boldsymbol{u}_{12}/\sqrt{5\unicode[STIX]{x1D6E9}_{1}/3}$. By applying Sturm’s Theorem to the characteristic polynomial, it is demonstrated that the model is hyperbolic over a wide range of these parameters, in particular, for the physically most relevant values.

JFM classification

Multiphase and Particle-laden Flows: Particle/fluid flow Rarefied Gas Flow: Kinetic theory
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 877 , 25 October 2019 , pp. 282 - 329
Copyright
© 2019 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Agrawal, K., Loezos, P. N., Syamlal, M. & Sundaresan, S. 2001 The role of meso-scale structures in rapid gas–solid flows. J. Fluid Mech. 445, 151–185.10.1017/S0022112001005663Google Scholar
Andries, P., Aoki, K. & Perthame, B. 2002 A consistent BGK-type model for gas mixtures. J. Stat. Phys. 106 (5/6), 993–1018.10.1023/A:1014033703134Google Scholar
Barajas, L., Garcia-Colin, L. S. & Pinã, E. 1973 On the Enskog–Thorne theory for a binary mixture of dissimilar rigid spheres. J. Stat. Phys. 7 (2), 161–183.10.1007/BF01024213Google Scholar
Blanc, F., Lemaire, É, Muenier, A. & Peters, F. 2013 Microstructure in sheared non-Brownian concentrated suspensions. J. Rheol. 57, 273–292.10.1122/1.4766597Google Scholar
Buyevich, Y. A. & Shchelchkova, I. N. 1978 Flow of dense suspensions. Prog. Aerosp. Sci. 18, 121–150.10.1016/0376-0421(77)90004-5Google Scholar
Capecelatro, J. & Desjardins, O. 2013 An Euler–Lagrange strategy for simulating particle-laden flows. J. Comput. Phys. 238, 1–31.10.1016/j.jcp.2012.12.015Google Scholar
Capecelatro, J., Desjardins, O. & Fox, R. O. 2015 On fluid–particle dynamics in fully developed cluster-induced turbulence. J. Fluid Mech. 780, 578–635.10.1017/jfm.2015.459Google Scholar
Capecelatro, J., Desjardins, O. & Fox, R. O. 2016a Strongly coupled fluid–particle flows in vertical channels. I. Reynolds-averaged two-phase turbulence statistics. Phys. Fluids 28, 033306.Google Scholar
Capecelatro, J., Desjardins, O. & Fox, R. O. 2016b Strongly coupled fluid–particle flows in vertical channels. II. Turbulence modeling. Phys. Fluids 28, 033307.Google Scholar
Capecelatro, J., Desjardins, O. & Fox, R. O. 2018 On the transition between turbulence regimes in particle-laden channel flows. J. Fluid Mech. 845, 499–519.10.1017/jfm.2018.259Google Scholar
Carnahan, N. F. & Starling, K. E. 1969 Equation of state for nonattracting rigid spheres. J. Chem. Phys. 51, 635–636.10.1063/1.1672048Google Scholar
Cercignani, C. 1988 The Boltzmann Equation and Its Applications. Springer.10.1007/978-1-4612-1039-9Google Scholar
Chalons, C., Fox, R. O., Laurent, F., Massot, M. & Vié, A. 2017 Multivariate Gaussian extended quadrature method of moments for turbulent fluid–particle flows. Multiscale Model. Simul. 15 (4), 1553–1583.10.1137/16M109209XGoogle Scholar
Chapman, S. 1912 Kinetic theory of a gas constituted of spherically symmetrical molecules. Phil. Trans. R. Soc. A 211, 433–483.10.1098/rsta.1912.0012Google Scholar
Chapman, S. & Cowling, T. G. 1952 The Mathematical Theory of Non-Uniform Gases, 2nd edn. Cambridge University Press.Google Scholar
Chliamovitch, C., Malaspinas, O. & Chopard, B. 2017 Kinetic theory beyond the Stosszahlansatz. Entropy 19, 381.10.3390/e19080381Google Scholar
Chu, S. & Prosperetti, A. 2016 On flux terms in volume averaging. Intl J. Multiphase Flow 80, 176–180.10.1016/j.ijmultiphaseflow.2015.12.009Google Scholar
Cravath, A. M. 1930 The rate at which ions lose energy in elastic collisions. Phys. Rev. 36, 248–250.10.1103/PhysRev.36.248Google Scholar
Desjardins, O., Fox, R. O. & Villedieu, P. 2008 A quadrature-based moment method for dilute fluid–particle flows. J. Comput. Phys. 227 (4), 2514–2539.10.1016/j.jcp.2007.10.026Google Scholar
Drew, P. A. & Passman, S. L. 1998 Theory of Multicomponent Fluids. Springer.Google Scholar
Enskog, D. 1911a Bemerkungen zu einer Fundamentalgleichung in der kinetishen Gastheorie. Phys. Z. 12, 533–539.Google Scholar
Enskog, D. 1911b Über eine Verallgemeinerung de zweiten Maxwellschen Theorie der Gase. Phys. Z. 12, 56–60.Google Scholar
Février, P., Simonin, O. & Squires, K. D. 2005 Partitioning of particle velocities in gas–solid turbulent flow into a continuous field and a spatially uncorrelated random distribution: theoretical formalism and numerical study. J. Fluid Mech. 533, 1–46.10.1017/S0022112005004088Google Scholar
Fox, R. O. 2008 A quadrature-based third-order moment method for dilute gas–particle flow. J. Comput. Phys. 227 (12), 6313–6350.10.1016/j.jcp.2008.03.014Google Scholar
Fox, R. O. 2009 Higher-order quadrature-based moment methods for kinetic equations. J. Comput. Phys. 228, 7771–7791.10.1016/j.jcp.2009.07.018Google Scholar
Fox, R. O. 2012 Large-eddy-simulation tools for multiphase flows. Annu. Rev. Fluid Mech. 44, 47–76.10.1146/annurev-fluid-120710-101118Google Scholar
Fox, R. O. 2014 On multiphase turbulence models for collisional fluid–particle flows. J. Fluid Mech. 742, 368–424.10.1017/jfm.2014.21Google Scholar
Fox, R. O., Laurent, F. & Massot, M. 2008 Numerical simulation of spray coalescence in an Eulerian framework: direct quadrature method of moments and multi-fluid method. J. Comput. Phys. 227, 3058–3088.10.1016/j.jcp.2007.10.028Google Scholar
Fox, R. O., Laurent, F. & Vié, A. 2018 Conditional hyperbolic quadrature method of moments for kinetic equations. J. Comput. Phys. 365, 269–293.10.1016/j.jcp.2018.03.025Google Scholar
Fox, R. O. & Vedula, P. 2010 Quadrature-based moment method for moderately dense polydisperse gas–particle flows. Indust. Engng Chem. Res. 49, 5174–5187.10.1021/ie9013138Google Scholar
Garzó, V., Dufty, J. W. & Hrenya, C. M. 2007 Enskog theory for polydisperse granular mixtures. Part 1. Navier–Stokes order transport. Phys. Rev. E 76, 031303.Google Scholar
Garzó, V., Santos, A. & Brey, J. J. 1989 A kinetic model for a multicomponent gas. Phys. Fluids A 1, 380–383.10.1063/1.857458Google Scholar
Garzó, V., Tenneti, S., Subramaniam, S. & Hrenya, C. M. 2012 Enskog kinetic theory for monodisperse gas–solid flows. J. Fluid Mech. 712, 129–168.10.1017/jfm.2012.404Google Scholar
Goldman, E. & Sirovich, L. 1967 Equations for gas mixtures. Phys. Fluids 10 (9), 1928–1940.10.1063/1.1762389Google Scholar
Goldman, E. & Sirovich, L. 1969 Equations for gas mixtures. II. Phys. Fluids 12 (1), 245–247.10.1063/1.1692276Google Scholar
Grad, H. 1960 Theory of rarefied gases. In Rarefied Gas Dynamics (ed. Devienne, F.). Pergamon Press.Google Scholar
Gross, E. P. & Krook, M. 1956 Model for collision processes in gases: small-amplitude oscillations of charged two-component systems. Phys. Rev. 102 (3), 593–604.10.1103/PhysRev.102.593Google Scholar
Guazzelli, É. & Pouliquen, O. 2018 Rheology of dense granular suspensions. J. Fluid Mech. 852, P1-1-73.10.1017/jfm.2018.548Google Scholar
Hamel, B. B. 1965 Kinetic model for binary gas mixtures. Phys. Fluids 8 (1), 418–425.10.1063/1.1761239Google Scholar
Hamel, B. B. 1966 Two-fluid hydrodynamic equations for a neutral, disparate-mass, binary mixture. Phys. Fluids 9 (12), 12–22.10.1063/1.1761507Google Scholar
Hank, S., Saurel, R. & Le Metayer, O. 2011 A hyperbolic Eulerian model for dilute two-phase suspensions. J. Modern Phys. 2, 997–1011.10.4236/jmp.2011.29120Google Scholar
Hansen-Goos, H. & Roth, R. 2006 A new generalization of the Carnahan–Starling equation of state to additive mixtures of hard spheres. J. Chem. Phys. 124, 154506.Google Scholar
Houim, R. W. & Oran, E. S. 2016 A multiphase model for compressible granular–gasous flows: Formulation and initial tests. J. Fluid Mech. 789, 166–220.10.1017/jfm.2015.728Google Scholar
Jackson, R. 1997 Locally averaged equations of motion for a mixture of identical spherical particles and a Newtonian fluid. Chem. Engng Sci. 52, 2457–2469.10.1016/S0009-2509(97)00065-1Google Scholar
Jenkins, J. T. & Savage, S. B. 1983 A theory for the rapid flow of identical, smooth, nearly elastic, spherical particles. J. Fluid Mech. 130, 187–202.10.1017/S0022112083001044Google Scholar
Kolodner, I.1957 Moment description of gas mixtures, I. Tech. Rep. NYU–7980. Courant Institute of Mathematical Sciences, New York University.Google Scholar
Kong, B. & Fox, R. O. 2017 A solution algorithm for fluid–particle flows across all flow regimes. J. Comput. Phys. 344, 575–594.10.1016/j.jcp.2017.05.013Google Scholar
Kong, B. & Fox, R. O. 2019 A moment-based kinetic theory model for polydisperse gas–particle flows. Powder Technol; doi:10.1016/j.powtec.2019.04.031.Google Scholar
Kong, B., Fox, R. O., Feng, H., Capecelatro, J., Patel, R. & Desjardins, O. 2017 Euler–Euler anisotropic Gaussian mesoscale simulation of homogeneous cluster-induced gas–particle turbulence. AIChE J. 63 (7), 2630–2643.10.1002/aic.15686Google Scholar
Kumbaro, A. & Ndjinga, M. 2011 Influence of interfacial pressure term on the hyperbolicity of a general multifluid model. J. Comput. Multiphase Flows 3 (3), 177–195.10.1260/1757-482X.3.3.177Google Scholar
Levermore, C. D. 1996 Moment closure hierarchies for kinetic theories. J. Stat. Phys. 83 (5/6), 1021–1065.10.1007/BF02179552Google Scholar
Levermore, C. D. & Morokoff, W. 1996 The Gaussian moment closure for gas dynamics. SIAM J. Appl. Math. 59, 72–96.10.1137/S0036139996299236Google Scholar
Lhuillier, D., Chang, C.-H. & Theofanous, T. G. 2013 On the quest for a hyperbolic effective-field model of disperse flows. J. Fluid Mech. 731, 184–194.10.1017/jfm.2013.380Google Scholar
Lhuillier, D. & Theofanous, T. G. 2010 Multiphase flows: compressible multi-hydrodynamics Part 1: effective field formulation of multiphase flows. In Handbook of Nuclear Engineering (ed. Cacuci, D. G.), pp. 1813–1857. Springer.10.1007/978-0-387-98149-9_16Google Scholar
Ling, Y., Wagner, J. L., Beresh, S. J., Kearney, S. P. & Balachandar, S. 2012 Interaction of a planar shock wave with a dense particle curtin: modeling and experiments. Phys. Fluids 24, 113301.10.1063/1.4768815Google Scholar
Marble, F. E. 1970 Dynamics of dusty gases. Annu. Rev. Fluid Mech. 2 (1), 397–446.10.1146/annurev.fl.02.010170.002145Google Scholar
Marchisio, D. L. & Fox, R. O. 2013 Computational Models for Polydisperse Particulate and Multiphase Systems. Cambridge University Press.10.1017/CBO9781139016599Google Scholar
Maxey, M. 2017 Simulation methods for particulate flows and concentrated suspensions. Annu. Rev. Fluid Mech. 49, 171–193.10.1146/annurev-fluid-122414-034408Google Scholar
McGrath II, T. P., St. Clair, J. G. & Balachandar, S. 2016 A compressible two-phase model for dispersed particle flows with application from dense to dilute regimes. J. Appl. Phys. 119 (17), 174903.10.1063/1.4948301Google Scholar
Mehrabadi, M., Tenneti, S., Garg, R. & Subramaniam, S. 2015 Pseudo-turbulent gas-phase velocity fluctuations in homogeneous gas–solid flow: fixed particle assemblies and freely evolving suspensions. J. Fluid Mech. 770, 210–246.10.1017/jfm.2015.146Google Scholar
Mehta, Y., Jackson, T. L. & Balachandar, S. 2019 Pseudo-turbulence in inviscid simulations of shock interacting with a bed of randomly distributed particles. Shock Waves doi:10.1007/s00193-019-00905-3.Google Scholar
Métivier, G. 2005 Remarks on the well-posedness of the nonlinear Cauchy problem. In Contemporary Mathematics, Geometric Analysis of PDE and Several Complex Variables, vol. 368, pp. 337–356. American Mathematical Society.10.1090/conm/368/06790Google Scholar
Minier, J.-P. 2015 On Lagrangian stochastic methods for turbulent polydisperse two-phase reactive flows. Prog. Energy Combust. Sci. 50, 1–62.10.1016/j.pecs.2015.02.003Google Scholar
Morse, T. F. 1963 Energy and momentum exchange between nonequipartition gases. Phys. Fluids 6 (10), 1420–1427.10.1063/1.1710963Google Scholar
Morse, T. F. 1964 Kinetic model equations for a gas mixture. Phys. Fluids 7 (12), 2012–2013.10.1063/1.1711112Google Scholar
Ndjinga, M. 2007 Influence of interfacial pressure on the hyperbolicity of the two-fluid model. C. R. Acad. Sci. Paris 1 344, 407–412.10.1016/j.crma.2007.02.006Google Scholar
Nigmatulin, R. I. 1979 Spatial averaging in the mechanics of heterogeneous and dispersed systems. Intl J. Multiphase Flow 5, 353–385.10.1016/0301-9322(79)90013-2Google Scholar
Osnes, A. N., Vartdal, M., Omang, M. G. & Reif, B. A. P. 2019 Computational analysis of shock-induced flow through stationary particle clouds. Intl J. Multiphase Flow 114, 268–286.10.1016/j.ijmultiphaseflow.2019.03.010Google Scholar
Panicker, N., Passalacqua, A. & Fox, R. O. 2018 On the hyperbolicity of the two-fluid model for gas–liquid flows. Appl. Math. Model. 57, 432–447.10.1016/j.apm.2018.01.011Google Scholar
Patel, R., Desjardins, O., Kong, B., Capecelatro, J. & Fox, R. O. 2017 Verification of Eulerian–Eulerian and Eulerian–Lagrangian simulations for turbulent fluid–particle flow. AIChE J. 63 (12), 5396–5412.10.1002/aic.15949Google Scholar
Patel, R. G., Desjardins, O. & Fox, R. O. 2019 Three-dimensional conditional hyperbolic quadrature method of moments. J. Comput. Phys. X 1, 100006.Google Scholar
Risso, F. 2018 Agitation, mixing, and transfers induced by bubbles. Annu. Rev. Fluid Mech. 50, 25–48.10.1146/annurev-fluid-122316-045003Google Scholar
Santos, A., Yuste, S. B. & López de Haro, M. 1999 Equation of state of a multicomponent d-dimensional hard-sphere fluid. Mol. Phys. 96, 1–5.Google Scholar
Saurel, R. & Abgrall, R. 1999 A multiphase Godunov method for compressible multifluid and multiphase flows. J. Comput. Phys. 150 (2), 425–467.10.1006/jcph.1999.6187Google Scholar
Saurel, R., Chinnayya, A. & Carmouze, Q. 2017 Modelling compressible dense and dilute two-phase flows. Phys. Fluids 29, 063301.10.1063/1.4985289Google Scholar
Shallcross, G. S., Fox, R. O. & Capecelatro, J. 2019 A volume-filtered description of compressible particle-laden flows. Intl J. Multiphase Flow (submitted).Google Scholar
Sinclair, J. & Jackson, R. 1989 Gas–particle flow in a vertical pipe with particle–particle interactions. AIChE J. 35 (9), 1473–1486.10.1002/aic.690350908Google Scholar
Sirovich, L. 1962 Kinetic modeling of gas mixtures. Phys. Fluids 5 (8), 908–918.10.1063/1.1706706Google Scholar
Sirovich, L. 1963 Errata: kinetic modeling of gas mixtures. Phys. Fluids 6, 598.10.1063/1.1706785Google Scholar
Struchtrup, H. 2005 Macroscopic Transport Equations for Rarefied Gas Flows. Springer.10.1007/3-540-32386-4Google Scholar
Sturm, J. C. F. 1829 Mémoire sur la résolution des équations numériques. Bull. Sci. Férussac 11, 419–425.Google Scholar
Syamlal, M. 2011 A hyperbolic model for fluid–solids two-phase flow. Chem. Engng Sci. 66, 4421–4425.10.1016/j.ces.2011.02.051Google Scholar
Tavenashad, V., Passalacqua, A., Fox, R. O. & Subramaniam, S. 2019 Effect of density ratio on velocity fluctuations in dispersed multiphase flow from simulations of finite-size particles. Acta Mech. 230 (2), 469–484.10.1007/s00707-018-2267-3Google Scholar
Tenneti, S. & Subramaniam, S. 2014 Particle-resolved direct numerical simulation for gas–solid flow model development. Annu. Rev. Fluid Mech. 46, 199–230.10.1146/annurev-fluid-010313-141344Google Scholar
Tham, M. K. & Gubbins, K. E. 1971 Kinetic theory of multicomponent dense fluid mixtures of rigid spheres. J. Chem. Phys. 55, 268–279.10.1063/1.1675518Google Scholar
Theofanous, T. G. & Chang, C.-H. 2017 The dynamics of dense particle clouds subjected to shock waves. Part 2. Modeling/numerical issues and the way forward. Intl J. Multiphase Flow 89, 177–206.10.1016/j.ijmultiphaseflow.2016.10.004Google Scholar
Theofanous, T. G., Mitkin, V. & Chang, C.-H. 2016 The dynamics of dense particle clouds subjected to shock waves. Part I: experiments and scaling laws. J. Fluid Mech. 792, 655–681.10.1017/jfm.2016.97Google Scholar
Theofanous, T. G., Mitkin, V. & Chang, C.-H. 2018 Shock dispersal of dilute particle clouds. J. Fluid Mech. 841, 732–745.10.1017/jfm.2018.110Google Scholar
Torrilhon, M. 2016 Modeling nonequilibrium gas flow based on moment equations. Annu. Rev. Fluid Mech. 48, 429–458.10.1146/annurev-fluid-122414-034259Google Scholar
Vazquez-Gonzalez, T., Llor, A. & Fochesato, C. 2016 Ransom test results from various two-fluid schemes: Is enforcing hyperbolicity a thermodynamically consistent option? Intl J. Multiphase Flow 81, 104–112.10.1016/j.ijmultiphaseflow.2015.12.007Google Scholar
Vié, A., Chalons, C., Fox, R. O., Laurent, F. & Massot, M. 2011 A multi-Gaussian quadrature method of moments for simulating high-Stokes-number turbulent two-phase flows. In Annu. Res. Briefs CTR, pp. 309–320.Google Scholar
Vié, A., Doisneau, F. & Massot, M. 2015 On the anisotropic Gaussian velocity closure for inertial-particle laden flows. Commun. Comput. Phys. 17 (01), 1–46.10.4208/cicp.021213.140514aGoogle Scholar
Wagner, J. L., Beresh, S. J., Kearney, S. P., Trott, W. M., Castaneda, J. N., Pruett, B. O. & Bear, M. R. 2012 A multiphase shock tube for shock wave interactions with dense particle fields. Exp. Fluids 52, 1507–1517.10.1007/s00348-012-1272-xGoogle Scholar
Zhang, D. Z., Ma, X. & Rauenzahn, R. M. 2006 Interspecies stress in momentum equations for dense binary particulate systems. Phys. Rev. Lett. 97 (4), 048301.10.1103/PhysRevLett.97.048301Google Scholar
Zhang, D. Z. & Prosperetti, A. 1994 Averaged equations for inviscid dispersed two-phase flows. J. Fluid Mech. 267, 185–219.10.1017/S0022112094001151Google Scholar