Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Shi, Xiao-Feng
Ma, Dong-Jun
Ma, Zong-Qiang
Sun, De-Jun
and
Wang, Pei
2021.
Multiple solutions and hysteresis in the flows driven by surface with antisymmetric velocity profile*
.
Chinese Physics B,
Vol. 30,
Issue. 9,
p.
090201.
Sarwar, Wasim
Mellibovsky, Fernando
Alam, Md. Mahbub
and
Zafar, Farhan
2021.
Transition to Periodic Behaviour of Flow Past a Circular Cylinder under the Action of Fluidic Actuation in the Transitional Regime.
Energies,
Vol. 14,
Issue. 16,
p.
5069.
Sarwar, Wasim
Bergadà, Josep M.
and
Mellibovsky, Fernando
2021.
Onset of temporal dynamics within a low reynolds-number laminar fluidic oscillator.
Applied Mathematical Modelling,
Vol. 95,
Issue. ,
p.
219.
El Mansy, R.
Sarwar, W.
Bergadà, J.M.
and
Mellibovsky, F.
2022.
Square cylinder in the interface of two different velocity streams.
Journal of Fluid Mechanics,
Vol. 950,
Issue. ,
Acharya, Nilankush
2022.
On the magnetohydrodynamic natural convective alumina nanofluidic transport inside a triangular enclosure fitted with fins.
Journal of the Indian Chemical Society,
Vol. 99,
Issue. 12,
p.
100784.
Unnikrishnan, Akash
Shahane, Shantanu
Narayanan, Vinod
and
Vanka, Surya Pratap
2022.
Shear-driven flow in an elliptical enclosure generated by an inner rotating circular cylinder.
Physics of Fluids,
Vol. 34,
Issue. 1,
Acharya, Nilankush
2022.
Effects of different thermal modes of obstacles on the natural convective Al2O3-water nanofluidic transport inside a triangular cavity.
Proceedings of the Institution of Mechanical Engineers, Part C: Journal of Mechanical Engineering Science,
Vol. 236,
Issue. 10,
p.
5282.
Borbora, Mustaque Hussain
Vasu, B.
and
Chamkha, Ali J.
2023.
A Review Study of Numerical Simulation of Lid-Driven Cavity Flow with Nanofluids.
Journal of Nanofluids,
Vol. 12,
Issue. 3,
p.
589.
An, Bo
Bergadà, Josep M.
Sang, Weimin
Li, Dong
and
Mellibovsky, F.
2023.
Square cavity flow driven by two mutually facing sliding walls.
Journal of Zhejiang University-SCIENCE A,
Vol. 24,
Issue. 7,
p.
612.
An, Bo
Guo, Shipeng
and
Bergadà, Josep M.
2023.
Lid Driven Triangular and Trapezoidal Cavity Flow: Vortical Structures for Steady Solutions and Hopf Bifurcations.
Applied Sciences,
Vol. 13,
Issue. 2,
p.
888.
An, Bo
Bergadà, J.M.
and
Sang, W.M.
2023.
A simplified new multigrid algorithm of lattice Boltzmann method for steady states.
Computers & Mathematics with Applications,
Vol. 135,
Issue. ,
p.
102.
An, Bo
Bergadà, J.M.
Li, D.
and
Sang, W.M.
2023.
Modified algorithms for curved and virtual boundaries in Lattice Boltzmann method applications based on tree grid.
Computers & Mathematics with Applications,
Vol. 146,
Issue. ,
p.
71.
Chen, Bai-Hui
Shi, Bao-Chang
Wang, Lei
and
Chai, Zhen-Hua
2023.
GPU based lattice Boltzmann simulation and analysis of two-dimensional trapezoidal cavity flow.
Acta Physica Sinica,
Vol. 72,
Issue. 15,
p.
154701.
Pichi, Federico
Ballarin, Francesco
Rozza, Gianluigi
and
Hesthaven, Jan S.
2023.
An artificial neural network approach to bifurcating phenomena in computational fluid dynamics.
Computers & Fluids,
Vol. 254,
Issue. ,
p.
105813.
Unnikrishnan, Akash
Narayanan, Vinod
Chamorro, Leonardo P.
and
Vanka, Surya Pratap
2024.
Taylor–Couette flow and heat transfer in an elliptical enclosure with a rotating inner cylinder.
Physics of Fluids,
Vol. 36,
Issue. 3,
Hu, X.
Bergadà, J. M.
Li, D.
Sang, W. M.
and
An, B.
2024.
A modified lattice Boltzmann approach based on radial basis function approximation for the non‐uniform rectangular mesh.
International Journal for Numerical Methods in Fluids,
Vol. 96,
Issue. 11,
p.
1695.
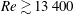 $Re\gtrsim 13\,400$, chaotic motion is nevertheless observed from as low as
$Re\gtrsim 13\,400$, chaotic motion is nevertheless observed from as low as 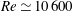 $Re\simeq 10\,600$. Chaotic dynamics is shown to arise from the destabilisation, following a variant of the classic Ruelle–Takens route, of a secondary solution branch that emerges at a relatively low
$Re\simeq 10\,600$. Chaotic dynamics is shown to arise from the destabilisation, following a variant of the classic Ruelle–Takens route, of a secondary solution branch that emerges at a relatively low 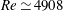 $Re\simeq 4908$ and appears to bear no connection to the base state. We analyse the bifurcation sequence that takes the flow from steady to periodic and then quasi-periodic and show that the invariant torus is finally destroyed in a period-doubling cascade of a phase-locked limit cycle. As a result, a strange attractor arises that induces chaotic dynamics.
$Re\simeq 4908$ and appears to bear no connection to the base state. We analyse the bifurcation sequence that takes the flow from steady to periodic and then quasi-periodic and show that the invariant torus is finally destroyed in a period-doubling cascade of a phase-locked limit cycle. As a result, a strange attractor arises that induces chaotic dynamics.