Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Sanmiguel Vila, Carlos
Örlü, Ramis
Vinuesa, Ricardo
Schlatter, Philipp
Ianiro, Andrea
and
Discetti, Stefano
2017.
Adverse-Pressure-Gradient Effects on Turbulent Boundary Layers: Statistics and Flow-Field Organization.
Flow, Turbulence and Combustion,
Vol. 99,
Issue. 3-4,
p.
589.
Ikeya, Yuta
Örlü, Ramis
Fukagata, Koji
and
Alfredsson, P. Henrik
2017.
Towards a theoretical model of heat transfer for hot-wire anemometry close to solid walls.
International Journal of Heat and Fluid Flow,
Vol. 68,
Issue. ,
p.
248.
Vinuesa, Ricardo
Örlü, Ramis
Sanmiguel Vila, Carlos
Ianiro, Andrea
Discetti, Stefano
and
Schlatter, Philipp
2017.
Revisiting History Effects in Adverse-Pressure-Gradient Turbulent Boundary Layers.
Flow, Turbulence and Combustion,
Vol. 99,
Issue. 3-4,
p.
565.
Rezaeiravesh, Saleh
Vinuesa, Ricardo
Liefvendahl, Mattias
and
Schlatter, Philipp
2018.
Assessment of uncertainties in hot-wire anemometry and oil-film interferometry measurements for wall-bounded turbulent flows.
European Journal of Mechanics - B/Fluids,
Vol. 72,
Issue. ,
p.
57.
Deng, Sichao
Pan, Chong
Wang, Jinjun
and
He, Guosheng
2018.
On the spatial organization of hairpin packets in a turbulent boundary layer at low-to-moderate Reynolds number.
Journal of Fluid Mechanics,
Vol. 844,
Issue. ,
p.
635.
Mallor, Fermin
Sanmiguel Vila, Carlos
Ianiro, Andrea
and
Discetti, Stefano
2018.
Wall-mounted perforated cubes in a boundary layer: Local heat transfer enhancement and control.
International Journal of Heat and Mass Transfer,
Vol. 117,
Issue. ,
p.
498.
Zhao, Jisong
2018.
Measurement of Wall Shear Stress in High Speed Air Flow Using Shear-Sensitive Liquid Crystal Coating.
Sensors,
Vol. 18,
Issue. 5,
p.
1605.
Tamano, Shinji
Uchikawa, Hiroki
Ito, Jun
and
Morinishi, Yohei
2018.
Streamwise variations of turbulence statistics up to maximum drag reduction state in turbulent boundary layer flow due to surfactant injection.
Physics of Fluids,
Vol. 30,
Issue. 7,
Buxton, O. R. H.
Ewenz Rocher, M.
and
Rodríguez-López, E.
2018.
Influence of strong perturbations on wall-bounded flows.
Physical Review Fluids,
Vol. 3,
Issue. 1,
Mallor, Fermin
Raiola, Marco
Sanmiguel Vila, Carlos
Örlü, Ramis
Discetti, Stefano
and
Ianiro, Andrea
2019.
Modal decomposition of flow fields and convective heat transfer maps: An application to wall-proximity square ribs.
Experimental Thermal and Fluid Science,
Vol. 102,
Issue. ,
p.
517.
Vaquero, J.
Renard, N.
and
Deck, S.
2019.
Effects of upstream perturbations on the solution of the laminar and fully turbulent boundary layer equations with pressure gradients.
Physics of Fluids,
Vol. 31,
Issue. 12,
Güemes, A.
Sanmiguel Vila, C.
Örlü, R.
Vinuesa, R.
Schlatter, P.
Ianiro, A.
and
Discetti, S.
2019.
Flow organization in the wake of a rib in a turbulent boundary layer with pressure gradient.
Experimental Thermal and Fluid Science,
Vol. 108,
Issue. ,
p.
115.
Tang, Zhanqi
Jiang, Nan
Zheng, Xiaobo
and
Wu, Yanhua
2019.
Local dynamic perturbation effects on amplitude modulation in turbulent boundary layer flow based on triple decomposition.
Physics of Fluids,
Vol. 31,
Issue. 2,
dos Santos, Fernanda Leticia
Sanders, Martinus P.
Dantas de Santana, Leandro
and
H. Venner, Cornelis
2020.
Influence of tripping devices in hastening transition in a flat plate submitted to zero and favorable pressure gradients.
Inasawa, Ayumu
Takagi, Shohei
and
Asai, Masahito
2020.
Improvement of the signal-to-noise ratio of the constant-temperature hot-wire anemometer using the transfer function.
Measurement Science and Technology,
Vol. 31,
Issue. 5,
p.
055302.
Dróżdż, Artur
Elsner, Witold
Niegodajew, Paweł
Vinuesa, Ricardo
Örlü, Ramis
and
Schlatter, Philipp
2020.
A description of turbulence intensity profiles for boundary layers with adverse pressure gradient.
European Journal of Mechanics - B/Fluids,
Vol. 84,
Issue. ,
p.
470.
Zimmerman, S.J.
Romero, S.K.
Philip, J.
and
Klewicki, J.C.
2020.
Downstream evolution of junction flow three-component velocity fluctuations through the lens of the diagnostic plot.
International Journal of Heat and Fluid Flow,
Vol. 85,
Issue. ,
p.
108665.
Kempaiah, Kushal U.
Scarano, Fulvio
Elsinga, Gerrit E.
van Oudheusden, Bas W.
and
Bermel, Leon
2020.
3-dimensional particle image velocimetry based evaluation of turbulent
skin-friction reduction by spanwise wall oscillation.
Physics of Fluids,
Vol. 32,
Issue. 8,
Sanmiguel Vila, C.
Vinuesa, R.
Discetti, S.
Ianiro, A.
Schlatter, P.
and
Örlü, R.
2020.
Experimental realisation of near-equilibrium adverse-pressure-gradient turbulent boundary layers.
Experimental Thermal and Fluid Science,
Vol. 112,
Issue. ,
p.
109975.
Sanmiguel Vila, C.
Vinuesa, R.
Discetti, S.
Ianiro, A.
Schlatter, P.
and
Örlü, R.
2020.
Separating adverse-pressure-gradient and Reynolds-number effects in turbulent boundary layers.
Physical Review Fluids,
Vol. 5,
Issue. 6,
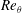 $Re_{\unicode[STIX]{x1D703}}$ of approximately 4000 (corresponding to 2.5 m from the leading edge) in a wind-tunnel experiment. Additionally, recent high-Reynolds-number data sets have been employed to validate the method. The results show that weak tripping configurations lead to deviations in the mean flow and the velocity fluctuations within the logarithmic region with respect to optimally tripped boundary layers. On the other hand, a strong trip leads to a more energized outer region, manifested in the emergence of an outer peak in the velocity-fluctuation profile and in a more prominent wake region. While established criteria based on skin-friction and shape-factor correlations yield generally equivalent results with the diagnostic-plot method in terms of convergence towards a well-behaved state, the proposed method has the advantage of being a practical surrogate that is a more efficient tool when designing the set-up for TBL experiments, since it diagnoses the state of the boundary layer without the need to perform extensive velocity profile measurements.
$Re_{\unicode[STIX]{x1D703}}$ of approximately 4000 (corresponding to 2.5 m from the leading edge) in a wind-tunnel experiment. Additionally, recent high-Reynolds-number data sets have been employed to validate the method. The results show that weak tripping configurations lead to deviations in the mean flow and the velocity fluctuations within the logarithmic region with respect to optimally tripped boundary layers. On the other hand, a strong trip leads to a more energized outer region, manifested in the emergence of an outer peak in the velocity-fluctuation profile and in a more prominent wake region. While established criteria based on skin-friction and shape-factor correlations yield generally equivalent results with the diagnostic-plot method in terms of convergence towards a well-behaved state, the proposed method has the advantage of being a practical surrogate that is a more efficient tool when designing the set-up for TBL experiments, since it diagnoses the state of the boundary layer without the need to perform extensive velocity profile measurements.