Hostname: page-component-8448b6f56d-t5pn6 Total loading time: 0 Render date: 2024-04-23T17:10:50.363Z Has data issue: false hasContentIssue false
  • English
  • Français

On the impulse response and global instability development of the infinite rotating-disc boundary layer

Published online by Cambridge University Press:  19 October 2018

Christian Thomas Open the ORCID record for Christian Thomas [Opens in a new window]  and
Christopher Davies
Christian Thomas*
Affiliation:
School of Mathematical Sciences, Monash University, Melbourne, VIC 3800, Australia
Christopher Davies
Affiliation:
School of Mathematics, Cardiff University, Cardiff CF24 4AG, UK
*
†Email address for correspondence: christian.thomas@monash.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

Linear disturbance development in the von Kármán boundary layer on an infinite rotating-disc is investigated for an extensive range of azimuthal mode numbers $n$. The study expands upon earlier investigations that were limited to those values of $n$ located near the onset of absolute instability (Lingwood, J. Fluid Mech., vol. 299, 1995, pp. 17–33), where disturbances to the genuine inhomogeneous flow were shown to be globally stable (Davies & Carpenter, J. Fluid Mech., vol. 486, 2003, pp. 287–329). Numerical simulations corresponding to azimuthal mode numbers greater than the conditions for critical absolute instability display a form of global linear instability that is characterised by a faster than exponential temporal growth, similar in appearance to that found on the rotating-disc with mass suction (Thomas & Davies, J. Fluid Mech., vol. 724, 2010, pp. 510–526) and other globally unstable flows (Huerre & Monkewitz, Annu. Rev. Fluid Mech., vol. 22, 1990, pp. 473–537). Solutions indicate that a change in the global behaviour arises for 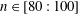$n\in [80:100]$ that is marginally greater than those disturbances studied previously. Furthermore, the Reynolds number associated with the larger azimuthal mode numbers coincides with the upper bound of experimental predictions for transition. Thus, the local–global linear stability of the infinite rotating-disc is similar to the scenario outlined by Huerre & Monkewitz (1990) that states a region of local absolute instability is necessary but not sufficient for global instability to ensue. Conditions are derived to predict the azimuthal mode number needed to bring about a change in global behaviour, based on solutions of the linearised complex Ginzburg–Landau equation coupled with numerical simulations of disturbances to the radially homogeneous flow. The long term response is governed by a detuning effect, based on radial variations of the temporal frequency and matching shifts in temporal growth that increases for larger $n$, eventually attaining values sufficient to engineer global linear instability. The analysis is extended to include mass transfer through the disc surface, with similar conclusions drawn for disturbances to large enough azimuthal mode numbers. Finally, we conclude that the high $n$ modes are unlikely to have a strong influence on disturbance development and transition in the von Kármán flow, as they will be unable to establish themselves across an extended radial range before nonlinear effects are triggered by the huge growth associated with the wavepacket maxima of the lower $n$-valued convective instabilities.

JFM classification

Instability: Absolute/convective instability Boundary Layers: Boundary layer stability
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 857 , 25 December 2018 , pp. 239 - 269
Check for updates
Copyright
© 2018 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Appelquist, E., Schlatter, P., Alfredsson, P. H. & Lingwood, R. J. 2015a Global linear instability of the rotating-disk flow investigated through simulations. J. Fluid Mech. 765, 612–631.Google Scholar
Appelquist, E., Schlatter, P., Alfredsson, P. H. & Lingwood, R. J. 2015b Investigation of the global instability of the rotating-disk boundary layer. Proc. IUTAM 14, 321–328.Google Scholar
Appelquist, E., Schlatter, P., Alfredsson, P. H. & Lingwood, R. J. 2016 On the global nonlinear instability of the rotating-disk flow over a finite domain. J. Fluid Mech. 803, 332–355.Google Scholar
Appelquist, E., Schlatter, P., Alfredsson, P. H. & Lingwood, R. J. 2018 Transition to turbulence in the rotating-disk boundary-layer flow with stationary vortices. J. Fluid Mech. 836, 43–71.Google Scholar
Briggs, R. J. 1964 Electron-Stream Interactions in Plasmas. MIT Press.Google Scholar
Chomaz, J. M., Huerre, P. & Redekopp, L. G. 1988 Bifurcations to local and global modes in spatially-developing flows. Phys. Rev. Lett. 60, 25–28.Google Scholar
Davies, C. & Carpenter, P. W. 2001 A novel velocity-vorticity formulation of the Navier–Stokes equations with applications to boundary layer disturbance evolution. J. Comput. Phys. 172, 119–165.Google Scholar
Davies, C. & Carpenter, P. W. 2003 Global behaviour corresponding to the absolute instability of the rotating-disc boundary layer. J. Fluid Mech. 486, 287–329.Google Scholar
Davies, C. & Thomas, C. 2017 Global stability behaviour for the BEK family of rotating boundary layers. Theor. Comput. Fluid Dyn. 31, 519–536.Google Scholar
Davies, C., Thomas, C. & Carpenter, P. W. 2007 Global stability of the rotating disc boundary layer. J. Engng Maths 57 (3), 219–236.Google Scholar
Faller, A. & Kaylor, R. 1966 A numerical study of the instability of the laminar Ekman boundary-layer. J. Atmos. Sci. 23, 466–480.Google Scholar
Garrett, S. J. & Peake, N. 2002 The stability and transition of the boundary layer on a rotating sphere. J. Fluid Mech. 456, 405–428.Google Scholar
Garrett, S. J. & Peake, N. 2007 The absolute instability of the boundary layer on a rotating cone. Eur. J. Mech. (B/Fluids) 26, 344–353.Google Scholar
Gregory, N., Stuart, J. T. & Walker, W. S. 1955 On the stability of three-dimensional boundary-layers with application to the flow due to a rotating disk. Phil. Trans. R. Soc. Lond. A 248, 155–199.Google Scholar
Gregory, N. & Walker, W. S. 1960 Experiments on the effect of suction on the flow due to a rotating disk. J. Fluid Mech. 9, 225–234.Google Scholar
Hannemann, K. & Oertel, H. 1990 Numerical simulation of the absolutely and convectively unstable wake. J. Fluid Mech. 199, 55–88.Google Scholar
Harris, D., Bassom, A. P. & Soward, A. M. 2000 An inhomogeneous Landau equation with application to spherical Couette flow in the narrow gap limit. Physica D 137, 260–276.Google Scholar
Healey, J. J. 2010 Model for unstable global modes in the rotating-disk boundary layer. J. Fluid Mech. 663, 148–159.Google Scholar
Huerre, P. 2000 Open shear flow instabilities. In Perspectives in Fluid Dynamics: A Collective Introduction to Current Research (ed. Batchelor, G. K., Moffatt, H. K. & Worster, M. G.). Cambridge University Press.Google Scholar
Huerre, P. & Monkewitz, P. A. 1990 Local and global instabilities in spatially developing flows. Annu. Rev. Fluid Mech. 22, 473–537.Google Scholar
Hunt, R. E. & Crighton, D. G. 1991 Instability of flows in spatially developing media. Proc. R. Soc. Lond. A 435, 109–128.Google Scholar
Imayama, S., Alfredsson, P. H. & Lingwood, R. J. 2012 A new way to describe the transition characteristics of a rotating-disk boundary-layer flow. Phys. Fluids 24, 031701.Google Scholar
Imayama, S., Alfredsson, P. H. & Lingwood, R. J. 2013 An experimental study of edge effects on rotating-disk transition. J. Fluid Mech. 716, 638–657.Google Scholar
Imayama, S., Alfredsson, P. H. & Lingwood, R. J. 2014 On the laminar-turbulent transition of the rotating-disk flow: the role of absolute instability. J. Fluid Mech. 745, 132–163.Google Scholar
Kobayashi, R., Kohama, Y. & Takamadate, C. 1980 Spiral vortices in boundary layer transition regime on a rotating disk. Acta Mech. 35, 71–82.Google Scholar
von Kármán, T. 1921 Über laminare und turbulente Reibung. Z. Angew. Math. Mech. 1, 233–252.Google Scholar
Leu, T.-Z. & Ho, C.-M. 2000 Control of global instability in a non-parallel near wake. J. Fluid Mech. 404, 345–378.Google Scholar
Lingwood, R. & Alfredsson, P. 2015 Instabilities of the von Kármán boundary layer. Appl. Mech. Rev. 67 (3), 030803.Google Scholar
Lingwood, R. J. 1995 Absolute instability of the boundary-layer on a rotating-disk. J. Fluid Mech. 299, 17–33.Google Scholar
Lingwood, R. J. 1996 An experimental study of absolute instability of the rotating-disk boundary-layer flow. J. Fluid Mech. 314, 373–405.Google Scholar
Lingwood, R. J. 1997a On the effects of suction and injection on the absolute instability of the rotating-disk boundary layers. Phys. Fluids 9, 1317–1328.Google Scholar
Lingwood, R. J. 1997b Absolute instability of the Ekman layer and related rotating flows. J. Fluid Mech. 331, 405–428.Google Scholar
Mack, L. M.1985 The wave pattern produced by point source on a rotating disk. AIAA Paper 85-0490.Google Scholar
Malik, M. R. 1986 The neutral curve for stationary disturbances in rotating-disk flow. J. Fluid Mech. 164, 275–287.Google Scholar
Malik, M. R., Wilkinson, S. & Orszag, S. A. 1981 Instability and transition in rotating disk flow. AIAA J. 19, 1131–1138.Google Scholar
Maxworthy, T. 1999 The flickering candle: transition to a global oscillation in a thermal plume. J. Fluid Mech. 390, 297–323 (and Corrigendum 399, 377).Google Scholar
Oertel, H. 1990 Wakes behind blunt bodies. Annu. Rev. Fluid Mech. 22, 539–564.Google Scholar
Othman, H. & Corke, T. C. 2006 Experimental investigation of absolute instability of a rotating-disk boundary-layer. J. Fluid Mech. 565, 63–94.Google Scholar
Pier, B. 2003 Finite-amplitude crossflow vortices, secondary instability and transition in the rotating-disk boundary layer. J. Fluid Mech. 487, 315–343.Google Scholar
Pier, B. 2007 Primary crossflow vortices, secondary absolute instabilities and their control in the rotating-disk boundary layer. J. Engng Maths 57, 237–251.Google Scholar
Pier, B. 2013 Transition near the edge of a rotating disk. J. Fluid Mech. 737, R1.Google Scholar
Schmid, P. J. & Henningson, D. 2001 Open Shear Flow Instabilities. Springer.Google Scholar
Smith, A. M. O. & Gamberoni, A. H.1956 Transition, pressure gradient and stability theory. Douglas Aircraft Co. Rep. No. ES26388.Google Scholar
Soward, A. M. 1977 On the finite amplitude thermal instability of a rapidly rotating sphere. Geophys. Astrophys. Fluid Dyn. 9, 19–74.Google Scholar
Soward, A. M. 1992 Thin disc kinematics 𝛼𝜔-dynamo models. Part II. Short length scale modes. Geophys. Astrophys. Fluid Dyn. 64, 201–225.Google Scholar
Thomas, C.2007 Numerical simulations of disturbance development in rotating boundary-layers. PhD thesis, Cardiff University.Google Scholar
Thomas, C. & Davies, C. 2010 The effects of mass transfer on the global stability of the rotating-disk boundary laer. J. Fluid Mech. 724, 510–526.Google Scholar
Thomas, C. & Davies, C. 2013 Global stability of the rotating-disc boundary layer with an axial magnetic field. J. Fluid Mech. 724, 510–526.Google Scholar
Van Ingen, J. L.1956 A suggested semi-empirical method for the calculation of the boundary layer transition region. Dept. Aeronaut. Eng., Delft Univ. Tech., Rep. No. VTH-74.Google Scholar
Viaud, B., Serre, E. & Chomaz, J.-M. 2011 Transition to turbulence through steep global-modes cascade in an open rotating cavity. J. Fluid Mech. 688, 493–506.Google Scholar
Wilkinson, S. & Malik, M. R. 1985 Stability experiments in the flow over a rotating disk. AIAA J. 23, 588–595.Google Scholar
Zielinska, J. A. & Westfried, J. E. 1995 On the spatial structure of global modes in wake flow. Phys. Fluids 7, 1418–1424.Google Scholar