Hostname: page-component-76fb5796d-dfsvx Total loading time: 0 Render date: 2024-04-26T01:18:09.442Z Has data issue: false hasContentIssue false
  • English
  • Français

Optimal perturbations in viscous round jets subject to Kelvin–Helmholtz instability

Published online by Cambridge University Press:  07 August 2020

Gabriele Nastro Open the ORCID record for Gabriele Nastro [Opens in a new window] ,
Jérôme Fontane Open the ORCID record for Jérôme Fontane [Opens in a new window]  and
Laurent Joly Open the ORCID record for Laurent Joly [Opens in a new window]
Gabriele Nastro*
Affiliation:
ISAE-SUPAERO, Université de Toulouse, 31055 Toulouse, France
Jérôme Fontane
Affiliation:
ISAE-SUPAERO, Université de Toulouse, 31055 Toulouse, France
Laurent Joly
Affiliation:
ISAE-SUPAERO, Université de Toulouse, 31055 Toulouse, France
*
†Email address for correspondence: gabriele.nastro@isae-supaero.fr
Get access Rights & Permissions [Opens in a new window]

Abstract

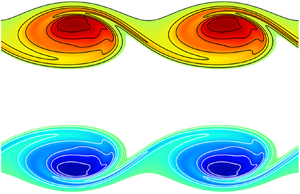
We investigate the development of three-dimensional instabilities on a time-dependent round jet undergoing the axisymmetric Kelvin–Helmholtz (KH) instability. A non-modal linear stability analysis of the resulting unsteady roll-up into a vortex ring is performed based on a direct-adjoint approach. Varying the azimuthal wavenumber $m$, the Reynolds number ${Re}$ and the aspect ratio $\alpha$ of the jet base flow, we explore the potential for secondary energy growth beyond the initial phase when the base flow is still quasi-parallel and universal shear-induced transient growth occurs. For ${Re}=1000$ and $\alpha = 10$, the helical $m=1$ and double-helix $m=2$ perturbations stand as global optimals with larger growth rates in the post roll-up phase. The secondary energy growth stems from the development of elliptical (E-type) and hyperbolic (H-type) instabilities. For $m>2$, the maximum of the kinetic energy of the optimal perturbation moves from the large scale vortex core towards the thin vorticity braid. With a Reynolds number one order of magnitude larger, the kinetic energy of the optimal perturbations exhibits sustained growth well after the saturation time of the base flow KH wave and the underlying length scale selection favours higher azimuthal wavenumbers associated with H-type instability in the less diffused vorticity braid. Doubling the jet aspect ratio yields initially thinner shear layers only slightly affected by axisymmetry. The resulting unsteady base flow loses scale selectivity and is prone to a common path of initial transient growth followed by the optimal secondary growth of a wide range of wavenumbers. Increasing both the aspect ratio and the Reynolds number thus yields an even larger secondary growth and a lower wavenumber selectivity. At a lower aspect ratio of $\alpha =5$, the base flow is smooth and a genuine round jet affected by the axisymmetry condition. The axisymmetric modal perturbation of the base flow parallel jet only weakly affects the first common phase of transient growth and the optimal helical perturbation $m=1$ dominates with energy gains considerably larger than those of larger azimuthal wavenumbers whatever the horizon time.

JFM classification

Wakes/Jets: Jets
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 900 , 10 October 2020 , A13
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Abid, M., Huerre, P. & Brachet, M. 1993 Linear hydrodynamic instability of circular jets with thin shear layers. Eur. J. Mech. B/Fluids 12 (5), 683–693.Google Scholar
Arratia, C., Caulfield, C. P. & Chomaz, J.-M. 2013 Transient perturbation growth in time-dependent mixing layers. J. Fluid Mech. 717, 90–133.CrossRefGoogle Scholar
Batchelor, G. K. & Gill, A. E. 1962 Analysis of the stability of axisymmetric jets. J. Fluid Mech. 14 (4), 529–551.CrossRefGoogle Scholar
Becker, H. A. & Massaro, T. A. 1968 Vortex evolution in a round jet. J. Fluid Mech. 31 (3), 435–448.CrossRefGoogle Scholar
Boronin, S. A., Healey, J. J. & Sazhin, S. S. 2013 Non-modal stability of round viscous jets. J. Fluid Mech. 716, 96–119.CrossRefGoogle Scholar
Brancher, P., Chomaz, J.-M. & Huerre, P. 1994 Direct numerical simulations of round jets: vortex induction and side jets. Phys. Fluids 6 (5), 1768–1774.CrossRefGoogle Scholar
Caulfield, C. P. & Kerswell, R. R. 2000 The nonlinear development of three-dimensional disturbances at hyperbolic stagnation points: a model of the braid region in mixing layers. Phys. Fluids 12 (5), 1032–1043.CrossRefGoogle Scholar
Corbett, P. & Bottaro, A. 2000 Optimal perturbations for boundary layers subject to stream-wise pressure gradient. Phys. Fluids 12 (1), 120–130.CrossRefGoogle Scholar
Corbett, P. & Bottaro, A. 2001 Optimal linear growth in swept boundary layers. J. Fluid Mech. 435, 1–23.CrossRefGoogle Scholar
Crighton, D. G. & Gaster, M. 1976 Stability of slowly diverging jet flow. J. Fluid Mech. 77 (2), 397–413.CrossRefGoogle Scholar
Drazin, P. G. & Reid, W. H. 1981 Hydrodynamic stability. Cambridge University Press.Google Scholar
Ellingsen, T. & Palm, E. 1975 Stability of linear flow. Phys. Fluids 18 (4), 487–488.CrossRefGoogle Scholar
Farrell, B. F. 1988 Optimal excitation of perturbations in viscous shear flow. Phys. Fluids 31 (8), 2093–2102.CrossRefGoogle Scholar
Farrell, B. F. & Ioannou, P. J. 1993 Perturbation growth in shear flow exhibits universality. Phys. Fluids A 5 (9), 2298–2300.CrossRefGoogle Scholar
Fontane, J. & Joly, L. 2008 The stability of the variable-density Kelvin–Helmholtz billow. J. Fluid Mech. 612, 237–260.CrossRefGoogle Scholar
Garnaud, X., Lesshafft, L., Schmid, P. J. & Huerre, P. 2013 a Modal and transient dynamics of jet flows. Phys. Fluids 25 (4), 044103.CrossRefGoogle Scholar
Garnaud, X., Lesshafft, L., Schmid, P. J. & Huerre, P. 2013 b The preferred mode of incompressible jets: linear frequency response analysis. J. Fluid Mech. 716, 189–202.CrossRefGoogle Scholar
Gunzburger, M. 2002 Perspectives in Flow Control and Optimization. Society for Industrial and Applied Mathematics.CrossRefGoogle Scholar
Jimenez-Gonzalez, J. I. & Brancher, P. 2017 Transient energy growth of optimal streaks in parallel round jets. Phys. Fluids 29 (11), 114101.CrossRefGoogle Scholar
Jimenez-Gonzalez, J. I., Brancher, P. & Martinez-Bazan, C. 2015 Modal and non-modal evolution of perturbations for parallel round jets. Phys. Fluids 27 (4), 044105.CrossRefGoogle Scholar
Joly, L., Fontane, J. & Chassaing, P. 2005 The Rayleigh–Taylor instability of two-dimensional high-density vortices. J. Fluid Mech. 537, 415–431.CrossRefGoogle Scholar
Joly, L. & Reinaud, J. N. 2007 The merger of two-dimensional radially stratified high-Froude-number vortices. J. Fluid Mech. 582, 133–151.CrossRefGoogle Scholar
Khorrami, M. R., Malik, M. R. & Ash, R. L. 1989 Application of spectral collocation techniques to the stability of swirling flows. J. Comput. Phys. 81 (1), 206–229.CrossRefGoogle Scholar
Klaassen, G. P. & Peltier, W. R. 1991 The influence of stratification on secondary instability in free shear layers. J. Fluid Mech. 227, 71–106.CrossRefGoogle Scholar
Landahl, M. T. 1975 Wave breakdown and turbulence. SIAM J. Appl. Maths 28 (4), 735–756.CrossRefGoogle Scholar
Landahl, M. T. 1980 A note on an algebraic instability of inviscid parallel shear flows. J. Fluid Mech. 98 (2), 243–251.CrossRefGoogle Scholar
Lasheras, J. C., Cho, J. S. & Maxworthy, T. 1986 On the origin and evolution of streamwise vortical structures in a plane, free shear layer. J. Fluid Mech. 172, 231–258.CrossRefGoogle Scholar
Lasheras, J. C. & Choi, H. 1988 Three-dimensional instability of a plane free shear layer: an experimental study of the formation and evolution of streamwise vortices. J. Fluid Mech. 189, 53–86.CrossRefGoogle Scholar
Lessen, M. P. & Singh, P. J. 1973 The stability of axisymmetric free shear layers. J. Fluid Mech. 60 (3), 433–457.CrossRefGoogle Scholar
Liepmann, D. 1991 Streamwise vorticity and entrainment in the near field of a round jet. Phys. Fluids A 3 (5), 1179–1185.CrossRefGoogle Scholar
Liepmann, D. & Gharib, M. 1992 The role of streamwise vorticity in the near-field entrainment of round jets. J. Fluid Mech. 245, 643–668.CrossRefGoogle Scholar
Lopez-Zazueta, A. 2015 Stabilité secondaire non-modale d'une couche de mélange inhomogène. PhD thesis, Université de Toulouse, Institut Supérieur de l'Aéronautique et de l'Espace.Google Scholar
Lopez-Zazueta, A., Fontane, J. & Joly, L. 2016 Optimal perturbations in time-dependent variable-density Kelvin–Helmholtz billows. J. Fluid Mech. 803, 466–501.CrossRefGoogle Scholar
Luchini, P. & Bottaro, A. 1998 Görtler vortices: a backward-in-time approach to the receptivity problem. J. Fluid Mech. 363, 1–23.CrossRefGoogle Scholar
Martin, J. E. & Meiburg, E. 1991 Numerical investigation of three-dimensionally evolving jets subject to axisymmetric and azimuthal perturbations. J. Fluid Mech. 230, 271–318.CrossRefGoogle Scholar
Michalke, A. 1964 On the inviscid instability of the hyperbolic-tangent velocity profile. J. Fluid Mech. 60 (4), 543–556.CrossRefGoogle Scholar
Michalke, A. 1971 Instabilität eines kompressiblen runden freistrahls unter berücksichtigung des einflusses des strahlgrenzschichtdicke. Z. Flugwiss 8–9, 319–328.Google Scholar
Michalke, A. 1984 Survey on jet instability theory. Prog. Aerosp. Sci. 21, 159–199.CrossRefGoogle Scholar
Monkewitz, P. A., Bechert, D. W., Barsikow, B. & Lehmann, B. 1990 Self-excited oscillations and mixing in a heated round jet. J. Fluid Mech. 213, 611–639.CrossRefGoogle Scholar
Monkewitz, P. A., Lehmann, B., Barsikow, B. & Bechert, D. W. 1989 The spreading of self-excited hot jets by side jets. Phys. Fluids 1 (3), 446–448.CrossRefGoogle Scholar
Monkewitz, P. A. & Pfizenmaier, E. 1991 Mixing by side jets in strongly forced and self-excited round jets. Phys. Fluids 3 (5), 1356–1361.CrossRefGoogle Scholar
Morris, P. J. 1976 The spatial viscous instability of axisymmetric jets. J. Fluid Mech. 77 (3), 511–529.CrossRefGoogle Scholar
Moser, R. D. & Rogers, M. M. 1993 The three-dimensional evolution of a plane mixing layer: pairing and transition to turbulence. J. Fluid Mech. 247, 275–320.CrossRefGoogle Scholar
Orr, W. M. F. 1907 The stability or instability of the steady motions of a perfect liquid and of a viscous liquid. Part I: A perfect liquid. Part II: A viscous liquid. Proc. R Irish Acad. A, 27, 9–138.Google Scholar
Ortiz, S. & Chomaz, J.-M. 2011 Transient growth of secondary instabilities in parallel wakes: anti lift-up mechanism and hyperbolic instability. Phys. Fluids 23 (11), 114106.CrossRefGoogle Scholar
Pierrehumbert, R. T. & Widnall, S. E. 1982 The two-and three-dimensional instabilities of a spatially periodic shear layer. J. Fluid Mech. 114, 59–82.CrossRefGoogle Scholar
Plaschko, P. 1979 Helical instabilities of slowly divergent jets. J. Fluid Mech. 92, 209–215.CrossRefGoogle Scholar
Reynolds, W. C. & Bouchard, E. E. 1981 The effect of forcing on the mixing-layer region of a round jet. In Unsteady Turbulent Shear Flows (ed. Michel, R., Cousteix, J. & Houdeville, R.), pp. 402–411. IUTAM, Springer.CrossRefGoogle Scholar
Rogers, M. M. & Moser, R. D. 1993 Spanwise scale selection in plane mixing layers. J. Fluid Mech. 247, 321–337.CrossRefGoogle Scholar
Schmid, P. J. 2007 Nonmodal stability theory. Annu. Rev. Fluid Mech. 39 (1), 129–162.CrossRefGoogle Scholar
Yule, A. J. 1978 Large-scale structure in the mixing layer of a round jet. J. Fluid Mech. 89 (3), 413–432.CrossRefGoogle Scholar