Hostname: page-component-784d4fb959-4tbfc Total loading time: 0 Render date: 2025-07-15T07:03:58.082Z Has data issue: false hasContentIssue false
  • English
  • Français

Quasi-local method of wave decomposition in a slowly varying medium

Published online by Cambridge University Press:  29 November 2019

Yohei Onuki Open the ORCID record for Yohei Onuki [Opens in a new window]
Yohei Onuki*
Affiliation:
Research Institute for Applied Mechanics, Kyushu University, 6-1 Kasuga-koen, Kasuga, Fukuoka, Japan
*
†Email address for correspondence: onuki@riam.kyushu-u.ac.jp
Get access Rights & Permissions [Opens in a new window]

Abstract

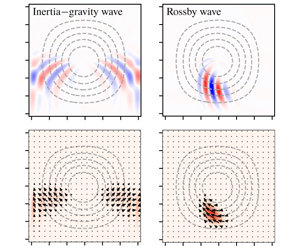
The general asymptotic theory for wave propagation in a slowly varying medium, classically known as the Wentzel–Kramers–Brillouin–Jeffreys (WKBJ) approximation, is revisited here with the aim of constructing a new data diagnostic technique useful in atmospheric and oceanic sciences. Using the Wigner transform, a kind of mapping that associates a linear operator with a function, we analytically decompose a flow field into mutually independent wave signals. This method takes account of the variations in the polarisation relations, an eigenvector that represents the kinematic characteristics of each wave component, so as to project the variables onto their eigenspace quasi-locally. The temporal evolution of a specific mode signal obeys a single wave equation characterised by the dispersion relation that also incorporates the effect from the local gradient in the medium. Combining this method with transport theory and applying them to numerical simulation data, we can detect the transfer of energy or other conserved quantities associated with the propagation of each wave signal in a wide variety of situations.

JFM classification

Geophysical and Geological Flows: Waves in rotating fluids Mathematical Foundations: Computational methods

Information

Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 883 , 25 January 2020 , A56
Copyright
© 2019 Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Aiki, H., Greatbatch, R. J. & Claus, M. 2017 Towards a seamlessly diagnosable expression for the energy flux associated with both equatorial and mid-latitude waves. Prog. Earth Planet. Sci. 4 (1), 11.CrossRefGoogle Scholar
Bender, C. M. & Orszag, S. A. 1999 Advanced Mathematical Methods for Scientists and Engineers I: Asymptotic Methods and Perturbation Theory. Springer.CrossRefGoogle Scholar
Bretherton, F. P. 1968 Propagation in slowly varying waveguides. Proc. R. Soc. Lond. A 302 (1471), 555–576.Google Scholar
Bühler, O. 2014 Waves and Mean Flows, 2nd edn. Cambridge University Press.CrossRefGoogle Scholar
Bühler, O., Callies, J. & Ferrari, R. 2014 Wave-vortex decomposition of one-dimensional ship-track data. J. Fluid Mech. 756, 1007–1026.CrossRefGoogle Scholar
Bühler, O., Kuang, M. & Tabak, E. G. 2017 Anisotropic Helmholtz and wave-vortex decomposition of one-dimensional spectra. J. Fluid Mech. 815, 361–387.CrossRefGoogle Scholar
Chassande-Mottin, E. & Pai, A. 2005 Discrete time and frequency Wigner–Ville distribution: Moyal’s formula and aliasing. IEEE Signal Process. Lett. 12, 508–511.CrossRefGoogle Scholar
Chouksey, M., Eden, C. & Brüggemann, N. 2018 Internal gravity wave emission in different dynamical regimes. J. Phys. Oceanogr. 48 (8), 1709–1730.CrossRefGoogle Scholar
Cohen, L. 2012 The Weyl Operator and its Generalization. Springer Basel.Google Scholar
Danioux, E. & Vanneste, J. 2016 Near-inertial-wave scattering by random flows. Phys. Rev. Fluids 1 (3), 033701.CrossRefGoogle Scholar
Eden, C. & Olbers, D. 2017 A closure for eddy-mean flow effects based on the Rossby wave energy equation. Ocean Model. 114, 59–71.CrossRefGoogle Scholar
Gérard, P., Markowich, P. A., Mauser, N. J. & Poupaud, F. 1997 Homogenization limits and Wigner transforms. Commun. Pure Appl. Maths 50 (4), 323–379.3.0.CO;2-C>CrossRefGoogle Scholar
Guo, M. & Wang, X. 1999 Transport equations for a general class of evolution equations with random perturbations. J. Math. Phys. 40 (10), 4828–4858.CrossRefGoogle Scholar
Haidvogel, D. B. & Beckmann, A. 1999 Numerical Ocean Circulation Modeling. World Scientific.CrossRefGoogle Scholar
Kafiabad, H. A., Savva, M. A. C. & Vanneste, J. 2019 Diffusion of inertia-gravity waves by geostrophic turbulence. J. Fluid Mech. 869, R7.CrossRefGoogle Scholar
Kinoshita, T. & Sato, K. 2013 A formulation of unified three-dimensional wave activity flux of inertia-gravity waves and Rossby waves. J. Atmos. Sci. 70 (6), 1603–1615.CrossRefGoogle Scholar
Kinoshita, T., Tomikawa, Y. & Sato, K. 2010 On the three-dimensional residual mean circulation and wave activity flux of the primitive equations. J. Met. Soc. Japan 88 (3), 373–394.Google Scholar
Kubo, R. 1964 Wigner representation of quantum operators and its applications to electrons in a magnetic field. J. Phys. Soc. Japan 19 (11), 2127–2139.CrossRefGoogle Scholar
Kunze, E. 1985 Near-inertial wave propagation in geostrophic shear. J. Phys. Oceanogr. 15 (5), 544–565.2.0.CO;2>CrossRefGoogle Scholar
Lien, R.-C. & Müller, P. 1992 Normal-mode decomposition of small-scale oceanic motions. J. Phys. Oceanogr. 22 (12), 1583–1595.2.0.CO;2>CrossRefGoogle Scholar
Lighthill, J. 1978 Waves in Fluids. Cambridge University Press.Google Scholar
Littlejohn, R. G. & Flynn, W. G. 1991 Geometric phases in the asymptotic theory of coupled wave equations. Phys. Rev. A 44 (8), 5239–5256.CrossRefGoogle ScholarPubMed
Machenhauer, B. 1977 On the dynamics of gravity oscillations in a shallow water model, with applications to normal mode initialization. Beitr. Phys. Atmos. 50, 253–271.Google Scholar
MacKinnon, J. A., Zhao, Z., Whalen, C. B., Waterhouse, A. F., Trossman, D. S., Sun, O. M., Laurent, L. C. S., Simmons, H. L., Polzin, K., Pinkel, R. et al. 2017 Climate process team on internal wave-driven ocean mixing. Bull. Am. Meteorol. Soc. 98 (11), 2429–2454.CrossRefGoogle Scholar
Matsuno, T. 1966 Quasi-geostrophic motions in the equatorial area. J. Met. Soc. Japan. Ser. II 44 (1), 25–43.CrossRefGoogle Scholar
McDonald, S. W. 1988 Phase-space representations of wave equations with applications to the eikonal approximation for short-wavelength waves. Phys. Rep. 158 (6), 337–416.Google Scholar
McKee, W. D. 1973 Internal-inertia waves in a fluid of variable depth. Math. Proc. Camb. Phil. Soc. 73 (1), 205–213.CrossRefGoogle Scholar
Morrison, P. J. 1998 Hamiltonian description of the ideal fluid. Rev. Mod. Phys. 70 (2), 467–521.CrossRefGoogle Scholar
Olbers, D. & Eden, C. 2013 A global model for the diapycnal diffusivity induced by internal gravity waves. J. Phys. Oceanogr. 43 (8), 1759–1779.CrossRefGoogle Scholar
Pedlosky, J. 1987 Geophysical Fluid Dynamics. Springer.CrossRefGoogle Scholar
Petruccione, F. & Breuer, H.-P. 2002 The Theory of Open Quantum Systems. Oxford University Press.Google Scholar
Plumb, R. A. 1985 On the three-dimensional propagation of stationary waves. J. Atmos. Sci. 42 (3), 217–229.2.0.CO;2>CrossRefGoogle Scholar
Powell, J. M. & Vanneste, J. 2005 Transport equations for waves in randomly perturbed Hamiltonian systems, with application to Rossby waves. Wave Motion 42 (4), 289–308.Google Scholar
Ryzhik, L., Papanicolaou, G. & Keller, J. B. 1996 Transport equations for elastic and other waves in random media. Wave Motion 24 (4), 327–370.Google Scholar
Sakurai, J. J. & Napolitano, J. 2011 Modern Quantum Mechanics. Addison-Wesley.Google Scholar
Sato, K., Kinoshita, T. & Okamoto, K. 2013 A new method to estimate three-dimensional residual-mean circulation in the middle atmosphere and its application to gravity wave-resolving general circulation model data. J. Atmos. Sci. 70 (12), 3756–3779.CrossRefGoogle Scholar
Savva, M. A. C. & Vanneste, J. 2018 Scattering of internal tides by barotropic quasigeostrophic flows. J. Fluid Mech. 856, 504–530.CrossRefGoogle Scholar
Shepherd, T. G. 1990 Symmetries, conservation laws, and Hamiltonian structure in geophysical fluid dynamics. Adv. Geophys. 32, 287–338.CrossRefGoogle Scholar
Takaya, K. & Nakamura, H. 1997 A formulation of a wave-activity flux for stationary Rossby waves on a zonally varying basic flow. Geophys. Res. Lett. 24, 2985–2988.CrossRefGoogle Scholar
Takaya, K. & Nakamura, H. 2001 A formulation of a phase-independent wave-activity flux for stationary and migratory quasigeostrophic eddies on a zonally varying basic flow. J. Atmos. Sci. 58 (6), 608–627.2.0.CO;2>CrossRefGoogle Scholar
Vanneste, J. 2013 Balance and spontaneous wave generation in geophysical flows. Annu. Rev. Fluid Mech. 45, 147–172.CrossRefGoogle Scholar
Vanneste, J. & Shepherd, T. G. 1999 On wave action and phase in the non–canonical Hamiltonian formulation. Proc. R. Soc. Lond. A 455, 3–21.CrossRefGoogle Scholar
WAMDI Group 1988 The WAM model – a third generation ocean wave prediction model. J. Phys. Oceanogr. 18 (12), 1775–1810.2.0.CO;2>CrossRefGoogle Scholar
Warn, T., Bokhove, O., Shepherd, T. G. & Vallis, G. K. 1995 Rossby number expansions, slaving principles, and balance dynamics. Q. J. R. Meteorol. Soc. 121 (523), 723–739.CrossRefGoogle Scholar
Whitham, G. B. 1970 Two-timing, variational principles and waves. J. Fluid Mech. 44 (2), 373–395.CrossRefGoogle Scholar
Wordsworth, R. D. 2009 A phase-space study of jet formation in planetary-scale fluids. Phys. Fluids 21 (5), 056602.CrossRefGoogle Scholar
Yasuda, Y., Sato, K. & Sugimoto, N. 2015 A theoretical study on the spontaneous radiation of inertia–gravity waves using the renormalization group method. Part I. Derivation of the renormalization group equations. J. Atmos. Sci. 72 (3), 957–983.CrossRefGoogle Scholar
Yiğit, E. & Medvedev, A. S. 2015 Internal wave coupling processes in Earth’s atmosphere. Adv. Space Res. 55 (4), 983–1003.CrossRefGoogle Scholar
Žagar, N., Kasahara, A., Terasaki, K., Tribbia, J. & Tanaka, H. 2015 Normal-mode function representation of global 3-D data sets: open-access software for the atmospheric research community. Geosci. Model Develop. 8, 1169–1195.CrossRefGoogle Scholar