Article contents
Resolved and subgrid dynamics of Rayleigh–Bénard convection
Published online by Cambridge University Press: 28 March 2019
Abstract
In this work we present and demonstrate the reliability of a theoretical framework for the study of thermally driven turbulence. It consists of scale-by-scale budget equations for the second-order velocity and temperature structure functions and their limiting cases, represented by the turbulent kinetic energy and temperature variance budgets. This framework represents an extension of the classical Kolmogorov and Yaglom equations to inhomogeneous and anisotropic flows, and allows for a novel assessment of the turbulent processes occurring at different scales and locations in the fluid domain. Two relevant characteristic scales, 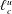 $\ell _{c}^{u}$ for the velocity field and
$\ell _{c}^{u}$ for the velocity field and 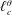 $\ell _{c}^{\unicode[STIX]{x1D703}}$ for the temperature field, are identified. These variables separate the space of scales into a quasi-homogeneous range, characterized by turbulent kinetic energy and temperature variance cascades towards dissipation, and an inhomogeneity-dominated range, where the production and the transport in physical space are important. This theoretical framework is then extended to the context of large-eddy simulation to quantify the effect of a low-pass filtering operation on both resolved and subgrid dynamics of turbulent Rayleigh–Bénard convection. It consists of single-point and scale-by-scale budget equations for the filtered velocity and temperature fields. To evaluate the effect of the filter length
$\ell _{c}^{\unicode[STIX]{x1D703}}$ for the temperature field, are identified. These variables separate the space of scales into a quasi-homogeneous range, characterized by turbulent kinetic energy and temperature variance cascades towards dissipation, and an inhomogeneity-dominated range, where the production and the transport in physical space are important. This theoretical framework is then extended to the context of large-eddy simulation to quantify the effect of a low-pass filtering operation on both resolved and subgrid dynamics of turbulent Rayleigh–Bénard convection. It consists of single-point and scale-by-scale budget equations for the filtered velocity and temperature fields. To evaluate the effect of the filter length 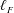 $\ell _{F}$ on the resolved and subgrid dynamics, the velocity and temperature fields obtained from a direct numerical simulation are split into filtered and residual components using a spectral cutoff filter. It is found that when
$\ell _{F}$ on the resolved and subgrid dynamics, the velocity and temperature fields obtained from a direct numerical simulation are split into filtered and residual components using a spectral cutoff filter. It is found that when 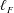 $\ell _{F}$ is smaller than the minimum values of the cross-over scales given by
$\ell _{F}$ is smaller than the minimum values of the cross-over scales given by  $\ell _{c,min}^{\unicode[STIX]{x1D703}\ast }=\ell _{c,min}^{\unicode[STIX]{x1D703}}Nu/H=0.8$, the resolved processes correspond to the exact ones, except for a depletion of viscous and thermal dissipations, and the only role of the subgrid scales is to drain turbulent kinetic energy and temperature variance to dissipate them. On the other hand, the resolved dynamics is much poorer in the near-wall region and the effects of the subgrid scales are more complex for filter lengths of the order of
$\ell _{c,min}^{\unicode[STIX]{x1D703}\ast }=\ell _{c,min}^{\unicode[STIX]{x1D703}}Nu/H=0.8$, the resolved processes correspond to the exact ones, except for a depletion of viscous and thermal dissipations, and the only role of the subgrid scales is to drain turbulent kinetic energy and temperature variance to dissipate them. On the other hand, the resolved dynamics is much poorer in the near-wall region and the effects of the subgrid scales are more complex for filter lengths of the order of 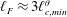 $\ell _{F}\approx 3\ell _{c,min}^{\unicode[STIX]{x1D703}}$ or larger. This study suggests that classic eddy-viscosity/diffusivity models employed in large-eddy simulation may suffer from some limitations for large filter lengths, and that alternative closures should be considered to account for the inhomogeneous processes at subgrid level. Moreover, the theoretical framework based on the filtered Kolmogorov and Yaglom equations may represent a valuable tool for future assessments of the subgrid-scale models.
$\ell _{F}\approx 3\ell _{c,min}^{\unicode[STIX]{x1D703}}$ or larger. This study suggests that classic eddy-viscosity/diffusivity models employed in large-eddy simulation may suffer from some limitations for large filter lengths, and that alternative closures should be considered to account for the inhomogeneous processes at subgrid level. Moreover, the theoretical framework based on the filtered Kolmogorov and Yaglom equations may represent a valuable tool for future assessments of the subgrid-scale models.
Information
- Type
- JFM Papers
- Information
- Copyright
- © 2019 Cambridge University Press
References
- 8
- Cited by

