Article contents
Scaling laws for jets of single cavitation bubbles
Published online by Cambridge University Press: 03 August 2016
Abstract
Fast liquid jets, called micro-jets, are produced within cavitation bubbles experiencing an aspherical collapse. Here we review micro-jets of different origins, scales and appearances, and propose a unified framework to describe their dynamics by using an anisotropy parameter  $\unicode[STIX]{x1D701}\geqslant 0$ , representing a dimensionless measure of the liquid momentum at the collapse point (Kelvin impulse). This parameter is rigorously defined for various jet drivers, including gravity and nearby boundaries. Combining theoretical considerations with hundreds of high-speed visualisations of bubbles collapsing near a rigid surface, near a free surface or in variable gravity, we classify the jets into three distinct regimes: weak, intermediate and strong. Weak jets (
$\unicode[STIX]{x1D701}\geqslant 0$ , representing a dimensionless measure of the liquid momentum at the collapse point (Kelvin impulse). This parameter is rigorously defined for various jet drivers, including gravity and nearby boundaries. Combining theoretical considerations with hundreds of high-speed visualisations of bubbles collapsing near a rigid surface, near a free surface or in variable gravity, we classify the jets into three distinct regimes: weak, intermediate and strong. Weak jets ( 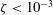 $\unicode[STIX]{x1D701}<10^{-3}$ ) hardly pierce the bubble, but remain within it throughout the collapse and rebound. Intermediate jets (
$\unicode[STIX]{x1D701}<10^{-3}$ ) hardly pierce the bubble, but remain within it throughout the collapse and rebound. Intermediate jets (  $10^{-3}<\unicode[STIX]{x1D701}<0.1$ ) pierce the opposite bubble wall close to the last collapse phase and clearly emerge during the rebound. Strong jets (
$10^{-3}<\unicode[STIX]{x1D701}<0.1$ ) pierce the opposite bubble wall close to the last collapse phase and clearly emerge during the rebound. Strong jets ( 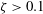 $\unicode[STIX]{x1D701}>0.1$ ) pierce the bubble early during the collapse. The dynamics of the jets is analysed through key observables, such as the jet impact time, jet speed, bubble displacement, bubble volume at jet impact and vapour-jet volume. We find that, upon normalising these observables to dimensionless jet parameters, they all reduce to straightforward functions of
$\unicode[STIX]{x1D701}>0.1$ ) pierce the bubble early during the collapse. The dynamics of the jets is analysed through key observables, such as the jet impact time, jet speed, bubble displacement, bubble volume at jet impact and vapour-jet volume. We find that, upon normalising these observables to dimensionless jet parameters, they all reduce to straightforward functions of 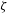 $\unicode[STIX]{x1D701}$ , which we can reproduce numerically using potential flow theory. An interesting consequence of this result is that a measurement of a single observable, such as the bubble displacement, suffices to estimate any other parameter, such as the jet speed. Remarkably, the dimensionless parameters of intermediate and weak jets (
$\unicode[STIX]{x1D701}$ , which we can reproduce numerically using potential flow theory. An interesting consequence of this result is that a measurement of a single observable, such as the bubble displacement, suffices to estimate any other parameter, such as the jet speed. Remarkably, the dimensionless parameters of intermediate and weak jets ( 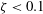 $\unicode[STIX]{x1D701}<0.1$ ) depend only on
$\unicode[STIX]{x1D701}<0.1$ ) depend only on 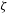 $\unicode[STIX]{x1D701}$ , not on the jet driver (i.e. gravity or boundaries). In the same regime, the jet parameters are found to be well approximated by power laws of
$\unicode[STIX]{x1D701}$ , not on the jet driver (i.e. gravity or boundaries). In the same regime, the jet parameters are found to be well approximated by power laws of  $\unicode[STIX]{x1D701}$ , which we explain through analytical arguments.
$\unicode[STIX]{x1D701}$ , which we explain through analytical arguments.
Information
- Type
- Papers
- Information
- Copyright
- © 2016 Cambridge University Press
References
Supponen et al. supplementary movie
Weak jet formation driven by gravity (see figure 4). The movie has been taken at 100 000 frames per second. The anisotropy parameter ζ equals 0.001.
Supponen et al. supplementary movie
Shock wave emission at the collapse of a bubble with a gravity-driven weak jet (see figure 5). The movie has been taken at 10 million frames per second. The anisotropy parameter ζ equals 0.001.
Supponen et al. supplementary movie
Bubble with an intermediate jet driven by gravity (see figure 6). The movie has been taken at 20 000 frames per second. The anisotropy parameter ζ equals 0.007.
- 237
- Cited by


