Barry, M., Ivey, G., Winters, K. & Imberger, J.
2001
Measurements of diapycnal diffusivities in stratified fluids. J. Fluid Mech.
442, 267–291.
Bartello, P.
1995
Geostrophic adjustment and inverse cascade in rotating stratified turbulence. J. Atmos. Sci.
52, 4410–4428.
Billant, P. & Chomaz, J. M.
2001
Self-similarity of strongly stratified inviscid flows. Phys. Fluids
13, 1645–1651.
Bluteau, C. E., Jones, N. L. & Ivey, G. N.
2013
Turbulent mixing efficiency at an energetic ocean site. J. Geophys. Res.
118, 1–11.
van Bokhoven, L. J. A., Clercx, H. J. H., van Heijst, G. J. F. & Trieling, R. R.
2009
Experiments on rapidly rotating turbulent flows. Phys. Fluids
21, 096601.
Bouffard, D. & Boegman, L.
2013
A diapycnal diffusivity model for stratified environmental flows. Dyn. Atmos. Oceans
61–62, 14–34.
Brethouwer, G., Billant, P., Lindborg, E. & Chomaz, J. M.
2007
Scaling analysis and simulation of strongly stratified turbulent flows. J. Fluid Mech.
585, 343–368.
de Bruyn Kops, S. M.
2015
Classical scaling and intermittency in strongly stratifed Boussinesq turbulence. J. Fluid Mech.
775, 436–463.
Cambon, C., Godeferd, F. S., Nicolleau, F. & Vassilicos, J. C.
2004
Turbulent diffusion in rapidly rotating flows with and without stable stratification. J. Fluid Mech.
499, 231–255.
Cambon, C. & Jacquin, L.
1989
Spectral approach to non-isotropic turbulence subjected to rotation. J. Fluid Mech.
202, 295–317.
D’Asaro, E., Lee, C., Rainville, L., Harcourt, R. & Thomas, L.
2011
Enhanced turbulence and energy dissipation at ocean fronts. Science
332, 318–322.
Davidson, P. A., Staplehurst, P. J. & Dalziel, S. B.
2006
On the evolution of eddies in a rapidly rotating system. J. Fluid Mech.
557, 135–144.
Davis, K. A. & Monismith, S. G.
2011
The modification of bottom boundary layer turbulence and mixing by internal waves shoaling on a barrier reef. J. Phys. Oceanogr.
41, 2223–2241.
de Lavergne, C., Madec, G., Le Sommier, J., Nurser, A. J. G. & Garabato, A. C. Naveira
2016
The impact of a variable mixing efficiency on the abyssal overturning. J. Phys. Ocean.
46, 663–681.
Dillon, T. M.
1982
Vertical overturns: A comparison of Thorpe and Ozmidov length scales. J. Geophys. Res.
87, 9601–9613.
Dimotakis, P. E.
2005
Turbulent mixing. Annu. Rev. Fluid Mech.
37, 329–356.
Dritschel, D. G. & McKiver, W. J.
2015
Effect of Prandtl’s ratio in geophysical turbulence. J. Fluid Mech.
777, 569–590.
Ferrari, R., Mashayek, A., McDougall, T. J., Nikurashin, M. & Campin, J. M.
2016
Turning ocean mixing upside down. J. Phys. Oceanogr.
46, 2239–2261.
Finnigan, J.
1999
A note on wave-turbulence interactions and the possibility of scaling the very stable planetary boundary layer. Boundary-Layer Meteorol.
90, 529–539.
Fleury, M. & Lueck, R. G.
1994
Direct heat flux estimates using a towed vehicle. J. Phys. Oceangr.
24, 801–818.
van Haren, H., Cimatoribus, A. A., Cyr, F. & Gostiaux, L.
2016
Insights from a 3-D temperature sensors mooring on stratified ocean turbulence. Geophys. Res. Lett.
43, 1–7.
Herbert, C., Marino, R., Pouquet, A. & Rosenberg, D.
2016
Waves and vortices in the inverse cascade regime of rotating stratified turbulence with or without rotation. J. Fluid Mech.
806, 165–204.
Herring, J. R.
1980
Statistical theory of quasi-geostrophic turbulence. J. Atmos. Sci.
37, 969–977.
Ishihara, T., Gotoh, T. & Kaneda, Y.
2009
Study of high Reynolds number isotropic turbulence by direct numerical simulation. Annu. Rev. Fluid Mech.
41, 165–180.
Ivey, G., Winters, K. & Koseff, J.
2008
Density stratification, turbulence but how much mixing?
Annu. Rev. Fluid Mech.
40, 169–184.
Iyer, K. P., Sreenivasan, K. R. & Yeung, P. K.
2017
Reynolds number scaling of velocity increments in isotropic turbulence. Phys. Rev. E
95, 021101(R).
Karimpour, F. & Venayagamoorthy, S. K.
2015
On turbulent mixing in stably stratified wall-bounded flows. Phys. Fluids
27, 046603.
Kimura, Y. & Herring, J. R.
1996
Diffusion in stably stratified turbulence. J. Fluid Mech.
328, 253–269.
Klymak, J. M., Pinkel, R. & Rainville, L.
2008
Direct breaking of the internal tide near topography: Kaena Ridge, Hawaii. J. Phys. Oceanogr.
38, 380–399.
Kurien, S. & Smith, L. M.
2014
Effect of rotation and domain aspect-ratio on layer formation in strongly stratified Boussinesq flows. J. Turbul.
15, 241–271.
Laval, J.-P., McWilliams, J. C. & Dubrulle, B.
2003
Forced stratified turbulence: Successive transitions with reynolds number. Phys. Rev. E
68, 036308.
Lelong, M.-P. & Riley, J. J.
1991
Internal wave-vortical mode interactions in strongly stratified flows. J. Fluid Mech.
232, 1–19.
Lelong, M.-P. & Sundermeyer, M.
2005
Geostrophic adjustment of an isolated diapycnal mixing event and its implications for small-scale lateral dispersion. J. Phys. Oceanogr.
35, 2352–2367.
Lindborg, E.
2006
The energy cascade in a strongly stratified fluid. J. Fluid Mech.
550, 207–242.
Lindborg, E. & Brethouwer, G.
2008
Vertical dispersion by stratified turbulence. J. Fluid Mech.
614, 303–314.
Liu, H. L., Yudin, V. & Roble, R.
2013
Day-to-day ionospheric variability due to lower atmosphere perturbations. Geophys. Res. Lett.
40, 665–670.
Lozovatsky, I. D. & Fernando, H. J. S.
2013
Mixing efficiency in natural flows. Phil. Trans. R. Soc. Lond. A
371, 20120213.
Luketina, D. & Imberger, J.
1989
Turbulence and entrainment in a buoyant surface plume. J. Geophys. Res.
94, 12619–12636.
Maffioli, A., Brethouwer, G. & Lindborg, E.
2016
Mixing efficiency in stratified turbulence. J. Fluid Mech.
794, R3.
Maffioli, A. & Davidson, P. A.
2016
Dynamics of stratified turbulence decaying from a high buoyancy Reynolds number. J. Fluid Mech.
786, 210–233.
Marino, R., Pouquet, A. & Rosenberg, D.
2015a
Resolving the paradox of oceanic large-scale balance and small-scale mixing. Phys. Rev. Lett.
114, 114504.
Marino, R., Rosenberg, D., Herbert, C. & Pouquet, A.
2015b
Interplay of waves and eddies in rotating stratified turbulence and the link with kinetic-potential energy partition. Eur. Phys. Lett.
112, 49001.
Mashayek, A. & Peltier, W. R.
2013
Shear-induced mixing in geophysical flows: does the route to turbulence matter to its efficiency?
J. Fluid Mech.
725, 216–261.
Mashayek, A., Salehipour, H., Bouffard, D., Caulfield, C. P., Ferrari, R., Nikurashin, M., Peltier, W. R. & Smyth, W. D.
2017
Efficiency of turbulent mixing in the abyssal ocean circulation. Geophys. Res. Lett.
44, 6296–6306.
Mater, B. D., Schaad, S. M. & Venayagamoorthy, S. K.
2013
Relevance of the Thorpe length scale in stably stratified turbulence. Phys. Fluids
25, 076604.
Mater, B. D. & Venayagamoorthy, S. K.
2014
The quest for an unambiguous parameterization of mixing efficiency in stably stratified geophysical flows. Geophys. Res. Lett.
41, 4646–4653.
McWilliams, J.
2016
Submesoscale currents in the ocean. Proc. R. Soc. Lond. A
472, 2016.0117.
Métais, O. & Herring, J.
1989
Numerical simulations of freely evolving turbulence in stably stratified fluids. J. Fluid Mech.
202, 117–148.
Mininni, P. D., Rosenberg, D. & Pouquet, A.
2012
Isotropization at small scale of rotating helically driven turbulence. J. Fluid Mech.
699, 263–279.
Mininni, P. D., Rosenberg, D., Reddy, R. & Pouquet, A.
2011
A hybrid MPI-OpenMP scheme for scalable parallel pseudospectral computations for fluid turbulence. Parallel Comput.
37, 316–326.
Monin, A. S. & Yaglom, A. M.
1979
Statistical Fluid Mechanics. MIT Press, Cambridge.
Oks, D., Mininni, P. D. & Pouquet, A.2018 Generation of turbulence through frontogenesis in sheared stratified flows. Phys. Fluids (submitted) arXiv:1706.10287v2.
Osborn, T. R.
1980
Estimates of the local rate of vertical diffusion from dissipation measurements. J. Phys. Oceanogr.
10, 83–89.
Paoli, R., Thouron, O., Escobar, J., Picot, J. & Cariolle, D.
2014
High-resolution large-eddy simulations of stably stratified flows: application to subkilometer-scale turbulence in the upper troposphere–lower stratosphere. Atm. Chem. Phys.
14, 5037–5055.
Patterson, M. D., Caulfield, C. P., McElwaine, J. N. & Dalziel, S. B.
2006
Time-dependent mixing in stratified Kelvin–Helmholtz billows: Experimental observations. Geophys. Res. Lett.
33, L15608.
Peltier, W. & Caulfield, C.
2003
Mixing efficiency in stratified shear flows. Annu. Rev. Fluid Mech.
35, 135–167.
Phillips, O. M.
1972
Turbulence in a strongly stratified fluid: Is it unstable?
Deep-Sea Res.
19, 79–81.
Pouquet, A. & Marino, R.
2013
Geophysical turbulence and the duality of the energy flow across scales. Phys. Rev. Lett.
111, 234501.
Pouquet, A., Marino, R., Mininni, P. D. & Rosenberg, D.
2017
Dual constant-flux energy cascades to both large scales and small scales. Phys. Fluids
29, 111108.
Praud, O., Sommeria, J. & Fincham, A.
2006
Decaying grid turbulence in a rotating stratified fluid. J. Fluid Mech.
547, 389–412.
Pumir, A., Xu, H. & Siggia, E. D.
2016
Small-scale anisotropy in turbulent boundary layers. J. Fluid Mech.
804, 5–23.
Riley, J. J. & deBruynKops, S. M.
2003
Dynamics of turbulence strongly influenced by buoyancy. Phys. Fluids
15, 2047–2059.
Rorai, C., Mininni, P. D. & Pouquet, A.
2014
Turbulence comes in bursts in stably stratified flows. Phys. Rev. E
89, 043002.
Rosenberg, D., Marino, R., Herbert, C. & Pouquet, A.
2016
Variations of characteristic time-scales in rotating stratified turbulence using a large parametric numerical study. Eur. Phys. J. E
39, 8.
Rosenberg, D., Marino, R., Herbert, C. & Pouquet, A.
2017
Correction to: Variations of characteristic time scales in rotating stratified turbulence using a large parametric numerical study. Eur. Phys. J. E
40, 87.
Rosenberg, D., Pouquet, A., Marino, R. & Mininni, P. D.
2015
Evidence for Bolgiano–Obukhov scaling in rotating stratified turbulence using high-resolution direct numerical simulations. Phys. Fluids
27, 055105.
Rubinstein, R., Clark, T. T. & Kurien, S.
2017
Leith diffusion model for homogeneous anisotropic turbulence. Comput. Fluids
151, 108–114.
Salehipour, H. & Peltier, W. R.
2015
Diapycnal diffusivity, turbulent Prandtl number and mixing efficiency in Boussinesq stratified turbulence. J. Fluid Mech.
775, 464–500.
Shih, L., Koseff, J., Ivey, G. & Ferziger, J.
2005
Parameterization of turbulent fluxes and scales using homogeneous sheared stably stratified turbulence simulations. J. Fluid Mech.
525, 193–214.
Smyth, W. D., Moum, J. N. & Caldwell, D. R.
2001
The efficiency of mixing in turbulent patches: Inferences from direct simulations and microstructure observations. J. Phys. Oceanogr.
31, 1969–1992.
Sozza, A., Boffetta, G., Muratore-Ginanneschi, P. & Musacchio, S.
2015
Dimensional transition of energy cascades in stably stratified forced thin fluid layers. Phys. Fluids
27, 035112.
Stacey, M., Monismith, S. & Burau, J.
1999
Observations of turbulence in a partially stratified estuary. J. Phys. Oceanogr.
29, 1950–1970.
Staquet, C. & Sommeria, J.
2002
Internal gravity waves: From instabilities to turbulence. Annu. Rev. Fluid Mech.
34, 559–593.
Stillinger, D., Helland, K. & van Atta, C.
1983
Experiments on the transition of homogeneous turbulence to internal waves in a stratified fluid. J. Fluid Mech.
131, 91–122.
Stretch, D. D., Rottman, J., Venayagamoorthy, S. K., Nomura, K. & Rehmann, C. R.
2010
Mixing efficiency in decaying stably stratified turbulence. Dyn. Atmos. Oceans
49, 25–36.
Sukoriansky, S., Galperin, B. & Staroselsky, I.
2005
A quasinormal scale elimination model of turbulent flows with stable stratification. Phys. Fluids
17, 085107.
Thorpe, S. A.
1987
Transitional phenomena and the development of turbulence in stratified fluids: a review. J. Geophys. Res.
92, 5231–5248.
Venayagamoorthy, S. K. & Koseff, J. R.
2016
On the flux Richardson number in stably stratified turbulence. J. Fluid Mech.
798, R1–R10.
Waite, M. & Bartello, P.
2006
The transition from geostrophic to stratified turbulence. J. Fluid Mech.
568, 89–108.
Wells, M., Cenedese, C. & Caulfield, C. P.
2010
The relationship between flux coefficient and entrainment ratio in density currents. J. Phys. Oceanogr.
40, 2713–2727.
Zakharov, V. E., L’vov, V. S. & Falkovich, G.
1992
Kolmogorov spectra of turbulence: Wave turbulence. In Non-Linear Dynamics, Springer.
Zilitinkevich, S. S., Elperin, T., Kleeorin, N., Rogachevskii, I. & Esau, I.
2013
A hierarchy of energy- and flux-budget (EFB) turbulence closure models for stably-stratified geophysical flows. Boundary-Layer Meteorol.
146, 341–373.
Zilitinkevich, S. S., Elperin, T., Kleeorin, N., Rogachevskii, I., Esau, I., Mauritsen, T. & Miles, M. W.
2008
Turbulence energetics in stably stratified geophysical flows: Strong and weak mixing regimes. Q. J. R. Meteorol. Soc.
134, 793–799.
$Ro,Fr$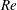
,
$Re$
. This model is based on quasi-equipartition between kinetic and potential modes, sub-dominant vertical velocity,
$w$
, and lessening of the energy transfer to small scales as measured by a dissipation efficiency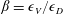
$\unicode[STIX]{x1D6FD}=\unicode[STIX]{x1D716}_{V}/\unicode[STIX]{x1D716}_{D}$
, with
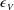$\unicode[STIX]{x1D716}_{V}$
the kinetic energy dissipation and
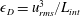$\unicode[STIX]{x1D716}_{D}=u_{rms}^{3}/L_{int}$
its dimensional expression, with
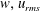$w,u_{rms}$
the vertical and root mean square velocities, and
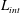$L_{int}$
the integral scale. We determine the domains of validity of such laws for a large numerical study of the unforced Boussinesq equations mostly on grids of
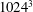$1024^{3}$
points, with
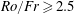$Ro/Fr\geqslant 2.5$
, and with
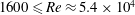$1600\leqslant Re\approx 5.4\times 10^{4}$
; the Prandtl number is one, initial conditions are either isotropic and at large scale for the velocity and zero for the temperature
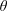$\unicode[STIX]{x1D703}$
, or in geostrophic balance. Three regimes in Froude number, as for stratified flows, are observed: dominant waves, eddy–wave interactions and strong turbulence. A wave–turbulence balance for the transfer time
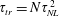$\unicode[STIX]{x1D70F}_{tr}=N\unicode[STIX]{x1D70F}_{NL}^{2}$
, with
$\unicode[STIX]{x1D70F}_{NL}=L_{int}/u_{rms}$
the turnover time and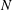
$N$
the Brunt–Väisälä frequency, leads to
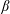$\unicode[STIX]{x1D6FD}$
growing linearly with
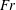$Fr$
in the intermediate regime, with a saturation at
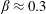$\unicode[STIX]{x1D6FD}\approx 0.3$
or more, depending on initial conditions for larger Froude numbers. The Ellison scale is also found to scale linearly with
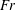$Fr$
. The flux Richardson number
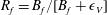$R_{f}=B_{f}/[B_{f}+\unicode[STIX]{x1D716}_{V}]$
, with
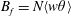$B_{f}=N\langle w\unicode[STIX]{x1D703}\rangle$
the buoyancy flux, transitions for approximately the same parameter values as for
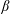$\unicode[STIX]{x1D6FD}$
. These regimes for the present study are delimited by
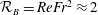${\mathcal{R}}_{B}=ReFr^{2}\approx 2$
and
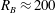$R_{B}\approx 200$
. With
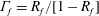$\unicode[STIX]{x1D6E4}_{f}=R_{f}/[1-R_{f}]$
the mixing efficiency, putting together the three relationships of the model allows for the prediction of the scaling
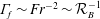$\unicode[STIX]{x1D6E4}_{f}\sim Fr^{-2}\sim {\mathcal{R}}_{B}^{-1}$
in the low and intermediate regimes for high
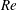$Re$
, whereas for higher Froude numbers,
$\unicode[STIX]{x1D6E4}_{f}\sim {\mathcal{R}}_{B}^{-1/2}$
, a scaling already found in observations: as turbulence strengthens,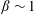
$\unicode[STIX]{x1D6FD}\sim 1$
,
$w\approx u_{rms}$
, and smaller buoyancy fluxes together correspond to a decoupling of velocity and temperature fluctuations, the latter becoming passive.
