Article contents
Suspension flow past a cylinder: particle interactions with recirculating wakes
Published online by Cambridge University Press: 10 November 2014
Abstract
Experimental observations of the flow of a suspension of solid fraction 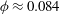 ${\it\phi}\approx 0.084$ over a circular cylindrical post in a shallow microchannel (depth smaller than the cylinder radius) find that the recirculating wake behind the obstacle at moderate Reynolds numbers is depleted or devoid of particles. Particles injected into the wake exit to regain the depleted state. By numerical simulation of the discrete particle motion, the basis for the depletion behind the cylinder is studied; rather than a shallow channel, the numerical simulations consider a periodic domain, mimicking the flow past an infinite cylinder. The Reynolds number is defined, using the average axial velocity
${\it\phi}\approx 0.084$ over a circular cylindrical post in a shallow microchannel (depth smaller than the cylinder radius) find that the recirculating wake behind the obstacle at moderate Reynolds numbers is depleted or devoid of particles. Particles injected into the wake exit to regain the depleted state. By numerical simulation of the discrete particle motion, the basis for the depletion behind the cylinder is studied; rather than a shallow channel, the numerical simulations consider a periodic domain, mimicking the flow past an infinite cylinder. The Reynolds number is defined, using the average axial velocity  ${\bar{U}}$ , diameter of the obstacle
${\bar{U}}$ , diameter of the obstacle 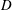 $D$ and the kinematic viscosity of the suspension
$D$ and the kinematic viscosity of the suspension  ${\it\nu}$ , as
${\it\nu}$ , as 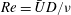 $Re={\bar{U}}D/{\it\nu}$ , and is studied for
$Re={\bar{U}}D/{\it\nu}$ , and is studied for 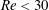 $Re<30$ in the simulation – conditions for which the pure fluid exhibits an extended steady closed-streamline (recirculating) wake behind the cylinder; unsteadiness is found to be suppressed by the channel walls in the experiments, allowing steady flow at a larger
$Re<30$ in the simulation – conditions for which the pure fluid exhibits an extended steady closed-streamline (recirculating) wake behind the cylinder; unsteadiness is found to be suppressed by the channel walls in the experiments, allowing steady flow at a larger 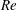 $Re$ than expected for an infinite cylinder (up to at least
$Re$ than expected for an infinite cylinder (up to at least 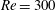 $Re=300$ ). The simulations use the lattice-Boltzmann method to determine the motion of the fluid and neutrally buoyant particles. The trajectory of a single particle (small relative to the cylinder) shows migration to a limit cycle inside the wake. With an increase of the number of particles in the wake alone (no particles in the free stream), particles can escape the wake due to velocity fluctuations. Simulation of the flow of suspensions of
$Re=300$ ). The simulations use the lattice-Boltzmann method to determine the motion of the fluid and neutrally buoyant particles. The trajectory of a single particle (small relative to the cylinder) shows migration to a limit cycle inside the wake. With an increase of the number of particles in the wake alone (no particles in the free stream), particles can escape the wake due to velocity fluctuations. Simulation of the flow of suspensions of  ${\it\phi}=0.04,0.06$ and 0.08 demonstrates that there is particle exchange between the wake and the free stream; the net flux of particles out of the wake leads to a particle-depleted wake, qualitatively very similar to the experimental observation.
${\it\phi}=0.04,0.06$ and 0.08 demonstrates that there is particle exchange between the wake and the free stream; the net flux of particles out of the wake leads to a particle-depleted wake, qualitatively very similar to the experimental observation.
Information
- Type
- Rapids
- Information
- Copyright
- © 2014 Cambridge University Press
References
Haddadi et al. supplementary movie
A single particle released in the free stream does not enter the wake region (δ = 0.09, Re = 18).
Haddadi et al. supplementary movie
A single particle released in the wake region migrates outward and reaches a limit cycle trajectory. (δ = 0.09, Re = 18).
- 36
- Cited by


