Article contents
A tent model of vortex reconnection under Biot–Savart evolution
Published online by Cambridge University Press: 17 November 2017
Abstract
Vortex reconnection under Biot–Savart evolution is investigated geometrically and numerically using a tent model consisting of vortex filaments initially in the form of two tilted hyperbolic branches; the vortices are antiparallel at their points of nearest approach. It is shown that the tips of these vortices approach each other, accelerating as they do so to form a finite-time singularity at the apex of the tent. The minimum separation of the vortices and the maximum velocity and axial strain rate exhibit nearly self-similar Leray scaling, but the exponents of the velocity and strain rate deviate slightly from their respective self-similar values of 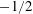 $-1/2$ and
$-1/2$ and 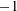 $-1$ ; this deviation is associated with the appearance of distinct minima of curvature leading to cusp structures at the tips. The writhe and twist of each vortex are both zero at all times up to the instant of reconnection. By way of validation of the model, the structure of the eigenvalues and eigenvectors of the rate-of-strain tensor is investigated: it is shown that the second eigenvalue
$-1$ ; this deviation is associated with the appearance of distinct minima of curvature leading to cusp structures at the tips. The writhe and twist of each vortex are both zero at all times up to the instant of reconnection. By way of validation of the model, the structure of the eigenvalues and eigenvectors of the rate-of-strain tensor is investigated: it is shown that the second eigenvalue 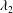 $\unicode[STIX]{x1D706}_{2}$ has dipole structure around the vortex filaments. At the tips, it is observed that
$\unicode[STIX]{x1D706}_{2}$ has dipole structure around the vortex filaments. At the tips, it is observed that 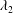 $\unicode[STIX]{x1D706}_{2}$ is positive and the corresponding eigenvector is tangent to the filament, implying persistent stretching of the vortex.
$\unicode[STIX]{x1D706}_{2}$ is positive and the corresponding eigenvector is tangent to the filament, implying persistent stretching of the vortex.
Information
- Type
- JFM Rapids
- Information
- Copyright
- © 2017 Cambridge University Press
References
- 12
- Cited by


