Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Moffatt, H. K.
2019.
Singularities in fluid mechanics.
Physical Review Fluids,
Vol. 4,
Issue. 11,
Yao, Jie
and
Hussain, Fazle
2020.
A physical model of turbulence cascade via vortex reconnection sequence and avalanche.
Journal of Fluid Mechanics,
Vol. 883,
Issue. ,
Moffatt, H. K.
and
Kimura, Yoshifumi
2020.
Towards a finite-time singularity of the Navier–Stokes equations. Part 2. Vortex reconnection and singularity evasion – CORRIGENDUM.
Journal of Fluid Mechanics,
Vol. 887,
Issue. ,
Kang, Di
Yun, Dongfang
and
Protas, Bartosz
2020.
Maximum amplification of enstrophy in three-dimensional Navier–Stokes flows.
Journal of Fluid Mechanics,
Vol. 893,
Issue. ,
Yao, Jie
and
Hussain, Fazle
2020.
On singularity formation via viscous vortex reconnection.
Journal of Fluid Mechanics,
Vol. 888,
Issue. ,
Josserand, Christophe
Pomeau, Yves
and
Rica, Sergio
2020.
Finite-time localized singularities as a mechanism for turbulent dissipation.
Physical Review Fluids,
Vol. 5,
Issue. 5,
Yao, Jie
and
Hussain, Fazle
2020.
Separation scaling for viscous vortex reconnection.
Journal of Fluid Mechanics,
Vol. 900,
Issue. ,
Zhao, Xinran
and
Scalo, Carlo
2021.
Helicity dynamics in reconnection events of topologically complex vortex flows.
Journal of Fluid Mechanics,
Vol. 920,
Issue. ,
Childress, Stephen
and
Gilbert, Andrew D
2021.
Area waves on a slender vortex revisited.
Fluid Dynamics Research,
Vol. 53,
Issue. 3,
p.
035508.
Moffatt, H.K.
2021.
Some topological aspects of fluid dynamics.
Journal of Fluid Mechanics,
Vol. 914,
Issue. ,
Hu, J.
and
Peterson, S. D.
2021.
The influence of collision angle for viscous vortex reconnection.
Physics of Fluids,
Vol. 33,
Issue. 9,
Mishra, Aakash
Pumir, Alain
and
Ostilla-Mónico, Rodolfo
2021.
Instability and disintegration of vortex rings during head-on collisions and wall interactions.
Physical Review Fluids,
Vol. 6,
Issue. 10,
Yao, Jie
and
Hussain, Fazle
2021.
Polarized vortex reconnection.
Journal of Fluid Mechanics,
Vol. 922,
Issue. ,
Yao, Jie
and
Hussain, Fazle
2022.
Vortex Reconnection and Turbulence Cascade.
Annual Review of Fluid Mechanics,
Vol. 54,
Issue. 1,
p.
317.
Protas, Bartosz
2022.
Systematic search for extreme and singular behaviour in some fundamental models of fluid mechanics.
Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 380,
Issue. 2225,
Faller, Hugues
Fery, Lucas
Geneste, Damien
and
Dubrulle, Bérengère
2022.
A Model of Interacting Navier–Stokes Singularities.
Entropy,
Vol. 24,
Issue. 7,
p.
897.
Dou, Hua-Shu
2022.
Origin of Turbulence.
p.
105.
Dubrulle, Bérengère
2022.
Multi-Fractality, Universality and Singularity in Turbulence.
Fractal and Fractional,
Vol. 6,
Issue. 10,
p.
613.
Kang, Di
and
Protas, Bartosz
2022.
Searching for Singularities in Navier–Stokes Flows Based on the Ladyzhenskaya–Prodi–Serrin Conditions.
Journal of Nonlinear Science,
Vol. 32,
Issue. 6,
Dou, Hua-Shu
2022.
No Existence and Smoothness of Solution of the Navier-Stokes Equation.
Entropy,
Vol. 24,
Issue. 3,
p.
339.
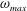 $\unicode[STIX]{x1D714}_{max}$ as a function of vortex Reynolds number
$\unicode[STIX]{x1D714}_{max}$ as a function of vortex Reynolds number 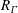 $R_{\unicode[STIX]{x1D6E4}}$ in the range
$R_{\unicode[STIX]{x1D6E4}}$ in the range 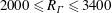 $2000\leqslant R_{\unicode[STIX]{x1D6E4}}\leqslant 3400$, and deduce a compatible behaviour
$2000\leqslant R_{\unicode[STIX]{x1D6E4}}\leqslant 3400$, and deduce a compatible behaviour 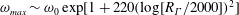 $\unicode[STIX]{x1D714}_{max}\sim \unicode[STIX]{x1D714}_{0}\exp [1+220(\log [R_{\unicode[STIX]{x1D6E4}}/2000])^{2}]$ as
$\unicode[STIX]{x1D714}_{max}\sim \unicode[STIX]{x1D714}_{0}\exp [1+220(\log [R_{\unicode[STIX]{x1D6E4}}/2000])^{2}]$ as 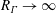 $R_{\unicode[STIX]{x1D6E4}}\rightarrow \infty$. This may be described as a physical (although not strictly mathematical) singularity, for all
$R_{\unicode[STIX]{x1D6E4}}\rightarrow \infty$. This may be described as a physical (although not strictly mathematical) singularity, for all 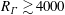 $R_{\unicode[STIX]{x1D6E4}}\gtrsim 4000$.
$R_{\unicode[STIX]{x1D6E4}}\gtrsim 4000$.
