Article contents
Waves from an oscillating point source with a free surface in the presence of a shear current
Published online by Cambridge University Press: 31 May 2016
Abstract
We investigate analytically the linearised water wave radiation problem for an oscillating submerged point source in an inviscid shear flow with a free surface. A constant depth is taken into account and the shear flow increases linearly with depth. The surface velocity relative to the source is taken to be zero, so that Doppler effects are absent. We solve the linearised Euler equations to calculate the resulting wave field as well as its far-field asymptotics. For values of the Froude number 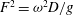 $F^{2}={\it\omega}^{2}D/g$ (where
$F^{2}={\it\omega}^{2}D/g$ (where 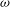 ${\it\omega}$ is the oscillation frequency,
${\it\omega}$ is the oscillation frequency, 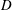 $D$ is the submergence depth and
$D$ is the submergence depth and 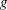 $g$ is the gravitational acceleration) below a resonant value
$g$ is the gravitational acceleration) below a resonant value  $F_{res}^{2}$ , the wave field splits cleanly into separate contributions from regular dispersive propagating waves and non-dispersive ‘critical waves’ resulting from a critical layer-like street of flow structures directly downstream of the source. In the subresonant regime, the regular waves behave like sheared ring waves, while the critical layer wave forms a street with a constant width of order
$F_{res}^{2}$ , the wave field splits cleanly into separate contributions from regular dispersive propagating waves and non-dispersive ‘critical waves’ resulting from a critical layer-like street of flow structures directly downstream of the source. In the subresonant regime, the regular waves behave like sheared ring waves, while the critical layer wave forms a street with a constant width of order 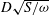 $D\sqrt{S/{\it\omega}}$ (where
$D\sqrt{S/{\it\omega}}$ (where 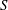 $S$ is the shear flow vorticity) and is convected downstream at the fluid velocity at the depth of the source. When the Froude number approaches its resonant value, the downstream critical and regular waves resonate, producing a train of waves of linearly increasing amplitude contained within a downstream wedge.
$S$ is the shear flow vorticity) and is convected downstream at the fluid velocity at the depth of the source. When the Froude number approaches its resonant value, the downstream critical and regular waves resonate, producing a train of waves of linearly increasing amplitude contained within a downstream wedge.
Information
- Type
- Papers
- Information
- Copyright
- © 2016 Cambridge University Press
References
Ellingsen and Tyvand supplementary movie
Movie animation of wave pattern from top panel of Figure 5 or article. With a low value of sigma, the wave pattern is completely dominated by the regular ring wave which is only slightly asymmetrical.
Ellingsen and Tyvand supplementary movie
Movie animation of wave pattern from Figure 5b of article. With sigma=0.5, a critical layer "wave" is clearly visible, and the regular waves are visibly sheared. Flow is from right to left while the surface is at rest.
Ellingsen and Tyvand supplementary movie
Movie animation of wave pattern from Figure 5c of article. An even higher value of sigma gives a more prominent critical layer and a more strongly sheared pattern of regular ring waves. Flow is from right to left while the surface is at rest.
Ellingsen and Tyvand supplementary movie
The far-field "critical waves" shown in Figure 7 of article, for increasing values of sigma. See main article for more information and discussion.
- 6
- Cited by
Linked content
Please note that this article has a (1).


