Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Ivanov, Alexandre A.
and
Macpherson, Dugald
1999.
Strongly determined types.
Annals of Pure and Applied Logic,
Vol. 99,
Issue. 1-3,
p.
197.
Kulpeshov, Beibut Sh.
and
Macpherson, H. Dugald
2005.
Minimality conditions on circularly ordered structures.
MLQ,
Vol. 51,
Issue. 4,
p.
377.
Guzy, Nicolas
2005.
p-Convexly valued rings.
Journal of Pure and Applied Algebra,
Vol. 199,
Issue. 1-3,
p.
111.
CLUCKERS, RAF
and
LOESER, FRANÇOIS
2007.
b-MINIMALITY.
Journal of Mathematical Logic,
Vol. 07,
Issue. 02,
p.
195.
Dolich, Alfred
Miller, Chris
and
Steinhorn, Charles
2009.
Structures having o-minimal open core.
Transactions of the American Mathematical Society,
Vol. 362,
Issue. 3,
p.
1371.
Mourgues, Marie‐Hélène
2009.
Cell decomposition for P‐minimal fields.
Mathematical Logic Quarterly,
Vol. 55,
Issue. 5,
p.
487.
Dolich, Alfred
Goodrick, John
and
Lippel, David
2011.
Dp-Minimality: Basic Facts and Examples.
Notre Dame Journal of Formal Logic,
Vol. 52,
Issue. 3,
Chatzidakis, Zoé
2011.
Motivic Integration and its Interactions with Model Theory and Non-Archimedean Geometry.
p.
35.
Leenknegt, Eva
2012.
Cell decomposition and definable functions for weak p‐adic structures.
Mathematical Logic Quarterly,
Vol. 58,
Issue. 6,
p.
482.
Leenknegt, Eva
2013.
Cell decomposition for semibounded p-adic sets.
Archive for Mathematical Logic,
Vol. 52,
Issue. 5-6,
p.
667.
Leenknegt, Eva
2014.
Reducts of p-adically closed fields.
Archive for Mathematical Logic,
Vol. 53,
Issue. 3-4,
p.
285.
Kuijpers, Tristan
2015.
Lipschitz extensions of definable p‐adic functions.
Mathematical Logic Quarterly,
Vol. 61,
Issue. 3,
p.
151.
Aschenbrenner, Matthias
Dolich, Alf
Haskell, Deirdre
Macpherson, Dugald
and
Starchenko, Sergei
2015.
Vapnik-Chervonenkis density in some theories without the independence property, I.
Transactions of the American Mathematical Society,
Vol. 368,
Issue. 8,
p.
5889.
Macpherson, Dugald
and
Tent, Katrin
2016.
Profinite groups with NIP theory andp-adic analytic groups.
Bulletin of the London Mathematical Society,
Vol. 48,
Issue. 6,
p.
1037.
Sinclair, Peter
2016.
Computable axiomatizability of elementary classes.
Mathematical Logic Quarterly,
Vol. 62,
Issue. 1-2,
p.
46.
Montenegro, Samaria
2017.
Pseudo real closed fields, pseudo p-adically closed fields and NTP2.
Annals of Pure and Applied Logic,
Vol. 168,
Issue. 1,
p.
191.
Chambille, Saskia
Cubides Kovacsics, Pablo
and
Leenknegt, Eva
2017.
Clustered cell decomposition in P-minimal structures.
Annals of Pure and Applied Logic,
Vol. 168,
Issue. 11,
p.
2050.
Thamrongthanyalak, Athipat
2017.
Linear extension operators for continuous functions on definable sets in the p‐adic context.
Mathematical Logic Quarterly,
Vol. 63,
Issue. 1-2,
p.
104.
Cluckers, Raf
and
Martin, Florent
2018.
A DEFINABLE -ADIC ANALOGUE OF KIRSZBRAUN’S THEOREM ON EXTENSIONS OF LIPSCHITZ MAPS.
Journal of the Institute of Mathematics of Jussieu,
Vol. 17,
Issue. 1,
p.
39.
Darnière, Luck
2019.
Model completion of scaled lattices and co‐Heyting algebras of p‐adic semi‐algebraic sets.
Mathematical Logic Quarterly,
Vol. 65,
Issue. 3,
p.
305.
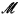 + is an L+-structure whose reduct to L is
+ is an L+-structure whose reduct to L is 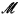 . Then
. Then 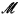 + is said to be
+ is said to be 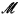 -minimal if, for every N+ elementarily equivalent to
-minimal if, for every N+ elementarily equivalent to 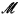 +, every parameterdefinable subset of its domain N+ is definable with parameters by a quantifier-free L-formula. Observe that if L has a single binary relation which in
+, every parameterdefinable subset of its domain N+ is definable with parameters by a quantifier-free L-formula. Observe that if L has a single binary relation which in 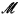 is interpreted by a total order on M, then we have just the notion of strong o-minimality, from [13]; and by a theorem from [6], strong o-minimality is equivalent to o-minimality. If L has no relations, functions, or constants (other than equality) then the notion is just strong minimality.
is interpreted by a total order on M, then we have just the notion of strong o-minimality, from [13]; and by a theorem from [6], strong o-minimality is equivalent to o-minimality. If L has no relations, functions, or constants (other than equality) then the notion is just strong minimality.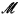 -minimality is investigated for a number of structures
-minimality is investigated for a number of structures 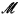 . In particular, the C-relation of [1] was considered, in place of the total order in the definition of strong o-minimality. The C-relation is essentially the ternary relation which naturally holds on the maximal chains of a sufficiently nice tree; see [1], [11] or [5] for more detail, and for axioms. Much of the motivation came from the observation that a C-relation on a field F which is preserved by the affine group AGL(1,F) (consisting of permutations (a,b) : x ↦ ax + b, where a ∈ F \ {0} and b ∈ F) is the same as a non-trivial valuation: to get a C-relation from a valuation ν, put C(x;y,z) if and only if ν(y − x) < ν(y − z).
. In particular, the C-relation of [1] was considered, in place of the total order in the definition of strong o-minimality. The C-relation is essentially the ternary relation which naturally holds on the maximal chains of a sufficiently nice tree; see [1], [11] or [5] for more detail, and for axioms. Much of the motivation came from the observation that a C-relation on a field F which is preserved by the affine group AGL(1,F) (consisting of permutations (a,b) : x ↦ ax + b, where a ∈ F \ {0} and b ∈ F) is the same as a non-trivial valuation: to get a C-relation from a valuation ν, put C(x;y,z) if and only if ν(y − x) < ν(y − z).