Article contents
AN EQUIDISTRIBUTION THEOREM FOR HOLOMORPHIC SIEGEL MODULAR FORMS FOR  $\mathit{GSp}_{4}$ AND ITS APPLICATIONS
$\mathit{GSp}_{4}$ AND ITS APPLICATIONS
Published online by Cambridge University Press: 22 February 2018
Abstract
We prove an equidistribution theorem for a family of holomorphic Siegel cusp forms for 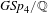 $\mathit{GSp}_{4}/\mathbb{Q}$ in various aspects. A main tool is Arthur’s invariant trace formula. While Shin [Automorphic Plancherel density theorem, Israel J. Math.192(1) (2012), 83–120] and Shin–Templier [Sato–Tate theorem for families and low-lying zeros of automorphic
$\mathit{GSp}_{4}/\mathbb{Q}$ in various aspects. A main tool is Arthur’s invariant trace formula. While Shin [Automorphic Plancherel density theorem, Israel J. Math.192(1) (2012), 83–120] and Shin–Templier [Sato–Tate theorem for families and low-lying zeros of automorphic  $L$-functions, Invent. Math.203(1) (2016) 1–177] used Euler–Poincaré functions at infinity in the formula, we use a pseudo-coefficient of a holomorphic discrete series to extract holomorphic Siegel cusp forms. Then the non-semisimple contributions arise from the geometric side, and this provides new second main terms
$L$-functions, Invent. Math.203(1) (2016) 1–177] used Euler–Poincaré functions at infinity in the formula, we use a pseudo-coefficient of a holomorphic discrete series to extract holomorphic Siegel cusp forms. Then the non-semisimple contributions arise from the geometric side, and this provides new second main terms  $A,B_{1}$ in Theorem 1.1 which have not been studied and a mysterious second term
$A,B_{1}$ in Theorem 1.1 which have not been studied and a mysterious second term  $B_{2}$ also appears in the second main term coming from the semisimple elements. Furthermore our explicit study enables us to treat more general aspects in the weight. We also give several applications including the vertical Sato–Tate theorem, the unboundedness of Hecke fields and low-lying zeros for degree 4 spinor
$B_{2}$ also appears in the second main term coming from the semisimple elements. Furthermore our explicit study enables us to treat more general aspects in the weight. We also give several applications including the vertical Sato–Tate theorem, the unboundedness of Hecke fields and low-lying zeros for degree 4 spinor  $L$-functions and degree 5 standard
$L$-functions and degree 5 standard  $L$-functions of holomorphic Siegel cusp forms.
$L$-functions of holomorphic Siegel cusp forms.
MSC classification
Information
- Type
- Research Article
- Information
- Journal of the Institute of Mathematics of Jussieu , Volume 19 , Issue 2 , March 2020 , pp. 351 - 419
- Copyright
- © Cambridge University Press 2018
Footnotes
The first author is partially supported by NSERC. The second author is partially supported by JSPS Grant-in-Aid for Scientific Research (nos. 26800006, 25247001, 15K04795). The third author is partially supported by JSPS Grant-in-Aid for Scientific Research (C) no. 15K04787.
References
- 8
- Cited by

