Published online by Cambridge University Press: 18 July 2019
We prove that the integral closure of a strongly Golod ideal in a polynomial ring over a field of characteristic zero is strongly Golod, positively answering a question of Huneke. More generally, the rational power 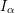 $I_{\unicode[STIX]{x1D6FC}}$ of an arbitrary homogeneous ideal is strongly Golod for
$I_{\unicode[STIX]{x1D6FC}}$ of an arbitrary homogeneous ideal is strongly Golod for  $\unicode[STIX]{x1D6FC}\geqslant 2$ and, if
$\unicode[STIX]{x1D6FC}\geqslant 2$ and, if 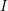 $I$ is strongly Golod, then
$I$ is strongly Golod, then 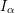 $I_{\unicode[STIX]{x1D6FC}}$ is strongly Golod for
$I_{\unicode[STIX]{x1D6FC}}$ is strongly Golod for 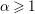 $\unicode[STIX]{x1D6FC}\geqslant 1$. We also show that all the coefficient ideals of a strongly Golod ideal are strongly Golod.
$\unicode[STIX]{x1D6FC}\geqslant 1$. We also show that all the coefficient ideals of a strongly Golod ideal are strongly Golod.