Article contents
On the notions of upper and lower density
Published online by Cambridge University Press: 30 August 2019
Abstract
Let 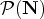 $\mathcal {P}(\mathbf{N})$ be the power set of N. We say that a function
$\mathcal {P}(\mathbf{N})$ be the power set of N. We say that a function 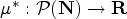 $\mu ^\ast : \mathcal {P}(\mathbf{N}) \to \mathbf{R}$ is an upper density if, for all X, Y ⊆ N and h, k ∈ N+, the following hold: (f1)
$\mu ^\ast : \mathcal {P}(\mathbf{N}) \to \mathbf{R}$ is an upper density if, for all X, Y ⊆ N and h, k ∈ N+, the following hold: (f1)  $\mu ^\ast (\mathbf{N}) = 1$; (f2)
$\mu ^\ast (\mathbf{N}) = 1$; (f2) 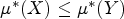 $\mu ^\ast (X) \le \mu ^\ast (Y)$ if X ⊆ Y; (f3)
$\mu ^\ast (X) \le \mu ^\ast (Y)$ if X ⊆ Y; (f3)  $\mu ^\ast (X \cup Y) \le \mu ^\ast (X) + \mu ^\ast (Y)$; (f4)
$\mu ^\ast (X \cup Y) \le \mu ^\ast (X) + \mu ^\ast (Y)$; (f4) 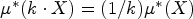 $\mu ^\ast (k\cdot X) = ({1}/{k}) \mu ^\ast (X)$, where k · X : = {kx: x ∈ X}; and (f5)
$\mu ^\ast (k\cdot X) = ({1}/{k}) \mu ^\ast (X)$, where k · X : = {kx: x ∈ X}; and (f5)  $\mu ^\ast (X + h) = \mu ^\ast (X)$. We show that the upper asymptotic, upper logarithmic, upper Banach, upper Buck, upper Pólya and upper analytic densities, together with all upper α-densities (with α a real parameter ≥ −1), are upper densities in the sense of our definition. Moreover, we establish the mutual independence of axioms (f1)–(f5), and we investigate various properties of upper densities (and related functions) under the assumption that (f2) is replaced by the weaker condition that
$\mu ^\ast (X + h) = \mu ^\ast (X)$. We show that the upper asymptotic, upper logarithmic, upper Banach, upper Buck, upper Pólya and upper analytic densities, together with all upper α-densities (with α a real parameter ≥ −1), are upper densities in the sense of our definition. Moreover, we establish the mutual independence of axioms (f1)–(f5), and we investigate various properties of upper densities (and related functions) under the assumption that (f2) is replaced by the weaker condition that 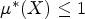 $\mu ^\ast (X)\le 1$ for every X ⊆ N. Overall, this allows us to extend and generalize results so far independently derived for some of the classical upper densities mentioned above, thus introducing a certain amount of unification into the theory.
$\mu ^\ast (X)\le 1$ for every X ⊆ N. Overall, this allows us to extend and generalize results so far independently derived for some of the classical upper densities mentioned above, thus introducing a certain amount of unification into the theory.
Keywords
Information
- Type
- Research Article
- Information
- Proceedings of the Edinburgh Mathematical Society , Volume 63 , Issue 1 , February 2020 , pp. 139 - 167
- Copyright
- Copyright © Edinburgh Mathematical Society 2019
Footnotes
Current address: Institute of Analysis and Number Theory, Graz University of Technology, Kopernikusgasse 24/II, 8010 Graz, Austria.
Current address: College of Mathematics and Information Science, Hebei Normal University, Shijiazhuang, Hebei Province 050000, China.
References
- 20
- Cited by

