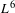 $L^{6}$ FRAMEWORK
$L^{6}$ FRAMEWORKPublished online by Cambridge University Press: 29 June 2017
Ill-posedness for the compressible Navier–Stokes equations has been proved by Chen et al. [On the ill-posedness of the compressible Navier–Stokes equations in the critical Besov spaces, Revista Mat. Iberoam.31 (2015), 1375–1402] in critical Besov space 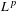 $L^{p}$
$L^{p}$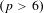 $(p>6)$ framework. In this paper, we prove ill-posedness with the initial data satisfying
$(p>6)$ framework. In this paper, we prove ill-posedness with the initial data satisfying 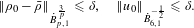 $$\begin{eqnarray}\displaystyle \Vert \unicode[STIX]{x1D70C}_{0}-\bar{\unicode[STIX]{x1D70C}}\Vert _{{\dot{B}}_{p,1}^{\frac{3}{p}}}\leqslant \unicode[STIX]{x1D6FF},\quad \Vert u_{0}\Vert _{{\dot{B}}_{6,1}^{-\frac{1}{2}}}\leqslant \unicode[STIX]{x1D6FF}. & & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle \Vert \unicode[STIX]{x1D70C}_{0}-\bar{\unicode[STIX]{x1D70C}}\Vert _{{\dot{B}}_{p,1}^{\frac{3}{p}}}\leqslant \unicode[STIX]{x1D6FF},\quad \Vert u_{0}\Vert _{{\dot{B}}_{6,1}^{-\frac{1}{2}}}\leqslant \unicode[STIX]{x1D6FF}. & & \displaystyle \nonumber\end{eqnarray}$$ $L(a)\unicode[STIX]{x1D6E5}u$ instead of
$L(a)\unicode[STIX]{x1D6E5}u$ instead of 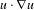 $u\cdot \unicode[STIX]{x1D6FB}u$ and construct a new decomposition of the density.
$u\cdot \unicode[STIX]{x1D6FB}u$ and construct a new decomposition of the density.
The original version of this article was submitted without an identified corresponding author. A notice detailing this has been published and the error rectified in the online PDF and HTML copies.
Author for correspondence.
Please note an has been issued for this article.