Hostname: page-component-848d4c4894-x24gv Total loading time: 0 Render date: 2024-05-28T02:53:52.928Z Has data issue: false hasContentIssue false
  • English
  • Français

Sandwiched SDEs with unbounded drift driven by Hölder noises

Part of: Stochastic processes Mathematical finance Stochastic analysis

Published online by Cambridge University Press:  08 March 2023

Giulia Di Nunno ,
Yuliya Mishura  and
Anton Yurchenko-Tytarenko
Giulia Di Nunno*
Affiliation:
University of Oslo and NHH Norwegian School of Economics
Yuliya Mishura*
Affiliation:
Taras Shevchenko National University of Kyiv
Anton Yurchenko-Tytarenko*
Affiliation:
University of Oslo
*
*Postal address: Department of Mathematics, University of Oslo, Moltke Moes vei 35, 0851 Oslo, Norway. Email address: giulian@math.uio.no
**Postal address: Department of Probability, Statistics and Actuarial Mathematics, Taras Shevchenko National University of Kyiv, Volodymyrska St. 64/13, Kyiv 01601, Ukraine. Email address: yuliyamishura@knu.ua
***Postal address:Department of Mathematics, University of Oslo, Moltke Moes vei 35, 0851 Oslo, Norway. Email address: antony@math.uio.no
Get access Rights & Permissions [Opens in a new window]

Abstract

We study a stochastic differential equation with an unbounded drift and general Hölder continuous noise of order 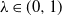$\lambda \in (0,1)$. The corresponding equation turns out to have a unique solution that, depending on a particular shape of the drift, either stays above some continuous function or has continuous upper and lower bounds. Under some mild assumptions on the noise, we prove that the solution has moments of all orders. In addition, we provide its connection to the solution of some Skorokhod reflection problem. As an illustration of our results and motivation for applications, we also suggest two stochastic volatility models which we regard as generalizations of the CIR and CEV processes. We complete the study by providing a numerical scheme for the solution.

Keywords

Sandwiched processunbounded driftHölder continuous noisenumerical schemestochastic volatility

MSC classification

Primary: 60H10: Stochastic ordinary differential equations 60H35: Computational methods for stochastic equations
Secondary: 60G22: Fractional processes, including fractional Brownian motion 91G30: Interest rates (stochastic models)
Type
Original Article
Information
Advances in Applied Probability , Volume 55 , Issue 3 , September 2023 , pp. 927 - 964
Check for updates
Copyright
© The Author(s), 2023. Published by Cambridge University Press on behalf of Applied Probability Trust

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Alfi, V., Coccetti, F., Petri, A. and Pietronero, L. (2007). Roughness and finite size effect in the NYSE stock-price fluctuations. Europ. Phys. J. B 55, 135–142.CrossRefGoogle Scholar
Andersen, L. B. G. and Piterbarg, V. V. (2006). Moment explosions in stochastic volatility models. Finance Stoch. 11, 29–50.CrossRefGoogle Scholar
Anh, V. and Inoue, A. (2005). Financial markets with memory I: dynamic models. Stoch. Anal. Appl. 23, 275–300.CrossRefGoogle Scholar
Ayache, A. and Peng, Q. (2012). Stochastic volatility and multifractional Brownian motion. In Stochastic Differential Equations and Processes, Springer, Berlin, Heidelberg, pp. 211–237.CrossRefGoogle Scholar
Azmoodeh, E., Sottinen, T., Viitasaari, L. and Yazigi, A. (2014). Necessary and sufficient conditions for Hölder continuity of Gaussian processes. Statist. Prob. Lett. 94, 230–235.CrossRefGoogle Scholar
Beran, J. (1994). Statistics for Long-Memory Processes. Chapman and Hall/CRC, Philadelphia, PA.Google Scholar
Boguslavskaya, E., Mishura, Y. and Shevchenko, G. (2018). Replication of Wiener-transformable stochastic processes with application to financial markets with memory. In Stochastic Processes and Applications, eds Silvestrov, S., Malyarenko, A. and Rančić, M., Springer, Cham, pp. 335–361.CrossRefGoogle Scholar
Bollerslev, T. and Mikkelsen, H. O. (1996). Modeling and pricing long memory in stock market volatility. J. Econometrics 73, 151–184.CrossRefGoogle Scholar
Chronopoulou, A. and Viens, F. G. (2010). Estimation and pricing under long-memory stochastic volatility. Ann. Finance 8, 379–403.CrossRefGoogle Scholar
Comte, F., Coutin, L. and Renault, E. (2010). Affine fractional stochastic volatility models. Ann. Finance 8, 337–378.CrossRefGoogle Scholar
Cox, J. C. (1996). The constant elasticity of variance option pricing model. J. Portfolio Manag. 23, 15–17.CrossRefGoogle Scholar
Cox, J. C., Ingersoll, J. E. and Ross, S. A. (1981). A re-examination of traditional hypotheses about the term structure of interest rates. J. Finance 36, 769–799.CrossRefGoogle Scholar
Cox, J. C., Ingersoll, J. E. and Ross, S. A. (1985). An intertemporal general equilibrium model of asset prices. Econometrica 53, 363–384.CrossRefGoogle Scholar
Cox, J. C., Ingersoll, J. E. and Ross, S. A. (1985). A theory of the term structure of interest rates. Econometrica 53, 385–407.CrossRefGoogle Scholar
Ding, Z., Granger, C. W. and Engle, R. F. (1993). A long memory property of stock market returns and a new model. J. Empirical Finance 1, 83–106.CrossRefGoogle Scholar
Domingo, D., d’Onofrio, A. and Flandoli, F. (2019). Properties of bounded stochastic processes employed in biophysics. Stoch. Anal. Appl. 38, 277–306.CrossRefGoogle Scholar
D’Onofrio, A. (ed.) (2013). Bounded Noises in Physics, Biology, and Engineering. Springer, New York.CrossRefGoogle Scholar
Friz, P. K. and Hairer, M. (2014). A Course on Rough Paths. Springer, Cham.CrossRefGoogle Scholar
Friz, P. K. and Victoir, N. B. (2010). Multidimensional Stochastic Processes as Rough Paths: Theory and Applications. Cambridge University Press.CrossRefGoogle Scholar
Garsia, A., Rodemich, E. and Rumsey, H. (1970). A real variable lemma and the continuity of paths of some Gaussian processes. Indiana Univ. Math. J. 20, 565–578.CrossRefGoogle Scholar
Gatheral, J., Jaisson, T. and Rosenbaum, M. (2018). Volatility is rough. Quant. Finance 18, 933–949.CrossRefGoogle Scholar
Hong, J., Huang, C., Kamrani, M. and Wang, X. (2020). Optimal strong convergence rate of a backward Euler type scheme for the Cox–Ingersoll–Ross model driven by fractional Brownian motion. Stoch. Process. Appl. 130, 2675–2692.CrossRefGoogle Scholar
Hu, Y., Nualart, D. and Song, X. (2008). A singular stochastic differential equation driven by fractional Brownian motion. Statist. Prob. Lett. 78, 2075–2085.CrossRefGoogle Scholar
Kroese, D. P. and Botev, Z. I. (2015). Spatial process simulation. In Stochastic Geometry, Spatial Statistics and Random Fields, Springer, Cham, pp. 369–404.CrossRefGoogle Scholar
Merino, R. et al. (2021). Decomposition formula for rough Volterra stochastic volatility models. Internat. J. Theoret. Appl. Finance 24, article no. 2150008.CrossRefGoogle Scholar
Mishura, Y. and Yurchenko-Tytarenko, A. (2018). Fractional Cox–Ingersoll–Ross process with non-zero ‘mean’. Modern Stoch. Theory Appl. 5, 99–111.CrossRefGoogle Scholar
Mishura, Y. and Yurchenko-Tytarenko, A. (2018). Fractional Cox–Ingersoll–Ross process with small Hurst indices. Modern Stoch. Theory Appl. 6, 13–39.Google Scholar
Mishura, Y. and Yurchenko-Tytarenko, A. (2020). Approximating expected value of an option with non-Lipschitz payoff in fractional Heston-type model. Internat. J. Theoret. Appl. Finance 23, article no. 2050031.CrossRefGoogle Scholar
Mishura, Y. and Yurchenko-Tytarenko, A. (2022). Standard and fractional reflected Ornstein–Uhlenbeck processes as the limits of square roots of Cox–Ingersoll–Ross processes. Stochastics.Google Scholar
Nourdin, I. (2012). Selected Aspects of Fractional Brownian Motion. Springer, Milan.CrossRefGoogle Scholar
Nualart, D. and Rascanu, A. (2002). Differential equations driven by fractional Brownian motion. Collectanea Math. 53, 55–81.Google Scholar
Samorodnitsky, G. (2016). Stochastic Processes and Long Range Dependence. Springer, Basel.CrossRefGoogle Scholar
Skorokhod, A. V. (1961). Stochastic equations for diffusion processes in a bounded region. Theory Prob. Appl. 6, 264–274.CrossRefGoogle Scholar
Skorokhod, A. V. (1962). Stochastic equations for diffusion processes in a bounded region. II. Theory Prob. Appl. 7, 3–23.CrossRefGoogle Scholar
Tarasov, V. (2019). On history of mathematical economics: application of fractional calculus. Mathematics 7, article no. 509.CrossRefGoogle Scholar
Yamasaki, K. et al. (2005). Scaling and memory in volatility return intervals in financial markets. Proc. Nat. Acad. Sci. USA 102, 9424–9428.CrossRefGoogle Scholar
Zähle, M. (1998). Integration with respect to fractal functions and stochastic calculus. I. Prob. Theory Relat. Fields 111, 333–374.CrossRefGoogle Scholar
Zhang, S.-Q. and Yuan, C. (2020). Stochastic differential equations driven by fractional Brownian motion with locally Lipschitz drift and their implicit Euler approximation. Proc. R. Soc. Edinburgh A 151, 1278–1304.CrossRefGoogle Scholar