Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Compaan, Erin
Lucà, Renato
and
Staffilani, Gigliola
2020.
Pointwise Convergence of the Schrödinger Flow.
International Mathematics Research Notices,
Vol. 2021,
Issue. 1,
p.
596.
Wang, Junfang
Yan, Wei
and
Yan, Xiangqian
2021.
Probabilistic pointwise convergence problem of Schrödinger equations on manifolds.
Proceedings of the American Mathematical Society,
Vol. 149,
Issue. 8,
p.
3367.
Yan, Xiangqian
Zhao, Yajuan
and
Yan, Wei
2022.
Convergence problem of Schrödinger equation in Fourier-Lebesgue spaces with rough data and random data.
Proceedings of the American Mathematical Society,
Chen, Xianghong
Duong, Xuan Thinh
Lee, Sanghyuk
and
Yan, Lixin
2022.
A sharp regularity estimate for the Schrödinger propagator on the sphere.
Journal de Mathématiques Pures et Appliquées,
Vol. 163,
Issue. ,
p.
433.
Eceizabarrena, Daniel
and
Ponce-Vanegas, Felipe
2022.
Counterexamples for the fractal Schrödinger convergence problem with an Intermediate Space Trick.
Communications on Pure and Applied Analysis,
Vol. 21,
Issue. 11,
p.
3777.
Eceizabarrena, Daniel
and
Lucà, Renato
2022.
Convergence over fractals for the periodic
Schrödinger equation.
Analysis & PDE,
Vol. 15,
Issue. 7,
p.
1775.
Lucà, Renato
2022.
Qualitative Properties of Dispersive PDEs.
Vol. 52,
Issue. ,
p.
61.
Eceizabarrena, Daniel
and
Ponce-Vanegas, Felipe
2022.
Pointwise convergence over fractals for dispersive equations with homogeneous symbol.
Journal of Mathematical Analysis and Applications,
Vol. 515,
Issue. 1,
p.
126385.
An, Chen
Chu, Rena
and
Pierce, Lillian B
2023.
Counterexamples for High-Degree Generalizations of the Schrödinger Maximal Operator.
International Mathematics Research Notices,
Vol. 2023,
Issue. 10,
p.
8371.
Yan, Xiangqian
Yan, Wei
Zhao, Yajuan
and
Yang, Meihua
2023.
Convergence problem of reduced Ostrovsky equation in Fourier–Lebesgue spaces with rough data and random data.
Stochastics and Dynamics,
Vol. 23,
Issue. 01,
Zhang, Yating
Yan, Wei
Yan, Xiangqian
and
Zhao, Yajuan
2023.
Convergence problem of Schrödinger equation and wave equation in low regularity spaces.
Journal of Mathematical Analysis and Applications,
Vol. 522,
Issue. 1,
p.
126921.
Zhang, Qiaoqiao
Yang, Meihua
Xu, Haoyuan
and
Yan, Wei
2024.
Convergence problem of the generalized Kadomtsev–Petviashvili II equation in anisotropic Sobolev space.
Nonlinear Differential Equations and Applications NoDEA,
Vol. 31,
Issue. 4,
Wang, Weimin
Yan, Wei
and
Zhang, Yating
2025.
Pointwise convergence problem of the one‐dimensional Boussinesq‐type equation.
Mathematical Methods in the Applied Sciences,
Vol. 48,
Issue. 2,
p.
1750.
Dewan, Utsav
2025.
Regularity and pointwise convergence of solutions of the Schrödinger operator with radial initial data on Damek-Ricci spaces.
Annali di Matematica Pura ed Applicata (1923 -),
Vol. 204,
Issue. 3,
p.
1161.
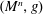 $(M^{n},g)$ be a Riemannian manifold without boundary. We study the amount of initial regularity required so that the solution to a free Schrödinger equation converges pointwise to its initial data. Assume the initial data is in
$(M^{n},g)$ be a Riemannian manifold without boundary. We study the amount of initial regularity required so that the solution to a free Schrödinger equation converges pointwise to its initial data. Assume the initial data is in 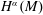 $H^{\unicode[STIX]{x1D6FC}}(M)$. For hyperbolic space, the standard sphere, and the two-dimensional torus, we prove that
$H^{\unicode[STIX]{x1D6FC}}(M)$. For hyperbolic space, the standard sphere, and the two-dimensional torus, we prove that  $\unicode[STIX]{x1D6FC}>\frac{1}{2}$ is enough. For general compact manifolds, due to the lack of a local smoothing effect, it is hard to improve on the bound
$\unicode[STIX]{x1D6FC}>\frac{1}{2}$ is enough. For general compact manifolds, due to the lack of a local smoothing effect, it is hard to improve on the bound 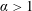 $\unicode[STIX]{x1D6FC}>1$ from interpolation. We managed to go below 1 for dimension
$\unicode[STIX]{x1D6FC}>1$ from interpolation. We managed to go below 1 for dimension 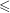 ${\leqslant}$ 3. The more interesting thing is that, for a one-dimensional compact manifold,
${\leqslant}$ 3. The more interesting thing is that, for a one-dimensional compact manifold,  $\unicode[STIX]{x1D6FC}>\frac{1}{3}$ is sufficient.
$\unicode[STIX]{x1D6FC}>\frac{1}{3}$ is sufficient.