No CrossRef data available.
Article contents
On the 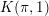 $K(\unicode[STIX]{x1D70B},1)$-problem for restrictions of complex reflection arrangements
$K(\unicode[STIX]{x1D70B},1)$-problem for restrictions of complex reflection arrangements
Published online by Cambridge University Press: 20 January 2020
Abstract
Let  $W\subset \operatorname{GL}(V)$ be a complex reflection group and
$W\subset \operatorname{GL}(V)$ be a complex reflection group and 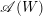 $\mathscr{A}(W)$ the set of the mirrors of the complex reflections in
$\mathscr{A}(W)$ the set of the mirrors of the complex reflections in 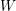 $W$. It is known that the complement
$W$. It is known that the complement 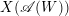 $X(\mathscr{A}(W))$ of the reflection arrangement
$X(\mathscr{A}(W))$ of the reflection arrangement 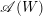 $\mathscr{A}(W)$ is a
$\mathscr{A}(W)$ is a 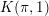 $K(\unicode[STIX]{x1D70B},1)$ space. For
$K(\unicode[STIX]{x1D70B},1)$ space. For  $Y$ an intersection of hyperplanes in
$Y$ an intersection of hyperplanes in 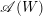 $\mathscr{A}(W)$, let
$\mathscr{A}(W)$, let 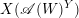 $X(\mathscr{A}(W)^{Y})$ be the complement in
$X(\mathscr{A}(W)^{Y})$ be the complement in 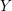 $Y$ of the hyperplanes in
$Y$ of the hyperplanes in 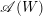 $\mathscr{A}(W)$ not containing
$\mathscr{A}(W)$ not containing 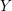 $Y$. We hope that
$Y$. We hope that 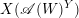 $X(\mathscr{A}(W)^{Y})$ is always a
$X(\mathscr{A}(W)^{Y})$ is always a  $K(\unicode[STIX]{x1D70B},1)$. We prove it in case of the monomial groups
$K(\unicode[STIX]{x1D70B},1)$. We prove it in case of the monomial groups 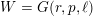 $W=G(r,p,\ell )$. Using known results, we then show that there remain only three irreducible complex reflection groups, leading to just eight such induced arrangements for which this
$W=G(r,p,\ell )$. Using known results, we then show that there remain only three irreducible complex reflection groups, leading to just eight such induced arrangements for which this 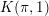 $K(\unicode[STIX]{x1D70B},1)$ property remains to be proved.
$K(\unicode[STIX]{x1D70B},1)$ property remains to be proved.
Keywords
MSC classification
Information
- Type
- Research Article
- Information
- Copyright
- © The Authors 2020

