Article contents
Ergodic properties of the Anzai skew-product for the non-commutative torus
Published online by Cambridge University Press: 14 January 2020
Abstract
We provide a systematic study of a non-commutative extension of the classical Anzai skew-product for the cartesian product of two copies of the unit circle to the non-commutative 2-tori. In particular, some relevant ergodic properties are proved for these quantum dynamical systems, extending the corresponding ones enjoyed by the classical Anzai skew-product. As an application, for a uniquely ergodic Anzai skew-product 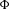 $\unicode[STIX]{x1D6F7}$ on the non-commutative
$\unicode[STIX]{x1D6F7}$ on the non-commutative  $2$ -torus
$2$ -torus 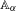 $\mathbb{A}_{\unicode[STIX]{x1D6FC}}$ ,
$\mathbb{A}_{\unicode[STIX]{x1D6FC}}$ , 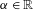 $\unicode[STIX]{x1D6FC}\in \mathbb{R}$ , we investigate the pointwise limit,
$\unicode[STIX]{x1D6FC}\in \mathbb{R}$ , we investigate the pointwise limit, 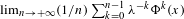 $\lim _{n\rightarrow +\infty }(1/n)\sum _{k=0}^{n-1}\unicode[STIX]{x1D706}^{-k}\unicode[STIX]{x1D6F7}^{k}(x)$ , for
$\lim _{n\rightarrow +\infty }(1/n)\sum _{k=0}^{n-1}\unicode[STIX]{x1D706}^{-k}\unicode[STIX]{x1D6F7}^{k}(x)$ , for  $x\in \mathbb{A}_{\unicode[STIX]{x1D6FC}}$ and
$x\in \mathbb{A}_{\unicode[STIX]{x1D6FC}}$ and  $\unicode[STIX]{x1D706}$ a point in the unit circle, and show that there are examples for which the limit does not exist, even in the weak topology.
$\unicode[STIX]{x1D706}$ a point in the unit circle, and show that there are examples for which the limit does not exist, even in the weak topology.
Keywords
Information
- Type
- Original Article
- Information
- Copyright
- © The Author(s) 2020. Published by Cambridge University Press
References
- 8
- Cited by


