Published online by Cambridge University Press: 14 March 2024
Existence of specific eternal solutions in exponential self-similar form to the following quasilinear diffusion equation with strong absorption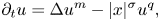
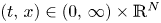 , with $m>1$
, with $m>1$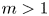 , $q\in (0,\,1)$
, $q\in (0,\,1)$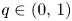 and $\sigma =\sigma _c:=2(1-q)/ (m-1)$
and $\sigma =\sigma _c:=2(1-q)/ (m-1)$ is proved. Looking for radially symmetric solutions of the form
is proved. Looking for radially symmetric solutions of the form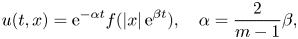
 for which there exists a one-parameter family $(u_A)_{A>0}$
for which there exists a one-parameter family $(u_A)_{A>0}$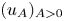 of solutions with compactly supported and non-increasing profiles $(f_A)_{A>0}$
of solutions with compactly supported and non-increasing profiles $(f_A)_{A>0}$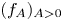 satisfying $f_A(0)=A$
satisfying $f_A(0)=A$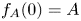 and $f_A'(0)=0$
and $f_A'(0)=0$ . An important feature of these solutions is that they are bounded and do not vanish in finite time, a phenomenon which is known to take place for all non-negative bounded solutions when $\sigma \in (0,\,\sigma _c)$
. An important feature of these solutions is that they are bounded and do not vanish in finite time, a phenomenon which is known to take place for all non-negative bounded solutions when $\sigma \in (0,\,\sigma _c)$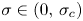 .
.