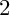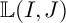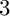1 Indecomposable rank 2 modules with tightly $3$ -interlacing layers

In [Reference Baur, Bogdanic and Elsener1], we studied the category ${\textrm {CM}}(B_{k,n})$ of Cohen–Macaulay modules over the completion of an algebra $B_{k,n}$ , which is a quotient of the preprojective algebra of type $A_{n-1}$ . The category ${\textrm {CM}}(B_{k,n})$ is important in a categorification of the cluster algebra structure on the homogeneous coordinate ring $\mathbb C[Gr(k, n)]$ of the Grassmannian variety of k-dimensional subspaces in $\mathbb C^n$ (see [Reference Geiss, Leclerc and Schröer3]–[Reference Jensen, King and Su5]).

For the notation and background results used in this note, we refer the reader to [Reference Baur, Bogdanic and Elsener1, Sect. 1]. We thank Karin Erdmann and Alastair King for useful conversations about indecomposable modules.

In [Reference Baur, Bogdanic and Elsener1, Def. 4.2], we constructed a Cohen–Macaulay module of an arbitrary rank. In the case of rank 2, in [Reference Baur, Bogdanic and Elsener1, Lem. 5.12], we claimed that the constructed module is indecomposble. In fact, the rank 2 module from this lemma is not indecomposable. The aim of this note is to correct this mistake, that is, for given k-subsets I and J that are tightly $3$ -interlacing, to construct explicitly an indecomposable rank 2 Cohen–Macaulay module with filtration $L_I\mid L_J$ .

We show that this module is indecomposable by proving that its endomorphism ring does not have nontrivial idempotents.

Assume that we are in the case when I and J are tightly 3-interlacing k-subsets ( $|I\setminus J|=|J\setminus I|=3$ and noncommon elements of I and J interlace). Write $I\setminus J$ as $\{i_1,i_2,i_3\}$ and $J\setminus I=\{j_1,j_2,j_3\}$ so that $1\le i_1<j_1<i_2<j_2<i_3<j_3\le n$ .

The following construction covers all indecomposable rank 2 modules in case when the category ${\textrm {CM}}(B_{k,n})$ is tame and $(k,n)=(3,9)$ .

We want to define a rank 2 module $\operatorname {\mathbb {L}}\nolimits (I,J)$ in ${\textrm {CM}}(B_{k,n})$ in a similar way as rank 1 modules are defined in [Reference Jensen, King and Su5]. Let $V_i:=\operatorname {\mathbb {C}}\nolimits [|t|]\oplus \operatorname {\mathbb {C}}\nolimits [|t|]$ , $i=1,\dots , n$ . The module $\operatorname {\mathbb {L}}\nolimits (I,J)$ has $V_i$ at each vertex $1,2,\dots , n$ of $\Gamma _n$ , where $\Gamma _n$ is the quiver of the boundary algebra, that is, with vertices $1,2,\dots , n$ on a cycle and arrows $x_i: i-1\to i$ , $y_i:i\to i-1$ . Observe the following matrices:

$$ \begin{align*} \begin{array}{llll} A_1:=\begin{pmatrix} t & -2 \\ 0 & 1 \end{pmatrix}, & B_1:=\begin{pmatrix} t & 0 \\ 0 & 1 \end{pmatrix}, & C_1:= \begin{pmatrix} t & -1 \\ 0 & 1 \end{pmatrix}, & D_1:= \begin{pmatrix} 1 & 0 \\ 0 & 1 \end{pmatrix}, \\ A_2:=\begin{pmatrix} 1 & 2 \\ 0 & t \end{pmatrix}, & B_2:= \begin{pmatrix} 1 & 0 \\ 0 & t \end{pmatrix}, & C_2:= \begin{pmatrix} 1 & 1 \\ 0 & t \end{pmatrix}, & D_2:= \begin{pmatrix} t & 0 \\ 0 & t \end{pmatrix}. \end{array} \end{align*} $$

Note that these are all matrix factorisations of $ \begin {pmatrix} t & 0 \\ 0 & t \end {pmatrix}$ : $A_1A_2=B_1B_2=C_1C_2=D_1D_2=\begin {pmatrix} t & 0 \\ 0 & t \end {pmatrix}$ .

Definition 1.1. Let $I, J$ be tightly 3-interlacing k-subsets of $\{1,2,\dots ,n\}$ . At the vertices of $\Gamma _n$ , $\operatorname {\mathbb {L}}\nolimits (I,J)$ has the spaces $V_1,\dots , V_n$ . We define the maps $x_i,y_i$ as follows:

$$ \begin{align*} x_i:V_{i-1}\to V_i \mbox{ acts as } \left\{ \begin{array}{rl} A_1, & \mbox{if}\ i=i_1, \\ B_2, & \mbox{if}\ i=j_1, \\ B_1, & \mbox{if}\ i=i_2, \\ C_2, & \mbox{if}\ i=j_2, \\ C_1, & \mbox{if}\ i=i_3, \\ A_2, & \mbox{if}\ i=j_3, \\ D_1, & \mbox{if}\ i\in I\cap J,\\ D_2, & \mbox{if}\ i\in I^c\cap J^c. \end{array}\right. \hskip.5cm y_i:V_{i}\to V_{i-1} \mbox{ acts as } \left\{ \begin{array}{rl} A_2, & \mbox{if}\ i=i_1, \\ B_1, & \mbox{if}\ i=j_1, \\ B_2, & \mbox{if}\ i=i_2, \\ C_1, & \mbox{if}\ i=j_2, \\ C_2, & \mbox{if}\ i=i_3, \\ A_1, & \mbox{if}\ i=j_3, \\ D_2, & \mbox{if}\ i\in I\cap J,\\ D_1, & \mbox{if}\ i\in I^c\cap J^c. \end{array}\right. \end{align*} $$

One easily checks that $xy=yx$ and $x^k=y^{n-k}$ at all vertices and that $\operatorname {\mathbb {L}}\nolimits (I,J)$ is free over the center of the boundary algebra. Hence, the following proposition holds.

For the remainder of the paper, if $w=tv$ , then $t^{-1}w$ stands for v.

Proposition 1.3. Let I and J be tightly 3-interlacing, $n\ge 6$ arbitrary, $I\setminus J=\{i_1,i_2,i_3\}$ and $J\setminus I=\{j_1,j_2,j_3\}$ where $1\le i_1<j_1<i_2<j_2<i_3<j_3\le n$ . If $\varphi =( \varphi _i)_{i=1}^n\in $ Hom $(\operatorname {\mathbb {L}}\nolimits (I,J),\operatorname {\mathbb {L}}\nolimits (I,J))$ , then

$$ \begin{align*} \varphi_{i_1}=\varphi_{i_2}=\varphi_{i_3}&=\begin{pmatrix}a & bt \\ c & d \end{pmatrix},\\ \varphi_{j_1}&=\begin{pmatrix} a & b \\ ct & d \end{pmatrix},\\ \varphi_{j_2}&=\begin{pmatrix} a+c & b+t^{-1}(d-a-c) \\ ct & d-c \end{pmatrix},\\ \varphi_{j_3}&=\begin{pmatrix} a+2c & b+2t^{-1}(d-a-2c) \\ ct & d-2c \end{pmatrix},\\ \varphi_{i}&=\varphi_{i-1}, \,\, \text{for } i\in (I^c \cap J^c)\cup (I\cap J), \end{align*} $$

with $a,b,c,d\in \operatorname {\mathbb {C}}\nolimits [|t|]$ . Furthermore, $t\mid c$ and $t\mid (a-d)$ .

Proof. First we prove the statement for $n=6$ , $I=\{1,3,5\}$ , $J=\{2,4,6\}$ , and $\varphi \in $ End $(\operatorname {\mathbb {L}}\nolimits (I,J))$ . Then $\varphi =\left ( \varphi _1, \varphi _2, \ldots , \varphi _6 \right ),$ where each $\varphi _i$ is an element of $M_2(\operatorname {\mathbb {C}}\nolimits [[t]])$ (matrices over the center).

We check the relations which arise when we go from a peak of the rim of $\operatorname {\mathbb {L}}\nolimits (I,J)$ to a valley of the rim:

$$ \begin{align*} \begin{array}{clccl} (i) & x_2\varphi_1 = \varphi_2x_2, & \quad & (ii) & x_3\varphi_2 = \varphi_3x_3, \\ (iii) & x_4\varphi_3 = \varphi_4x_4, & & (iv) & x_5\varphi_4 = \varphi_5x_5, \\ (v) & x_6\varphi_5 = \varphi_6x_6, & & (vi) & x_1\varphi_6 = \varphi_1 x_1. \end{array} \end{align*} $$

Let $\varphi _1=\begin {pmatrix} a & b \\ c & d \end {pmatrix}$ . The equalities $\varphi _1=\varphi _3=\varphi _5$ follow immediately from $t\varphi _3=\varphi _3B_1B_2=B_1B_2\varphi _1=t\varphi _1$ and $t\varphi _5=\varphi _5C_1C_2=C_1C_2\varphi _3=t\varphi _3$ .

If we consider matrices $x_i$ and $y_i$ as elements of the ring $M_2(\operatorname {\mathbb {C}}\nolimits ((t)))$ , where all of them are units, then from $ x_2\varphi _1 = \varphi _2x_2$ follows that

$$ \begin{align*}\varphi_2=x_2\varphi_1x_2^{-1}=\begin{pmatrix} 1 & 0 \\ 0 & t \end{pmatrix} \begin{pmatrix} a & b \\ c & d \end{pmatrix} \begin{pmatrix} 1 & 0 \\ 0 & t^{-1} \end{pmatrix} = \begin{pmatrix} a & t^{-1}b \\ ct & d \end{pmatrix}.\end{align*} $$

Thus, $t\mid b$ , so if we replace b by $bt$ , this yields

$$ \begin{align*}\varphi_1=\begin{pmatrix} a & bt \\ c & d\end{pmatrix}, \quad \varphi_2=\begin{pmatrix} a & b \\ ct & d\end{pmatrix}.\end{align*} $$

Similarly, from $x_4\varphi _3 = \varphi _4x_4$ , we have

$$ \begin{align*}\varphi_4=x_4\varphi_3x_4^{-1}= \begin{pmatrix} 1 & 1 \\ 0 & t \end{pmatrix} \begin{pmatrix} a & bt \\ c & d\end{pmatrix} \begin{pmatrix} 1 & -t^{-1} \\ 0 & t^{-1} \end{pmatrix} = \begin{pmatrix} a+c & b+t^{-1}(d-a-c) \\ ct & d-c \end{pmatrix} ,\end{align*} $$

and from $x_6\varphi _5 = \varphi _6x_6$ , we have

$$ \begin{align*}\varphi_6=x_6\varphi_5x_6^{-1}=\begin{pmatrix} 1 & 2 \\ 0 & t \end{pmatrix} \begin{pmatrix} a & bt \\ c & d\end{pmatrix} \begin{pmatrix} 1 & -2t^{-1} \\ 0 & t^{-1} \end{pmatrix} =\begin{pmatrix} a+2c & b+2t^{-1}(d-a-2c) \\ ct & d-2c \end{pmatrix}.\end{align*} $$

The statement about the divisibility follows since we have the two properties $t\mid (d-c-a)$ and $t\mid (d-a-2c)$ . Combined, they imply $t\mid c$ and $t\mid d-a$ as claimed.

In the general case, the proof is almost the same as in the case $n=6$ . The only thing left to note is that if $i\in (I^c \cap J^c)\cup (I\cap J)$ , then $x_i$ is a scalar matrix (either identity or t times identity), so the equality $x_{i}\varphi _{i-1}=\varphi _{i}x_{i}$ yields $\varphi _{i-1}=\varphi _{i}$ .

Proof. We first consider $n=6$ . In this case, we can assume $I=\{1,3,5\}$ and $J=\{2,4,6\}$ . Take $\varphi =(\varphi _i)_i\in $ End $(\operatorname {\mathbb {L}}\nolimits (I,J))$ as in the previous proposition.

To show the indecomposability, we assume that $\varphi $ is an idempotent endomorphism of $\operatorname {\mathbb {L}}\nolimits (I,J)$ and show that $\varphi $ is trivial (the identity or the zero endomorphism).

Assume that $\varphi _2^2=\varphi _2$ , that is

$$ \begin{align*} \varphi_2^2=\begin{pmatrix} a^2+bct & (a+d)b \\ (a+d)ct & d^2+bct\end{pmatrix}= \begin{pmatrix}a & b \\ ct & d \end{pmatrix}. \end{align*} $$

The equations $a^2+bct=a \quad \mbox {and} \quad d^2+bct=d$ on the diagonal entries give $a-a^2=d-d^2$ , that is, $a-d=a^2-d^2=(a-d)(a+d)$ and hence $a=d$ or $a+d=1$ . The equations also show that $t\mid a(1-a)$ and that $t\mid d(1-d)$ .

Assume first $a=d$ . If $b\ne 0$ , we get $a=\frac {1}{2}$ , which contradicts to $t\mid a-a^2$ . Analogously for $c\ne 0$ . Thus $b=c=0$ and $a=d=0$ or $a=d=1$ , the two trivial cases (note that if $\varphi _2$ is trivial, then $x_{i}\varphi _{i-1}=\varphi _{i}x_{i}$ yields $\varphi _2=\varphi _i$ , for all i).

So assume that $a\ne d$ and $d=1-a$ . Combining $t\mid a(1-a)$ with the fact that t divides $a-d=2a-1$ implies that $t\mid 1$ , which is a contradiction.

For a general n, since $\varphi _i=\varphi _{i+1}$ for $i+1\in (I^c\cap J^c)\cup (I\cap J)$ , the proof follows as for $n=6$ .

The question of uniqueness of such a rank 2 indecomposable module is studied in [Reference Baur, Bogdanic and Li2]. For given tightly $3$ -interlacing I and J, there is a unique indecomposable rank 2 module with filtration $L_I\mid L_J$ . This statement is clear in case when the category ${\textrm {CM}}(B_{k,n})$ is of finite representation type and in case when ${\textrm {CM}}(B_{k,n})$ is tame, with $(k,n)\in \{(3,9),\, (4,8)\}$ . Consequently, we have the following theorem.