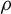Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Corte, Alessandro Della
2023.
Generic $$\delta $$-chaos for erasing interval maps.
Bollettino dell'Unione Matematica Italiana,
Vol. 16,
Issue. 2,
p.
163.