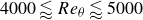Most read
This page lists the top ten most read articles for this journal based on the number of full text views and downloads recorded on Cambridge Core over the last 90 days. This list is updated on a daily basis.
Contents
Breaking wave impacts on an elastic plate
-
- Published online by Cambridge University Press:
- 24 July 2025, A54
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Interactions enhance dispersion in fluctuating channels via emergent flows
-
- Published online by Cambridge University Press:
- 27 September 2023, A8
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
The onset of outer-layer self-similarity in turbulent boundary layers
-
- Published online by Cambridge University Press:
- 21 July 2025, A37
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Wave-riding and wave-passing by ducklings in formation swimming
-
- Published online by Cambridge University Press:
- 05 October 2021, R2
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Transport phenomena in microswimmer suspensions: migration, collective motion, diffusion and rheology
-
- Published online by Cambridge University Press:
- 08 August 2025, P1
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Beyond Nusselt number: assessing Reynolds and length scalings in rotating convection under stress-free boundary conditions
-
- Published online by Cambridge University Press:
- 25 July 2025, A3
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Variable-property and intrinsic compressibility corrections for turbulence models using near-wall scaling theories
-
- Published online by Cambridge University Press:
- 15 September 2025, A8
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Turbulence–chemistry interaction in a non-equilibrium hypersonic boundary layer
-
- Published online by Cambridge University Press:
- 20 August 2025, A30
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Reduced-order variational mode decomposition to reveal transient and non-stationary dynamics in fluid flows
-
- Published online by Cambridge University Press:
- 28 June 2023, A7
-
- Article
-
- You have access
- HTML
- Export citation
On the maximum spreading of viscous droplets impacting flat solid surfaces
-
- Published online by Cambridge University Press:
- 05 September 2025, A42
-
- Article
-
- You have access
- Open access
- HTML
- Export citation