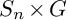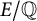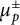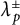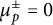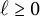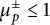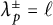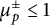Refine listing
Actions for selected content:
350 results in 11Rxx
THE FERMAT QUARTIC X4 + Y4 = 2m IN QUADRATIC NUMBER FIELDS
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society , First View
- Published online by Cambridge University Press:
- 15 August 2025, pp. 1-10
-
- Article
- Export citation
Counting cyclic extensions with local conditions and applications to L-values in the critical strip
- Part of
-
- Journal:
- Canadian Journal of Mathematics , First View
- Published online by Cambridge University Press:
- 08 August 2025, pp. 1-39
-
- Article
- Export citation
Ergodicity and algebraicity of the fast and slow triangle maps
- Part of
-
- Journal:
- Ergodic Theory and Dynamical Systems , First View
- Published online by Cambridge University Press:
- 08 August 2025, pp. 1-35
-
- Article
- Export citation
RIGID INNER FORMS OVER GLOBAL FUNCTION FIELDS
- Part of
-
- Journal:
- Journal of the Institute of Mathematics of Jussieu , First View
- Published online by Cambridge University Press:
- 21 July 2025, pp. 1-76
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
On the Fitting ideals of anticyclotomic Selmer groups of elliptic curves with good ordinary reduction
- Part of
-
- Journal:
- Canadian Mathematical Bulletin , First View
- Published online by Cambridge University Press:
- 14 July 2025, pp. 1-11
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Elliptic curves and spin
- Part of
-
- Journal:
- Mathematical Proceedings of the Cambridge Philosophical Society , First View
- Published online by Cambridge University Press:
- 11 July 2025, pp. 1-21
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
On the
 $\mathfrak{M}_H(G)$-property
$\mathfrak{M}_H(G)$-property
- Part of
-
- Journal:
- Mathematical Proceedings of the Cambridge Philosophical Society / Volume 179 / Issue 2 / September 2025
- Published online by Cambridge University Press:
- 24 June 2025, pp. 449-501
- Print publication:
- September 2025
-
- Article
- Export citation
Class groups and Selmer groups in special families
- Part of
-
- Journal:
- Canadian Mathematical Bulletin , First View
- Published online by Cambridge University Press:
- 10 June 2025, pp. 1-23
-
- Article
- Export citation
Congruent elliptic curves and the
 $\tau $-component in the Iwasawa main conjecture
$\tau $-component in the Iwasawa main conjecture
- Part of
-
- Journal:
- Mathematical Proceedings of the Cambridge Philosophical Society / Volume 179 / Issue 2 / September 2025
- Published online by Cambridge University Press:
- 29 May 2025, pp. 373-408
- Print publication:
- September 2025
-
- Article
- Export citation
The p-adic limits of class numbers in
 ${\mathbb{Z}}_p$-towers
${\mathbb{Z}}_p$-towers
- Part of
-
- Journal:
- Mathematical Proceedings of the Cambridge Philosophical Society / Volume 179 / Issue 2 / September 2025
- Published online by Cambridge University Press:
- 23 May 2025, pp. 299-329
- Print publication:
- September 2025
-
- Article
- Export citation
Annihilating class groups in p-elementary abelian extensions
- Part of
-
- Journal:
- Mathematical Proceedings of the Cambridge Philosophical Society / Volume 179 / Issue 1 / July 2025
- Published online by Cambridge University Press:
- 19 May 2025, pp. 145-166
- Print publication:
- July 2025
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Squarefree values of polynomial discriminants II
- Part of
-
- Journal:
- Forum of Mathematics, Pi / Volume 13 / 2025
- Published online by Cambridge University Press:
- 13 May 2025, e17
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
MONOGENITY OF THE COMPOSITION OF POLYNOMIALS
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society , First View
- Published online by Cambridge University Press:
- 08 May 2025, pp. 1-9
-
- Article
- Export citation
A note on the values of Mahler measure in quadratic fields
- Part of
-
- Journal:
- Canadian Mathematical Bulletin , First View
- Published online by Cambridge University Press:
- 21 April 2025, pp. 1-11
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Canonical integral models for Shimura varieties of abelian type
- Part of
-
- Journal:
- Forum of Mathematics, Sigma / Volume 13 / 2025
- Published online by Cambridge University Press:
- 07 April 2025, e69
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Bounds on the Pythagoras number and indecomposables in biquadratic fields
- Part of
-
- Journal:
- Proceedings of the Edinburgh Mathematical Society / Volume 68 / Issue 3 / August 2025
- Published online by Cambridge University Press:
- 31 March 2025, pp. 843-868
-
- Article
- Export citation
On the
 $\mu$-invariant of two-variable
$\mu$-invariant of two-variable  $2$-adic
$2$-adic  $\boldsymbol{L}$-functions
$\boldsymbol{L}$-functions
- Part of
-
- Journal:
- Glasgow Mathematical Journal / Volume 67 / Issue 3 / September 2025
- Published online by Cambridge University Press:
- 24 February 2025, pp. 325-355
- Print publication:
- September 2025
-
- Article
- Export citation
ON MALLE’S CONJECTURE FOR THE PRODUCT OF SYMMETRIC AND NILPOTENT GROUPS
- Part of
-
- Journal:
- Nagoya Mathematical Journal , First View
- Published online by Cambridge University Press:
- 17 February 2025, pp. 1-26
-
- Article
- Export citation
A bound on the
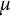 $\mu $-invariants of supersingular elliptic curves
$\mu $-invariants of supersingular elliptic curves
- Part of
-
- Journal:
- Canadian Mathematical Bulletin , First View
- Published online by Cambridge University Press:
- 07 February 2025, pp. 1-10
-
- Article
- Export citation
ON PSEUDO-NULLITY OF THE FINE MORDELL–WEIL GROUP
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society / Volume 112 / Issue 1 / August 2025
- Published online by Cambridge University Press:
- 03 February 2025, pp. 71-79
- Print publication:
- August 2025
-
- Article
- Export citation