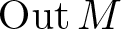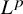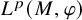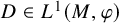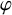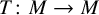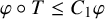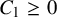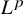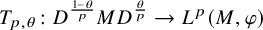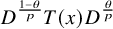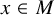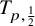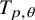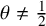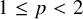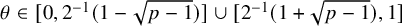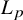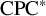Refine listing
Actions for selected content:
415 results in 46Lxx
A central limit theorem for partial transposes of multipartite Wishart matrices
- Part of
-
- Journal:
- Proceedings of the Royal Society of Edinburgh. Section A: Mathematics , First View
- Published online by Cambridge University Press:
- 08 September 2025, pp. 1-38
-
- Article
-
- You have access
- HTML
- Export citation
PROPERTY (T) AND STRONG 1-BOUNDEDNESS FOR VON NEUMANN ALGEBRAS
- Part of
-
- Journal:
- Journal of the Institute of Mathematics of Jussieu , First View
- Published online by Cambridge University Press:
- 01 September 2025, pp. 1-34
-
- Article
- Export citation
ON TOEPLITZ ALGEBRAS OF PRODUCT SYSTEMS
- Part of
-
- Journal:
- Journal of the Australian Mathematical Society , First View
- Published online by Cambridge University Press:
- 26 August 2025, pp. 1-28
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Operator realizations of non-commutative analytic functions
- Part of
-
- Journal:
- Forum of Mathematics, Sigma / Volume 13 / 2025
- Published online by Cambridge University Press:
- 22 August 2025, e137
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Quasi-invariant lifts of completely positive maps for groupoid actions
- Part of
-
- Journal:
- Mathematical Proceedings of the Cambridge Philosophical Society , First View
- Published online by Cambridge University Press:
- 19 August 2025, pp. 1-29
-
- Article
- Export citation
Semigroups of self-similar actions and higher rank Baumslag–Solitar semigroups
- Part of
-
- Journal:
- Proceedings of the Royal Society of Edinburgh. Section A: Mathematics , First View
- Published online by Cambridge University Press:
- 18 August 2025, pp. 1-30
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Local topological order and boundary algebras
- Part of
-
- Journal:
- Forum of Mathematics, Sigma / Volume 13 / 2025
- Published online by Cambridge University Press:
- 15 August 2025, e135
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
X-ROKHLIN PROPERTY FOR ACTIONS OF COUNTABLE DISCRETE GROUPS
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society , First View
- Published online by Cambridge University Press:
- 04 July 2025, pp. 1-11
-
- Article
- Export citation
QUANTUM EXPANDERS AND QUANTIFIER REDUCTION FOR TRACIAL VON NEUMANN ALGEBRAS
- Part of
-
- Journal:
- The Journal of Symbolic Logic , First View
- Published online by Cambridge University Press:
- 04 July 2025, pp. 1-37
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Elementary equivalence and disintegration of tracial von Neumann algebras
- Part of
-
- Journal:
- Forum of Mathematics, Sigma / Volume 13 / 2025
- Published online by Cambridge University Press:
- 02 July 2025, e105
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
SIMPLE TRACIALLY
 $\mathcal {Z}$-ABSORBING C*-ALGEBRAS
$\mathcal {Z}$-ABSORBING C*-ALGEBRAS
- Part of
-
- Journal:
- Journal of the Institute of Mathematics of Jussieu / Volume 24 / Issue 6 / November 2025
- Published online by Cambridge University Press:
- 04 June 2025, pp. 2135-2179
- Print publication:
- November 2025
-
- Article
- Export citation
CORONA RIGIDITY
- Part of
-
- Journal:
- Bulletin of Symbolic Logic / Volume 31 / Issue 2 / June 2025
- Published online by Cambridge University Press:
- 30 June 2025, pp. 195-287
- Print publication:
- June 2025
-
- Article
- Export citation
Cartesian closed varieties II: links to algebra and self-similarity
- Part of
-
- Journal:
- Proceedings of the Royal Society of Edinburgh. Section A: Mathematics , First View
- Published online by Cambridge University Press:
- 14 May 2025, pp. 1-45
-
- Article
- Export citation
On Fock covariance for product systems and the reduced Hao–Ng isomorphism problem by discrete actions
- Part of
-
- Journal:
- Proceedings of the Royal Society of Edinburgh. Section A: Mathematics , First View
- Published online by Cambridge University Press:
- 28 April 2025, pp. 1-53
-
- Article
- Export citation
Classification of homomorphisms from
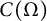 $C(\Omega)$ to a
$C(\Omega)$ to a 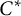 $C^*$-algebra
$C^*$-algebra
- Part of
-
- Journal:
- Canadian Journal of Mathematics , First View
- Published online by Cambridge University Press:
- 21 April 2025, pp. 1-24
-
- Article
- Export citation
Every locally compact group is the outer automorphism group of a II1 factor
- Part of
-
- Journal:
- Proceedings of the Royal Society of Edinburgh. Section A: Mathematics , First View
- Published online by Cambridge University Press:
- 28 March 2025, pp. 1-12
-
- Article
- Export citation
On the extension of positive maps to Haagerup noncommutative
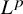 $L^p$-spaces
$L^p$-spaces
- Part of
-
- Journal:
- Forum of Mathematics, Sigma / Volume 13 / 2025
- Published online by Cambridge University Press:
- 28 March 2025, e65
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
The local geometry of idempotent Schur multipliers
- Part of
-
- Journal:
- Forum of Mathematics, Pi / Volume 13 / 2025
- Published online by Cambridge University Press:
- 24 March 2025, e14
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Nuclearity and
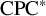 ${\mathrm {CPC}^*}$-Systems
${\mathrm {CPC}^*}$-Systems
- Part of
-
- Journal:
- Forum of Mathematics, Sigma / Volume 13 / 2025
- Published online by Cambridge University Press:
- 20 March 2025, e59
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
MULTIPLICATIVE (IN)STABILITY OF BANACH ALGEBRAS
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society / Volume 112 / Issue 1 / August 2025
- Published online by Cambridge University Press:
- 17 March 2025, pp. 194-200
- Print publication:
- August 2025
-
- Article
- Export citation











































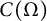
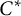




 , there exists a homomorphism
, there exists a homomorphism